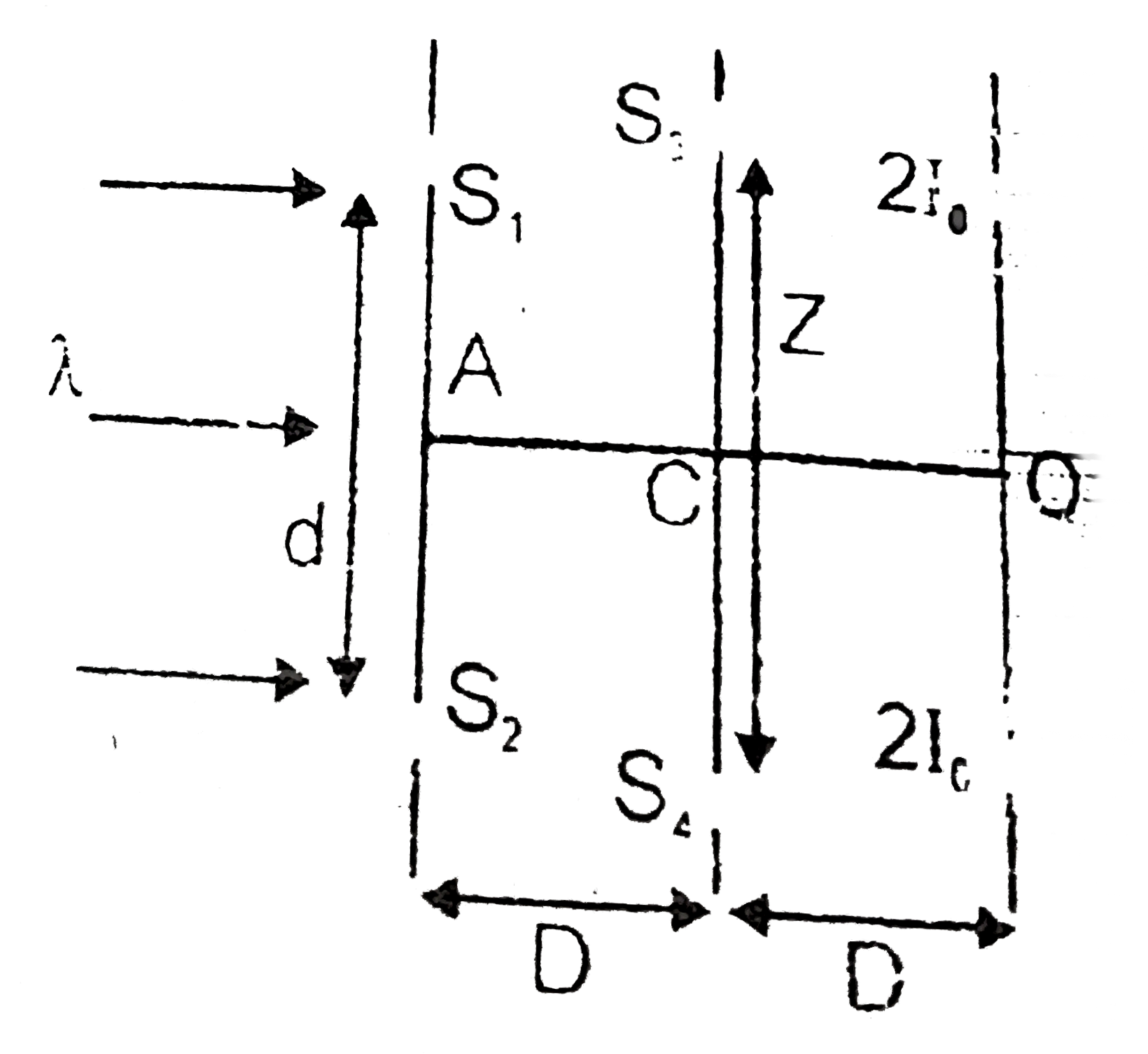
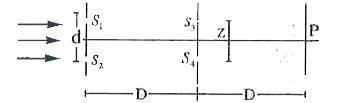
In arrangement shown in figure, plane wavefront of monochromatic light

The circuit was in the shown state for a long time. Now if the switch S is closed then the net charge that flows through the switch S, will be
ocr_image

View Solution

If E denotes of electric field, the dimension of a quantity $\in_{0} \frac{d E}{d t}$ are those of

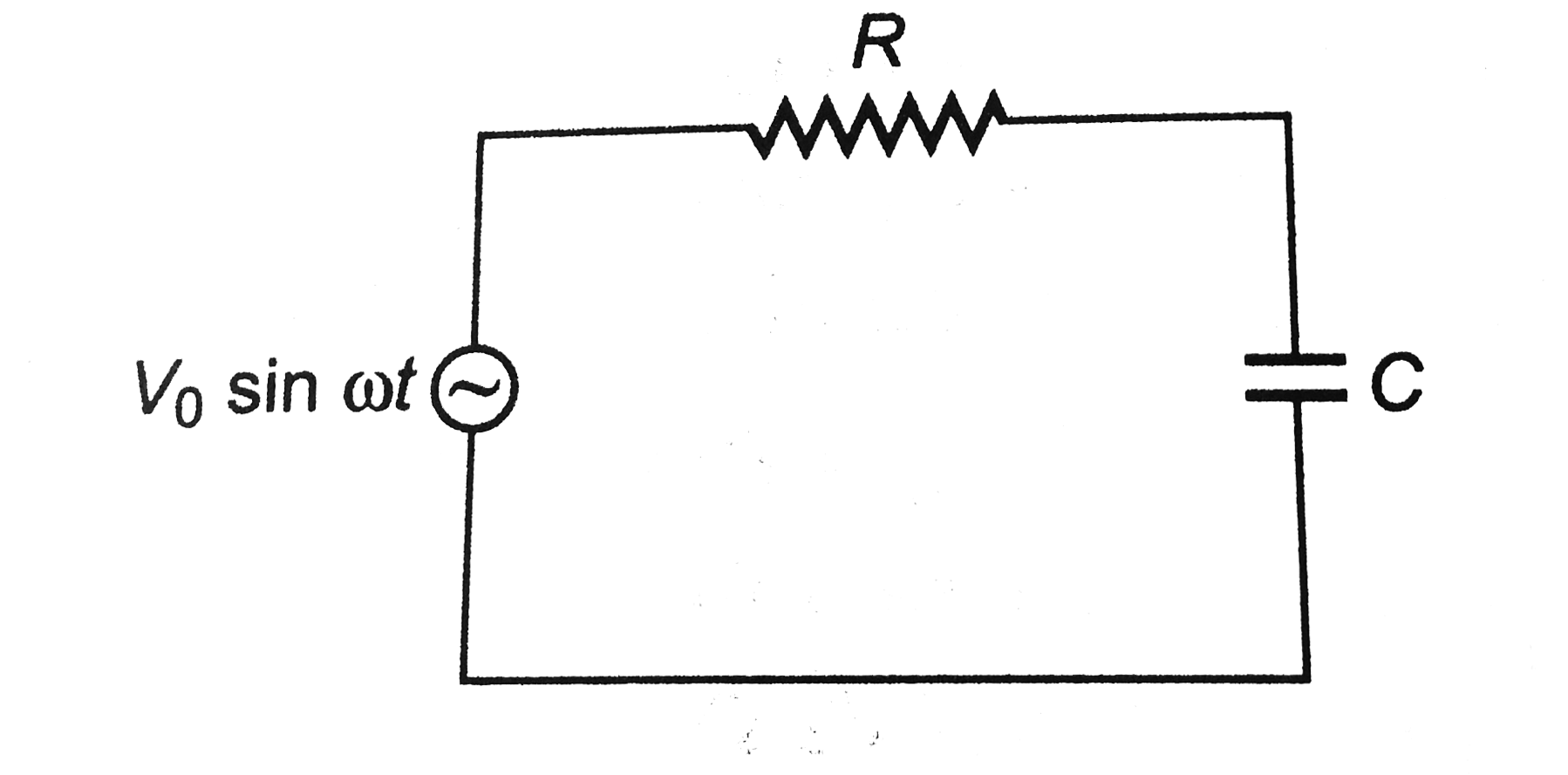
View Solution

An $A C$ voltage source $V = V_{0} sin ω t$ is connected across resistance $R$ and capacitance $C$ as shown in figure. It is given that $ω C = \frac{1}{R}$ . The peak current is $I_{0}$ . If the angular frequency of the voltage source is changed to $\frac{ω}{\sqrt{3}},$ then the new peak current in the circuit is
ocr_image .

View Solution

A galvanometer having 30 divisions has a current sensitivity of $20 μ A /$ division. It has a resistance of 5 $Ω$ How will you convert it into an ammeter measuring upto 1A ?

View Solution

An electromagnetic wave of frequency $υ = 3$ MHz passes from vacuum into a dielectric medium with permittivity $ε = 4$ . Then

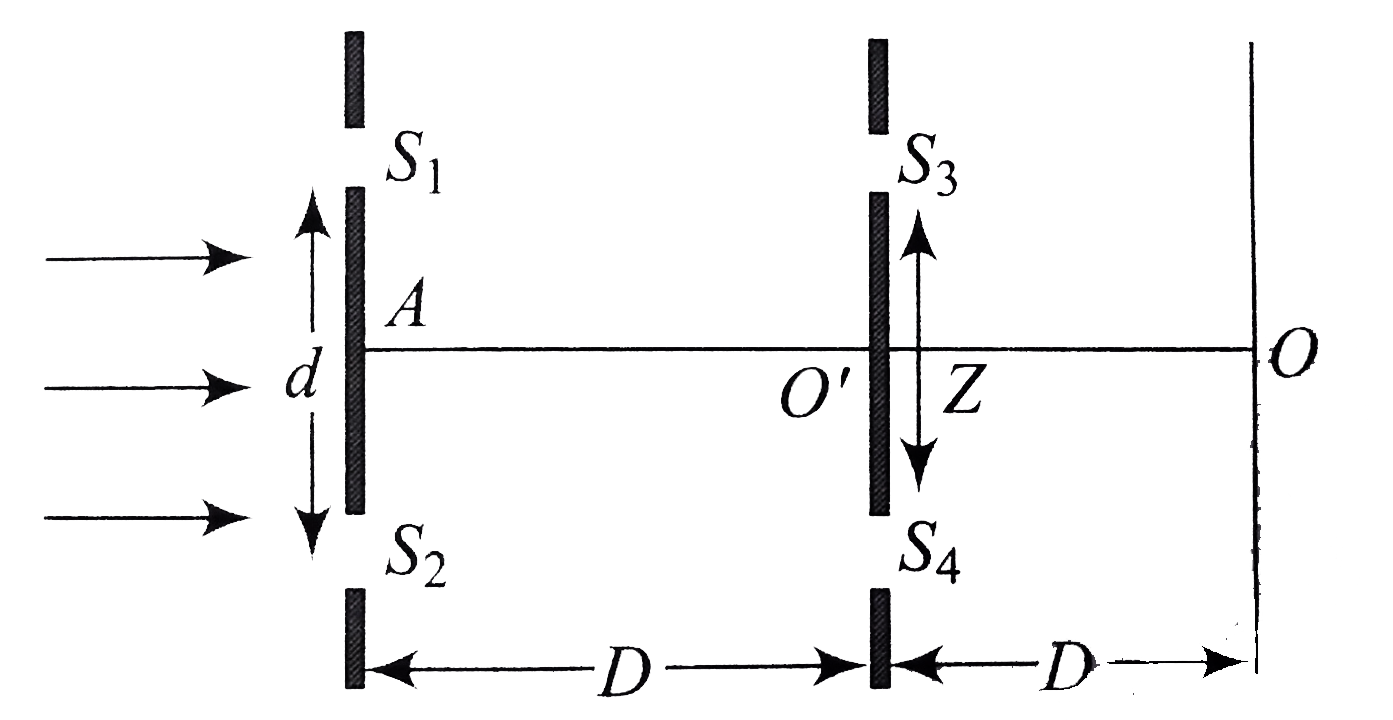
View Solution

In the arrangement shown in figure, light of wavelength 6000 Å is incident on slits S_(1) and S_(2) have been opened such that S_(3) is the position of first maximum above the central maximum and S_(4) is the closest position where intensity is same as that of the ligth used, below the central maximum Point O is equidistant from S_(1) and S_(2) and O' is equidistant from S_(3) and S_(4) . Then intensity of inciden light is I_(0) Find the intensity at O' (on the screen).

View Solution

In the arrangement shown in figure, light of wavelength 6000 Å is incident on slits S_(1) and S_(2) have been opened such that S_(3) is the position of first maximum above the central maximum and S_(4) is the closest position where intensity is same as that of the ligth used, below the central maximum Point O is equidistant from S_(1) and S_(2) and O' is equidistant from S_(3) and S_(4) . Then intensity of inciden light is I_(0) Find the intensity of the brightest fringe.

View Solution

Consider the situation shown in fig. The two slits $S_{1}$ and $S_{2}$ placed symmetrically around the central line are illuminated by monochromatic light of wavelength $λ$ . The separation between the slit is d. The ligth transmitted by the slits falls on a screen $S_{0}$ placed at a distance D form the slits. The slit $S_{3}$ is at the central line and the slit $S_{4}$ is at a distance z from $S_{3}$ Another screen $S_{c}$ is placed a further distance D away from $S_{c}$ Find the ratio of the maximum to minimum intensity observed on $S_{c}$
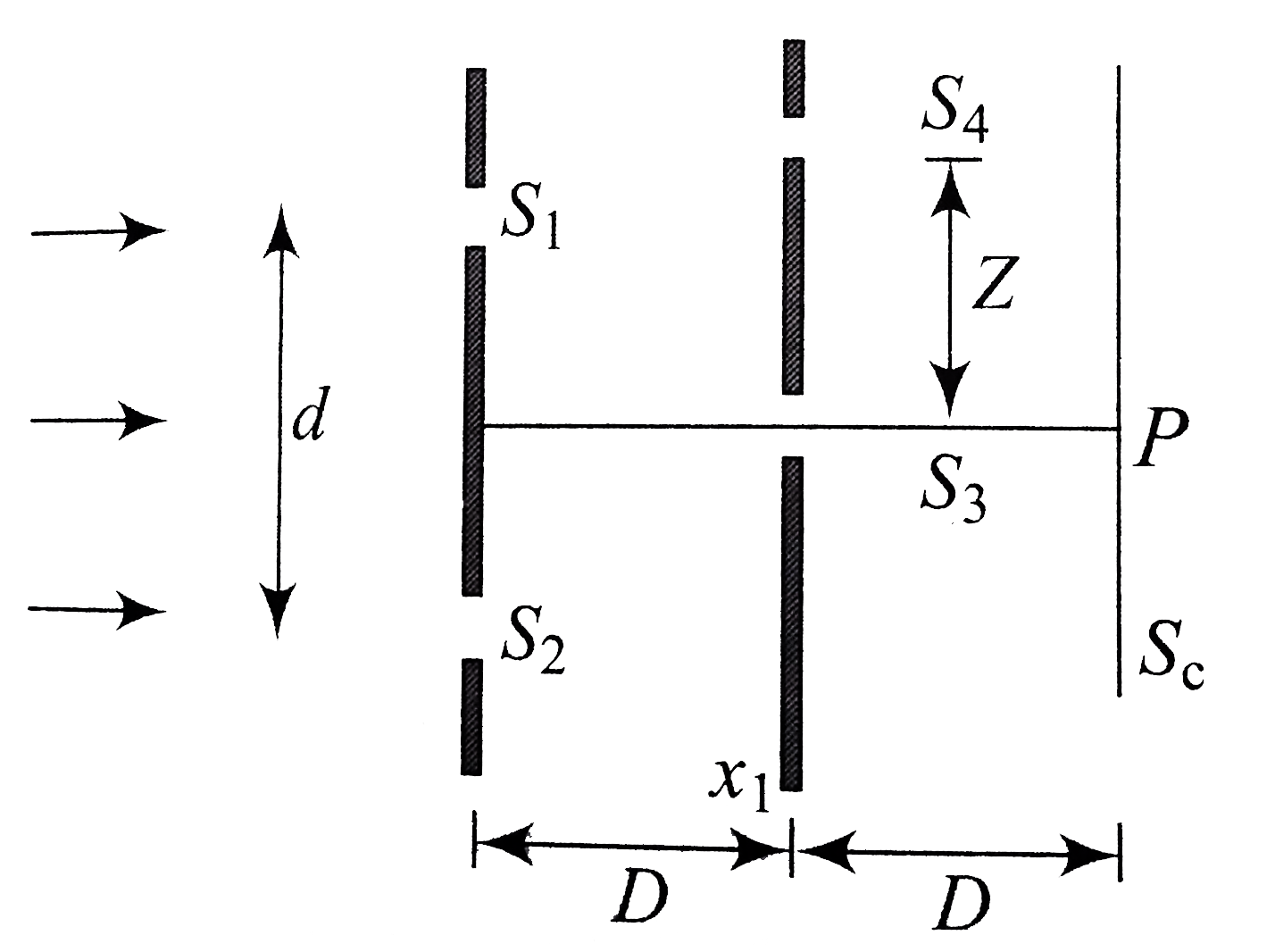
ocr_image
If $z = \frac{λ D}{4 d}$

View Solution

In the arrangement shown in Fig., slits $S_{1}$ and $S_{4}$ are having a variable separation Z. Point O on the screen is at the common perpendicular bisector of $S_{1} S_{2}$ and $S_{3} S_{4}$ .
ocr_image
When $Z = \frac{λ D}{2 d}$ , the intensity measured at O is $I_{0}$ . The intensity at O When $Z = \frac{2 λ D}{d}$ is

View Solution

In the arrangement shown in Fig., slits S_(1) and S_(4) are having a variable separation Z. Point O on the screen is at the common perpendicular bisector of S_(1) S_(2) and S_(3) S_(4) . The minimum value of Z for which the intensity at O is zero is

View Solution

In the arrangement shown in Fig., slits S_(1) and S_(4) are having a variable separation Z. Point O on the screen is at the common perpendicular bisector of S_(1) S_(2) and S_(3) S_(4) . If a hole is made at O' on AO' O and the slit S_(4) is closed, then the ratio of the maximum to minimum observed on screen at O , if O' S_(3) is equal to (lambda D)/(4d) , is

View Solution

A monochromatic light source of wavelength lamda is placed at S. three slits S_(1),S_(2) and S_(3) are equidistant from the source S and the point P on the screen S_(1)P-S_(2)P=lamda//6 and S_(1)P-S_(3)P=2lamda//3 . if I be the intensity at P when only one slit is open the intensity at P when all the three slits are open is-

View Solution

In arrangement shown in figure, plane wavefront of monochromatic light of wavelength $λ$ is incident on identical slits $S_{1}$ and $S_{2}$ . There is another pair of indentical slits $S_{3}$ and $S_{4}$ which are having separation $Z = \frac{λ D}{2 d}$ Point O is on the screen at the common perpendicular bisector of $S_{1} S_{2}$ and $S_{3} S_{4} . I_{1}$ is the intensity at point O Now the board having slits $S_{3} S_{4}$ is moved upward parallel to itself and perpendicular to line AO till slit $S_{4}$ is on line AO and it is observed that now intensity at point O is $I_{2}$ then $\frac{I_{2}}{I_{1}}$ is :
ocr_image

View Solution

Parallel monochromatic beam is falling normally on two slits S_(1) and S_(2) separated by d as shown in figure. By some mechanism, the separation between the slits S_(3) and S_(4) can be changed. The intensity is measured at the point P which is at the common perpendicular bisector of S_(1)S_(2) and S_(3)S_(4) . When z=(Dlambda)/(2d) , the intensity measured at P is I. and when z is equal to (4Dlambda)/(d) , Intensity is x l. Find x.

View Solution

In the figure shown S_(1)O-S_(2)O=S_(3)O-S_(2)O=(lambda)/(4) , Intensity at O due to any one of the slits is I_(0) . What is the intensity due to all the three coherent sources S_(1), S_(2) and S_(3) ?

View Solution

Consider the arrangement shown in figure, By some mechanism, the separation between the slits $S_{3}$ and $S_{4}$ can be changed. The intensity is measured at the point P which is at the common perpendicular bisector of $S_{1} S_{2}$ and $S_{3} S_{4}$ . When $z = \frac{D λ}{2 d}$ , the intensity measured at P is I. Find this intensity when z is equal to 1) $\frac{D λ}{d}$ , (2) $\frac{3 D λ}{2 d}$ and (3) $\frac{2 D λ}{d}$ .
ocr_image

View Solution

The correct Answer is:A

At $S_{3}$ & $S_{4}$
$Δ X = \frac{λ}{4}, Δ ϕ = \frac{π}{2}$
$I_{S_{3}} = I_{0} + I_{0} = I_{S_{4}}, I_{1} = 4 (2 I_{0}) = 8 I_{0}$
In final situation
$I_{S_{3}} = 0 I_{S_{4}} = 4 I_{0}$
$I_{2} = 4 I_{0}$
$∴ \frac{I_{2}}{I_{1}} = \frac{1}{2}$ .

Topper's Solved these Questions

Similar Questions

RESONANCE ENGLISH-DAILY PRACTICE PROBLEM-DPP No.54

The correct Answer is:A

At S3 & S4 ΔX=λ4,Δϕ=π2 IS3=I0+I0=IS4,I1=4(2I0)=8I0 In final situation IS3=0 IS4=4I0 I2=4I0 ∴I2I1=12.

Topper's Solved these Questions

Similar Questions

RESONANCE ENGLISH-DAILY PRACTICE PROBLEM-DPP No.54

At $S_{3}$ & $S_{4}$
$Δ X = \frac{λ}{4}, Δ ϕ = \frac{π}{2}$
$I_{S_{3}} = I_{0} + I_{0} = I_{S_{4}}, I_{1} = 4 (2 I_{0}) = 8 I_{0}$
In final situation
$I_{S_{3}} = 0 I_{S_{4}} = 4 I_{0}$
$I_{2} = 4 I_{0}$
$∴ \frac{I_{2}}{I_{1}} = \frac{1}{2}$ .