What is the amount of power delivered by the ac source in the circuit shown (in watts)

500 watt
1014 watt
1514 watt
2013 watt
The correct Answer is:C
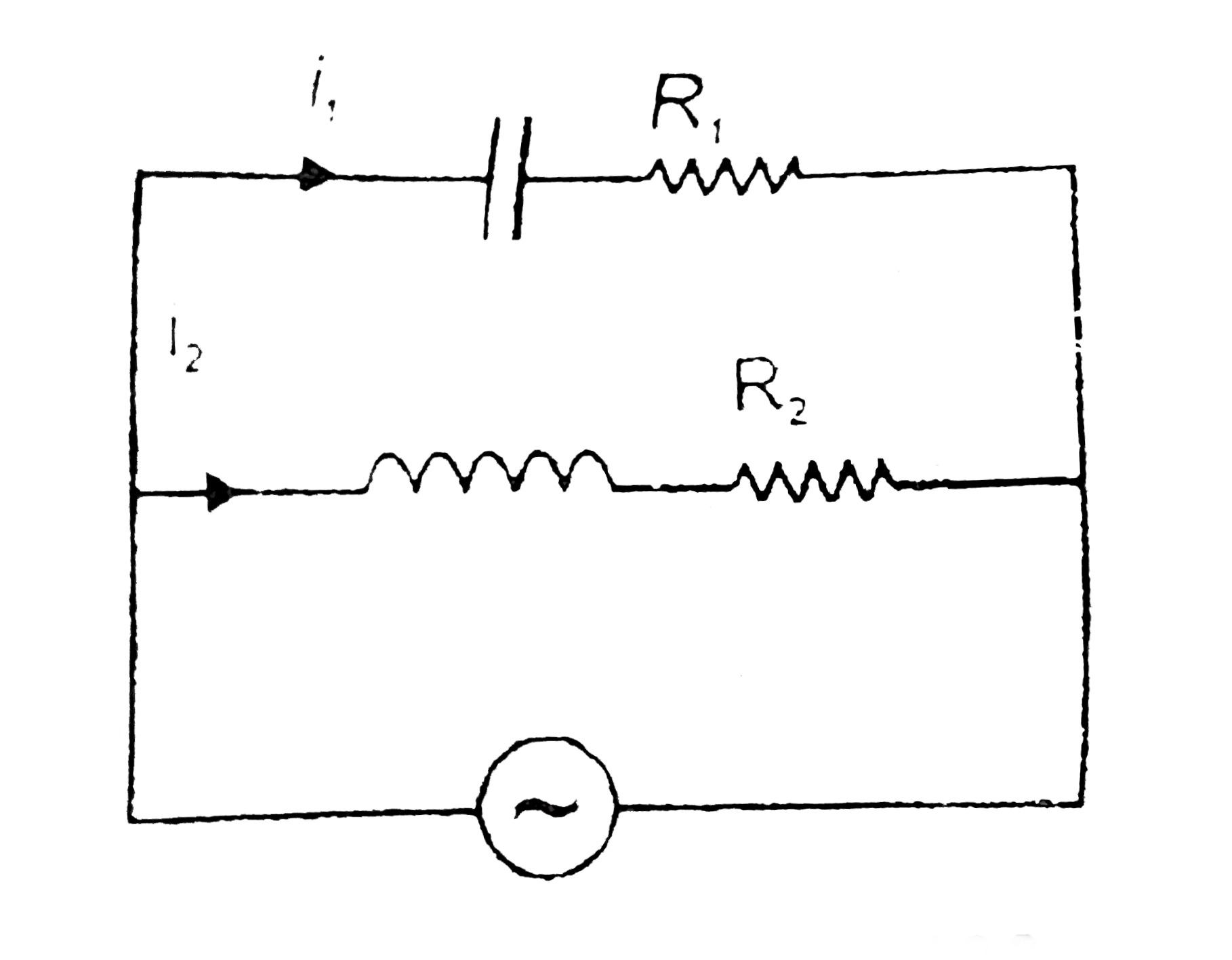
I1rms=Erms√X2C+R21=13013=10A
i2rms=Erms√X2L+R22=13A
Power dissipated
=i21rmsR1+R22rmsR2=102×5+132×6
=Power delivered by battery
=500+169×6=1514 watt.
Topper's Solved these Questions
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.54
9 Videos
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.55
9 Videos
- DAILY PRACTICE PROBLEMBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.52
9 Videos
- CURRENT ELECTRICITYBOOK - RESONANCE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - High Level Problems (HIP)
19 Videos
- ELECTRO MAGNETIC WAVESBOOK - RESONANCE ENGLISHCHAPTER - ELECTRO MAGNETIC WAVESEXERCISE - Exercise 3
27 Videos
Similar Questions
Power delivered by the source of the circuit becomes maximum , when :
View SolutionIn an L−C−R ereis circuit R=150Ω,L=0.0750H and C=0.0180μF . The source has voltage amplitude V=150V and a frequencey equal to the resonacne frequency of the circuit.
(a) What is the power factor ?
(b) What is the average power delivered by the source?
(c) The capacitor is replaced by one with C=0.0360μF and the source frequency is adjusted to the new resonance value. Then, what is the average power delivered by the source?
View Solution
Knowledge Check
The power factor of the circuit as shown in figure is
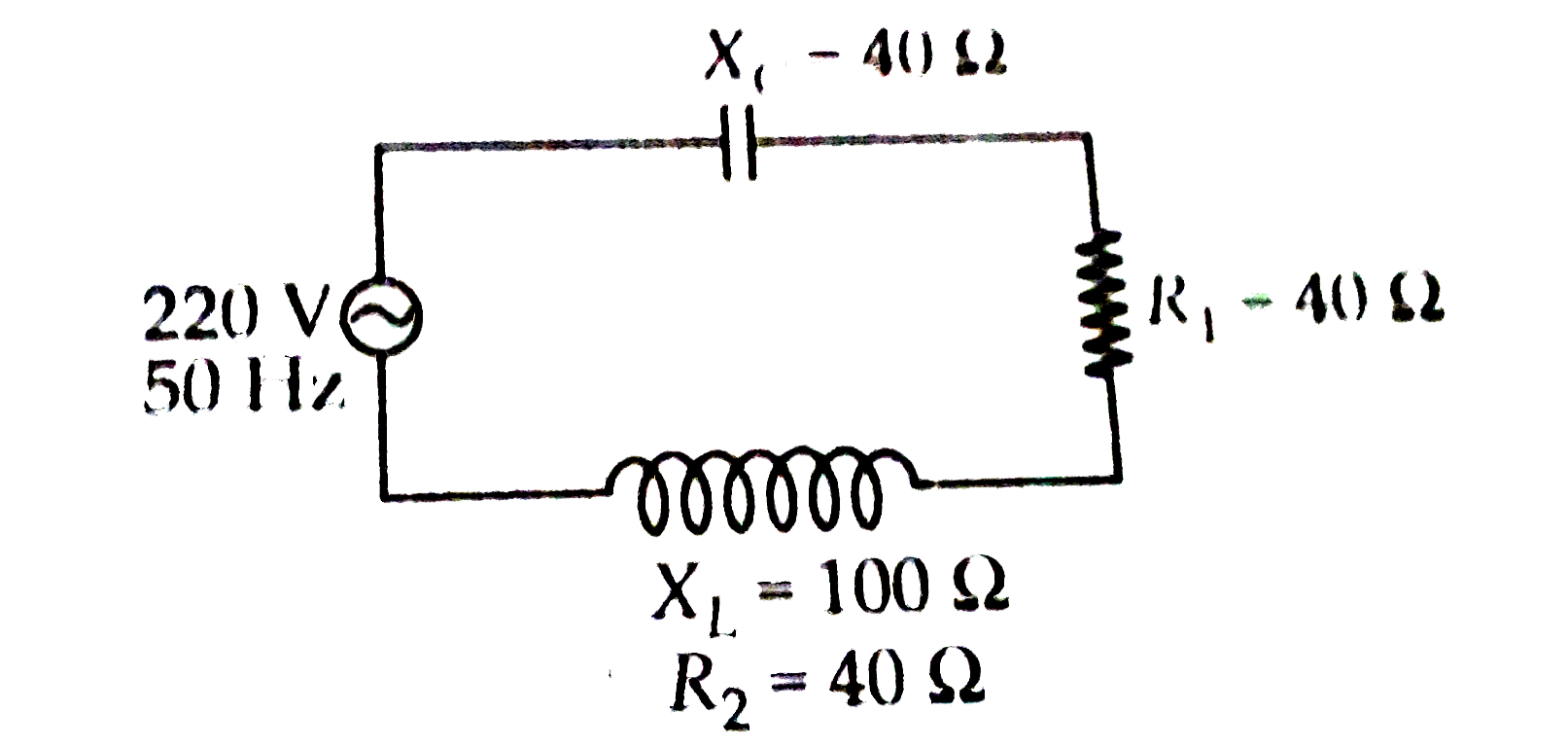 A0.2B0.4C0.8D0.6
A0.2B0.4C0.8D0.6
Power factor of the circuit shown here is

View SolutionIf the power dissipated in the 27Ω resistor in the circuit shown is 108 watt, the potential difference across the 4Ω resistor is
View SolutionThe power factor of the circuit shown in the figure is
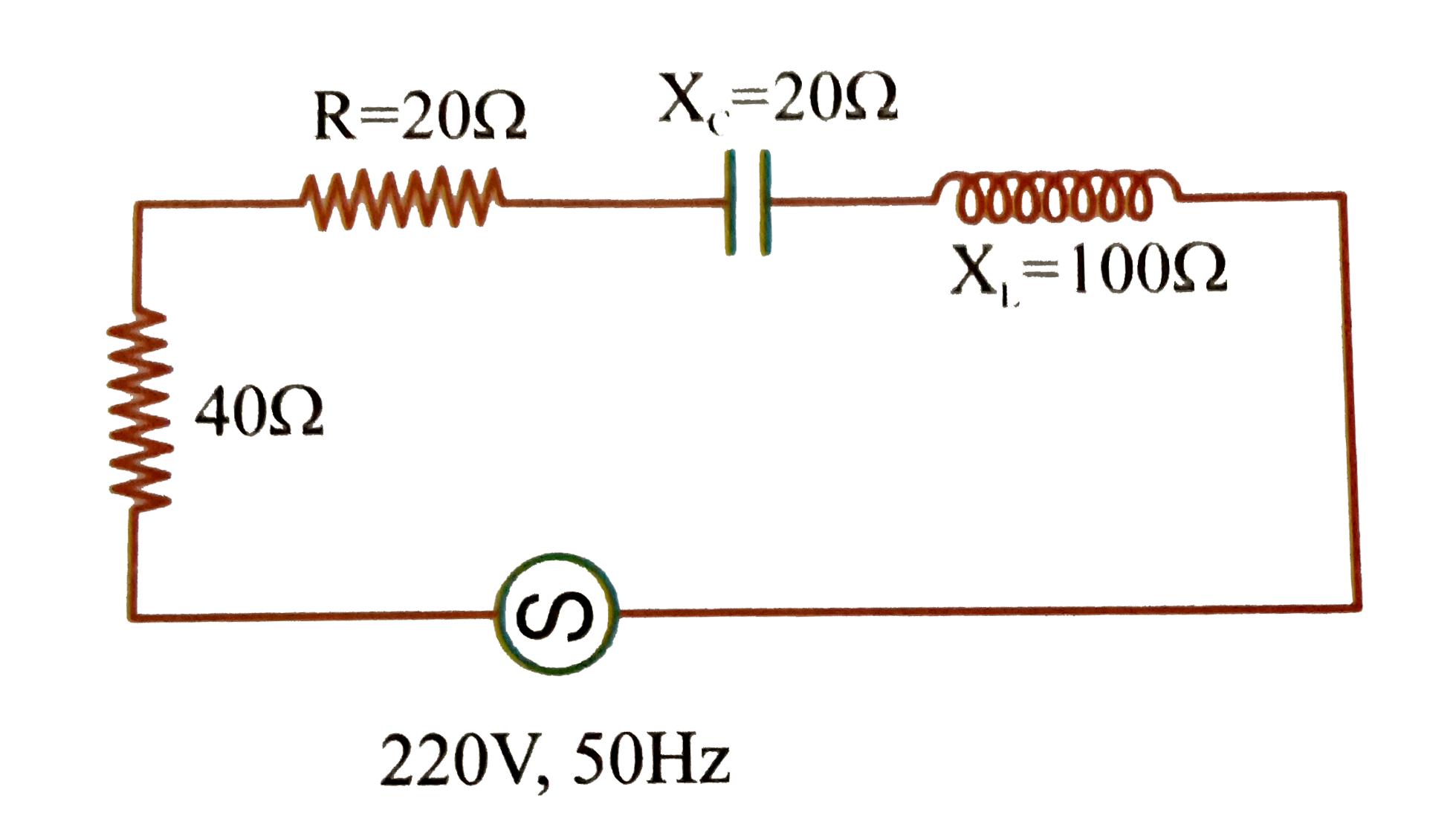
View SolutionThe total power dissipated in watts in the circuit shown here is

View SolutionWhat is the power factor for the circuit shown ?
View SolutionWhat is the power factor for the circuit shown ?

View SolutionWhat is the ratio of powers delivered by 20 V dc and 20 V peak ac to the same load resistance?
View Solution

