Two block with heat capacities C1 and C2 are connected by a rod of length l, cross-sectional area A and heat conductivity K. Initial temperature difference between the two blocks I T0 . Assuming the entire system to be isolated from surroundings , heat capacity of the rod of be negligible. The temperature difference between the blocks as a function of time is.
The correct Answer is:ΔT=(ΔT)0e−αt, where α=(1/C1+1/C2)SK/l
dQdt=Ksl(T1−T2)
where T1 and T2 are temperature of two chunks are function of time 't'. ltbRgt −C1dT1dt=Ksl(T1−T2)
C1dT2dt=Ksl(T1−T2)
or −dT1dt=KslC1(T1−T2)
or dT2dt=KslC2(T1−T2) ltbgt or −d(T1−T2)dt=Ksl(1C1+1C2)
or −ΔT∫ΔT0d(T1−T2)(T1−T2)=Ksl[1C1+1C2]t∫0dt
ΔT=ΔT0e−αt
where α=(1/C1±1/C2)SK/l
Topper's Solved these Questions
Similar Questions
Two bodies of masses m1 and m2 and specific heat capacities S1 and S2 are connected by a rod of length l, cross-section area A, thermal conductivity K and negligible heat capacity. The whole system is thermally insulated. At time t=0 , the temperature of the first body is T1 and the temperature of the second body is T2(T2>T1) . Find the temperature difference between the two bodies at time t.
View SolutionTwo bodies of masses m1 and m2 and specific heat capacities S1 and S2 are connected by a rod of length l, cross-section area A, thermal conductivity K and negligible heat capacity. The whole system is thermally insulated. At time t=0 , the temperature of the first body is T1 and the temperature of the second body is T2(T2>T1) . Find the temperature difference between the two bodies at time t.
View Solution
Knowledge Check
Two chunks of metal with heat capacities C1 and C2, are interconnected by a rod length l and cross-sectional area S and fairly low heat conductivity K. The whole system is thermally insulated from the environment. At a moment t=0 the temperature difference betwene the two chunks of metal equals (ΔT)0. Assuming the heat capacity of the rod to be negligible, find the temperature difference between the chucks as a function of time.
AT0exp(−KA(C1+C2)tC1C2)BT0exp(−KA(C1+C2)C1C2)CT0exp(KA(C1+C2)tC1C2)DT0exp(KA(C1+C2)t2C1C2)
Two identical rods of length (L), area of cross-sectional (A) and thermal conductivity k are joined end to end. If temperature difference of free ends is ΔT, the heat Q0 flows along rods per second. Find the total heat flowing per second along the rods if the two rods are placed parallel and temperature difference of free ends is ΔT. There is no heat loss from curved surface.
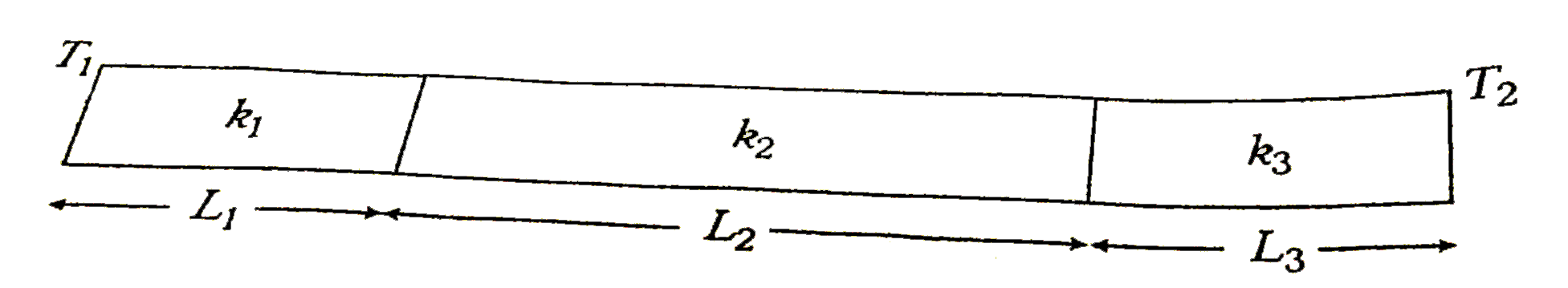
View SolutionThree rods of same cross-section but different length and conductivity are joined in series . If the temperature of the two extreme ends are T1andT2(T1>T2) find the rate of heat transfer H.
View SolutionFour identical rods AB, CD, CF and DE are joined as shown in figure. The length, cross-sectional area and thermal conductivity of each rod are l, A and K respectively. The ends A, E and F are maintained at temperature T1 , T2 and T3 respectively. Assuming no loss of heat to the atmosphere, find the temperature at B.

View SolutionFour identical rods AB,CD,CFandDE are joined as shown in figure. The length, cross-sectional area and thermal conductivity of each rod are l,AandK respectively. The ends A,EandF are maintained at temperature T1 , T2 and T3 respectively. Assuming no loss of heat to the atmosphere, find the temperature at B.

View SolutionA metal rod of length 'L' and cross-sectional area 'A' is heated through 'T'∘C What is the force required to prevent the expansion of the rod lengthwise ?
View SolutionWhat are the two specific heat capacities of a gas and why are they different? Find the difference between the two molar heat capacities in terms of the constant R.
View SolutionDifferentiate between heat capacity and specific heat capacity.
View Solution