Calculated thermal conductance for radial flow of a spherical sheel of inner and outer radius r1and r2. Assume that thermal conductivity of the material is K
The correct Answer is:4πkr1r2(r2−r1)
To calculate the thermal conductance G for the radial flow of a spherical shell with inner radius r1 and outer radius r2, and given thermal conductivity K, we can follow these steps:
Step 1: Understand the Concept of Thermal Resistance
The thermal resistance R for a spherical shell can be expressed as:
R=LKA
where:
- L is the thickness of the shell,
- K is the thermal conductivity,
- A is the surface area.
Step 2: Define the Variables
For a spherical shell, the thickness dR is an infinitesimal change in radius, and the surface area A at a radius r is given by:
A=4πr2
Step 3: Express the Thermal Resistance
The thermal resistance dR for an infinitesimal thickness dR can be expressed as:
dR=dRK⋅4πr2
Step 4: Integrate the Thermal Resistance
To find the total thermal resistance from r1 to r2, we integrate:
R=∫r2r1dRK⋅4πr2
This simplifies to:
R=1K⋅4π∫r2r11r2dR
Step 5: Solve the Integral
The integral of 1r2 is:
∫1r2dR=−1r
Thus, we have:
R=1K⋅4π(−1r)r2r1
Evaluating the limits gives:
R=1K⋅4π(−1r2+1r1)=1K⋅4π(1r1−1r2)
Step 6: Calculate the Thermal Conductance
The thermal conductance G is the reciprocal of the thermal resistance R:
G=1R=K⋅4π1r1−1r2
This can be simplified further:
G=4πKr1r2r2−r1
Final Result
Thus, the thermal conductance for the radial flow of a spherical shell is:
G=4πKr1r2r2−r1
The thermal resistance R for a spherical shell can be expressed as:
R=LKA
where:
- L is the thickness of the shell,
- K is the thermal conductivity,
- A is the surface area.
For a spherical shell, the thickness dR is an infinitesimal change in radius, and the surface area A at a radius r is given by:
A=4πr2
The thermal resistance dR for an infinitesimal thickness dR can be expressed as:
dR=dRK⋅4πr2
To find the total thermal resistance from r1 to r2, we integrate:
R=∫r2r1dRK⋅4πr2
This simplifies to:
R=1K⋅4π∫r2r11r2dR
The integral of 1r2 is:
∫1r2dR=−1r
Thus, we have:
R=1K⋅4π(−1r)r2r1
Evaluating the limits gives:
R=1K⋅4π(−1r2+1r1)=1K⋅4π(1r1−1r2)
The thermal conductance G is the reciprocal of the thermal resistance R:
G=1R=K⋅4π1r1−1r2
This can be simplified further:
G=4πKr1r2r2−r1
Thus, the thermal conductance for the radial flow of a spherical shell is:
G=4πKr1r2r2−r1
Topper's Solved these Questions
Similar Questions
Calculate thermal conductance for radial flow of an annular cylinder of length l and inner and outer radius r1 and r2. Assume that thermal conductivity of the material is K

View SolutionA cylinder of radius R is surrounded by a cylindrical shell of inner radius R and oputer radius 2R. The thermal conductivity of the material of the inner cylinder is k1 and that of the outer cylinder is K2 ,Assumming no loss of heat , the effective thermal conductivity of the system for heat flowing along the length of the cylinder is :
View SolutionA cylinder of radius r made of a material of thermal conductivity K1 is surrounded by a cylindrical shell of inner radius r and outer radius 2r made of a material of thermal conductivity K2. The two ends of the combined system are maintained at two different temperatures. There is no loss of heat across the cylindrical surface and the system is in steady state. Show that the effective thermal conductivity of the system is (K1+3K2)/4.
View SolutionA cylinder of radius R made of a material of thermal conductivity K1 is surrounded by a cylindrical shell of inner radius R and outer radius 2R made of a material of thermal conductivity K2. The two ends of the combined system are maintained at two different temperatures. There is no loss of heat across the cylindrical surface and the system is in steady state. The effective thermal conductivity of the system is
View SolutionA cylinder of radius R made of a material of thermal conductivity K1 is surrounded by a cylindrical shell of inner radius R and outer radius 2R made of a material of thermal conductivity K2. The two ends of the combined system are maintained at two different temperatures. There is no loss of heat across the cylindrical surface and the system is in steady state. The effective thermal conductivity of the system is
(a) K1+K2 (b) K1K2/(K1+K2)
(c )(K1+3K2)/4
(d) (3K1+K2)/4.
View SolutionA cylinder of radius R made of a material of thermal conductivity K1 is surrounded by cylindrical shell of inner radius R and outer radius 2R made of a material of thermal con-ductivity K2 The two ends of the combined system are maintained at two differnet tem-peratures There is no loss of heat across the cylindrical surface and system is in steady state What is the effective thermal conductivity of the system
 .
.
View SolutionA point source of heat of power P is placed at the centre of a spherical shell of mean radius R. The material of the shell has thermal conductivity K. If the temperature difference between the outer and inner surface of the shell in not to exceed T, the thickness of the shell should not be less than .......
View SolutionFind the rate of heat flow through a cross section of the rod shown in figure (θ2>θ1) . Thermal conductivity of the material of the rod is K.

View SolutionA slab consists of two layers of different materials of the same thickness and having thermal conductivities K1 and K2. The equivalent thermal conductivity of the slab is
View SolutionThermal conductivity of inner core of radius r is K and of the outer one of radius 2r is 2K. Find equivalent value of thermal conductivity between its two ends.
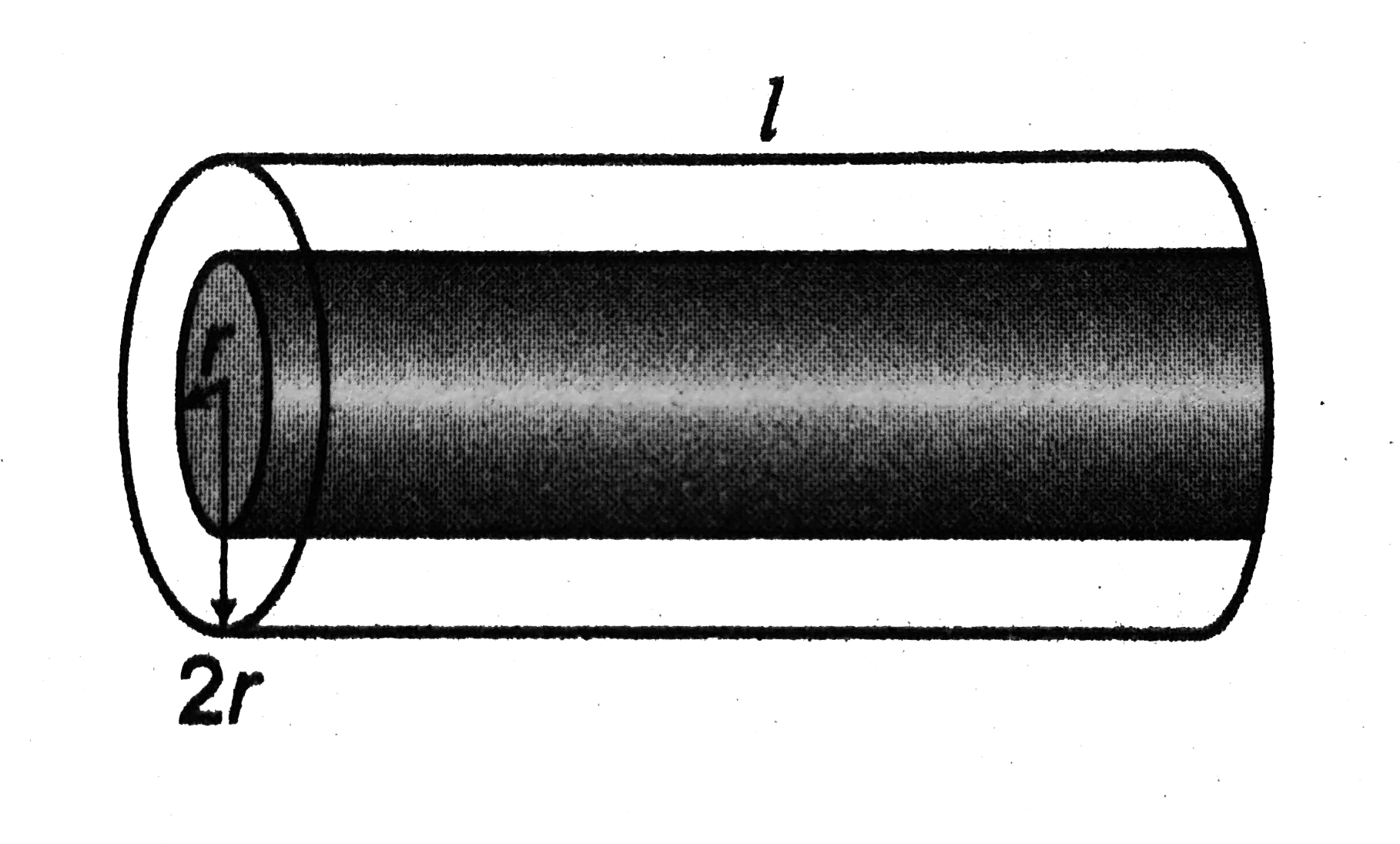
View Solution
