One end of a copper rod of uniform cross section and length 1.5 m is kept in contact with ice and the other end with water at . At what point along its length should a temperature of be maintained so that in the steady state, the mass of ice melting be equal to that of the steam produced in same interval of time. Assume that the whole system is insulated from surroundings:
The correct Answer is:10
To solve the problem step by step, we will analyze the heat transfer through the copper rod and set up equations based on the heat absorbed by ice and the heat released by water.
Step 1: Understand the setup
We have a copper rod of length 1.5 m with one end at 0°C (ice) and the other end at 100°C (water). We need to maintain a temperature of 200°C at some point along the rod such that the mass of ice melted equals the mass of steam produced.
Step 2: Define variables
Let:
- x = distance from the 100°C end to the 200°C point.
- L=1.5m=150cm (length of the rod).
- Lf=80cal/g (latent heat of fusion for ice).
- Lv=540cal/g (latent heat of vaporization for steam).
The length from the ice to the 200°C point is 1.5−x.
Step 3: Calculate heat transfer to ice
The heat Q1 transferred to the ice can be expressed as:
Q1=KA(200−0)T1.5−x
This heat is used to melt the ice:
Q1=m1Lf
where m1 is the mass of ice melted.
Step 4: Calculate heat transfer to water
The heat Q2 transferred to the water can be expressed as:
Q2=KA(200−100)Tx
This heat is used to convert water to steam:
Q2=m2Lv
where m2 is the mass of steam produced.
Step 5: Equate the masses
Since the mass of ice melted equals the mass of steam produced, we have:
m1=m2
Step 6: Substitute and simplify
From the equations for Q1 and Q2:
1. m1=KA(200)TLf(1.5−x)
2. m2=KA(100)TLvx
Setting m1=m2:
KA(200)T80(1.5−x)=KA(100)T540x
Canceling KAT from both sides:
20080(1.5−x)=100540x
Step 7: Cross-multiply and solve for x
Cross-multiplying gives:
200⋅540x=100⋅80(1.5−x)
Expanding both sides:
108000x=8000(1.5−x)
108000x=12000−8000x
Combining like terms:
108000x+8000x=12000
116000x=12000
x=12000116000=12116=0.1034m=10.34cm
Step 8: Conclusion
The distance from the 100°C end to the point where the temperature is maintained at 200°C is approximately 10.34 cm.
---
We have a copper rod of length 1.5 m with one end at 0°C (ice) and the other end at 100°C (water). We need to maintain a temperature of 200°C at some point along the rod such that the mass of ice melted equals the mass of steam produced.
Let:
- x = distance from the 100°C end to the 200°C point.
- L=1.5m=150cm (length of the rod).
- Lf=80cal/g (latent heat of fusion for ice).
- Lv=540cal/g (latent heat of vaporization for steam).
The heat Q1 transferred to the ice can be expressed as:
Q1=KA(200−0)T1.5−x
This heat is used to melt the ice:
Q1=m1Lf
where m1 is the mass of ice melted.
The heat Q2 transferred to the water can be expressed as:
Q2=KA(200−100)Tx
This heat is used to convert water to steam:
Q2=m2Lv
where m2 is the mass of steam produced.
Since the mass of ice melted equals the mass of steam produced, we have:
m1=m2
From the equations for Q1 and Q2:
1. m1=KA(200)TLf(1.5−x)
2. m2=KA(100)TLvx
KA(200)T80(1.5−x)=KA(100)T540x
Canceling KAT from both sides:
20080(1.5−x)=100540x
Cross-multiplying gives:
200⋅540x=100⋅80(1.5−x)
Expanding both sides:
108000x=8000(1.5−x)
108000x=12000−8000x
Combining like terms:
108000x+8000x=12000
116000x=12000
x=12000116000=12116=0.1034m=10.34cm
The distance from the 100°C end to the point where the temperature is maintained at 200°C is approximately 10.34 cm.
Topper's Solved these Questions
- HEAT TRANSFERBOOK - RESONANCE ENGLISHCHAPTER - HEAT TRANSFEREXERCISE - Exercise-2
1 Videos
- HEAT TRANSFERBOOK - RESONANCE ENGLISHCHAPTER - HEAT TRANSFEREXERCISE - Exercise-3
1 Videos
- HEAT TRANSFERBOOK - RESONANCE ENGLISHCHAPTER - HEAT TRANSFEREXERCISE - Exercise-1
1 Videos
- GRAVITATIONBOOK - RESONANCE ENGLISHCHAPTER - GRAVITATIONEXERCISE - Exercise
21 Videos
- KINEMATICSBOOK - RESONANCE ENGLISHCHAPTER - KINEMATICSEXERCISE - Exercise
65 Videos
Similar Questions
One end of a steel rod (K=46Js−1m−1C−1) of length 1.0m is kept in ice at 0∘C and the other end is kept in boiling water at 100∘C . The area of cross section of the rod is 0.04cm2 . Assuming no heat loss to the atmosphere, find the mass of the ice melting per second. Latent heat of fusion of ice =3.36×105Jkg−1 .
View SolutionOne end of a brass rod of length 2.0 m and cross section 1cm2 is kept in steam at 100∘C and the other end in ice at 0∘C. The lateral surface of the rod is covered by heat insulator. Determine the amount of ice melting per minute. Thermal conductivity of brass is 110W/m−K and specific latent heat of fusion of ice is 80cal/g.
View SolutionOne end of a metal rod is dipped in boiling water and the other is dipped in melting ice.
View SolutionA block of ice with a lead shot embedded in it is floating on water contained in a vessel. The temperature of the system is maintained at 0∘C as the ice melts. When the ice melts completely the level of water in the vessel rises.
View SolutionOne end of a copper rod of length 1 m and area of cross - section 400×(10−4)m2 is maintained at 100∘C. At the other end of the rod ice is kept at 0∘C. Neglecting the loss of heat from the surrounding, find the mass of ice melted in 1h. Given, KCu=401W/m−KandLf=3.35×(105)J/kg.
View SolutionThree rods each of same length and cross - section are joined in series. The thermal conductivity of the materials are K, 2K and 3K respectively. If one end is kept at 200∘C and the other at 100∘C. What would be the temperature of the junctions in the steady state? Assume that no heat is lost due to radiation from the sides of the rods.
View SolutionTwo ends of a conducting rod of varying cross-section are maintained at 200∘C and 0∘C respectively. In steady state:
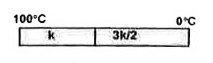
View SolutionTwo rods of similar area of cross section and length are joined as shown in the figure. The ratio of their thermal conductivity is 2:3. The free ends are maintained at 100∘C and 0∘C respectivley. THE temperature at junction in steady state is
View SolutionEqual masses of ice (at0∘C) and water are in contact. Find the temperature of water needed to just melt the complete ice.
View SolutionOne end of a copper rod of length 1.0 m and area of cross-section 10−3 is immersed in boiling water and the other end in ice. If the coefficient of thermal conductivity of copper is 92cal/m−s−.∘C and the latent heat of ice is 8×104cal/kg, then the amount of ice which will melt in one minute is
View Solution
