Consider a hypotheticla gas with molecules that can move along only a single axis. The following table givesfour situations. The velocities is meter per seocond of such a gas having four molecules. The plus and minus sign refer to the direction of the velcoity along the axis.
Situation Velocities–––––––––––––––––––––––––––––––––––––––––a -2 +3 -4 +5−−−−−−−−−−−−−b +1 -3 +4 -6−−−−−−−−−−−−−c +2 +3 +4 +5−−−−−−−−−−−−−d +3 +3 -4 -5−−−−−−−−−−−−−
In which situation root-square speed of the molecules is greatest
a
b
c
d
The correct Answer is:B
To determine which situation has the greatest root mean square (RMS) speed of the molecules, we will calculate the RMS speed for each situation provided in the question. The formula for the root mean square speed is given by:
VRMS=√V21+V22+V23+V24N
where V1,V2,V3,V4 are the velocities of the molecules, and N is the total number of molecules (which is 4 in this case).
Step-by-step Calculation:
1. Situation A: Velocities = -2, +3, -4, +5
VRMS=√(−2)2+(3)2+(−4)2+(5)24
=√4+9+16+254=√544=√13.5≈3.674
2. Situation B: Velocities = +1, -3, +4, -6
VRMS=√(1)2+(−3)2+(4)2+(−6)24
=√1+9+16+364=√624=√15.5≈3.937
3. Situation C: Velocities = +2, +3, +4, +5
VRMS=√(2)2+(3)2+(4)2+(5)24
=√4+9+16+254=√544=√13.5≈3.674
4. Situation D: Velocities = +3, +3, -4, -5
VRMS=√(3)2+(3)2+(−4)2+(−5)24
=√9+9+16+254=√594=√14.75≈3.843
Summary of RMS Speeds:
- Situation A: ≈3.674
- Situation B: ≈3.937
- Situation C: ≈3.674
- Situation D: ≈3.843
Conclusion:
The situation with the greatest root mean square speed is Situation B with an RMS speed of approximately 3.937.
VRMS=√(−2)2+(3)2+(−4)2+(5)24
=√4+9+16+254=√544=√13.5≈3.674
VRMS=√(1)2+(−3)2+(4)2+(−6)24
=√1+9+16+364=√624=√15.5≈3.937
VRMS=√(2)2+(3)2+(4)2+(5)24
=√4+9+16+254=√544=√13.5≈3.674
VRMS=√(3)2+(3)2+(−4)2+(−5)24
=√9+9+16+254=√594=√14.75≈3.843
- Situation A: ≈3.674
- Situation B: ≈3.937
- Situation C: ≈3.674
- Situation D: ≈3.843
The situation with the greatest root mean square speed is Situation B with an RMS speed of approximately 3.937.
Topper's Solved these Questions
- KTG & THERMODYNAMICSBook:RESONANCE ENGLISHChapter:KTG & THERMODYNAMICSExercise:PART -III
Explore 25 Videos
- KTG & THERMODYNAMICSBook:RESONANCE ENGLISHChapter:KTG & THERMODYNAMICSExercise:PART -IV
Explore 9 Videos
- KTG & THERMODYNAMICSBook:RESONANCE ENGLISHChapter:KTG & THERMODYNAMICSExercise:Exercise-2
Explore 1 Video
- KINETIC THEORY OF GASES AND THERMODYNAMICSBook:RESONANCE ENGLISHChapter:KINETIC THEORY OF GASES AND THERMODYNAMICSExercise:Exercise
Explore 64 Videos
- MAGNETIC FIELD AND FORCESBook:RESONANCE ENGLISHChapter:MAGNETIC FIELD AND FORCESExercise:Exercise
Explore 64 Videos
Similar Questions
Consider a hypothetical gas with molecules that can move along only a single axis. The following table gives four situations. The velocities in meter per second of such a gas having four molecules are given below. The plus and minus sign refer to the direction of the velocity along the axis.
Situation Velocities–––––––––––––––––––––––––––––––––––––––––a -2 +3 -4 +5−−−−−−−−−−−−−b +1 -3 +4 -6−−−−−−−−−−−−−c +2 +3 +4 +5−−−−−−−−−−−−−d +3 +3 -4 -5−−−−−−−−−−−−−
In which situation root-mean-square speed of the molecules is greatest
View SolutionWrite the singificance of plus and minus signs in representing the opitals.
View SolutionWrite the significance of plus and minus sign shown in representing the orbitals.
View Solutionif we move along y-axis then find the value of abscissa.
View SolutionThe molecules in a gas move about in all directions at a high speed.
View SolutionWhich d-orbital has its four lobes along the axis?
View SolutionRoot mean square velocity of a gas molecule is proprotional to
View SolutionFind the angular momentum of a disc about the axis shown in figure in the following situations.
View SolutionIf we move along x-axis then find the value of ordinate.
View SolutionWhat is the component of the vector →a along the x-axis in the situation shown in figure ? |→a|=5 units.
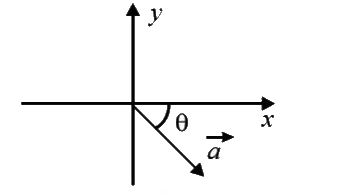
View Solution