A particle is ejected from the tube at A with a velocity V at an angle θ with the verticle y-axis at a height h above the ground as shown. A strong horizontal wind gives the particle a constant horizontal acceleration a in the positive x-direction. If the particle strikes the ground at a point directly under its released position and the downward y-acceleration is taken as g then find h.

h=2V2sinθcosθa
h=2V2sinθcosθg
h=2V2gsinθ[cosθ+agsinθ]
h=2V2asinθ[cosθ+gasinθ]
The correct Answer is:D
A particle is …………
Since x - displecement is zero
0=Vsinθt−12at2
t=2Vsinθa
h=Vcosθt+12gt2
h=2V2asinθ[cosθ+gasinθ]
Topper's Solved these Questions
- TEST PAPERSBook:RESONANCE ENGLISHChapter:TEST PAPERSExercise:PART - II PHYSICS
Explore 106 Videos
- SIMPLE HARMONIC MOTION Book:RESONANCE ENGLISHChapter:SIMPLE HARMONIC MOTION Exercise:Advanced Level Problems
Explore 13 Videos
- TEST SERIESBook:RESONANCE ENGLISHChapter:TEST SERIESExercise:PHYSICS
Explore 130 Videos
Similar Questions
A particle Is projected at point 'A' with initial velocity 5m/s at an angle θ=37∘ with the vertical y axis. A strong horizontal wind gives the particle a constant horizontal acceleration 6m/s2 in the x direction. If the particle strikes the ground at a point directly under its released position, determine the height 'h' of point A. The downward acceleration is taken as the constant
g=10m/s2(take sin37∘=35,cos37∘=45)
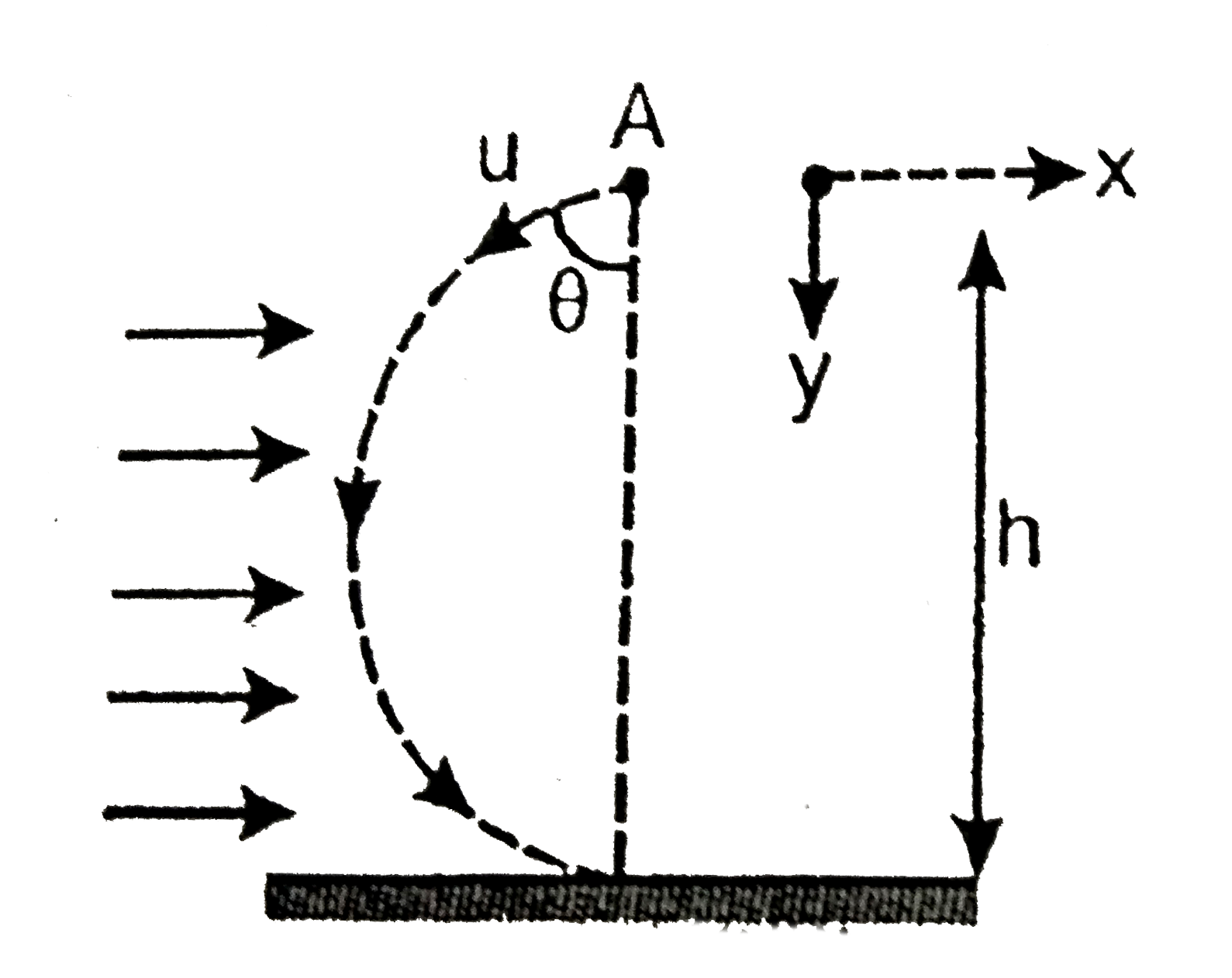
View SolutionA particle is projected from the ground with velocity u making an angle θ with the horizontal. At half of its maximum heights,
View SolutionA particle is projected from a point P with a velocity v at an angle θ with horizontal. At a certain point Q it moves at right angles to its initial direction. Then
View SolutionA particle is projected from a point P with a velocity v at an angle θ with horizontal. At a certain point Q it moves at right angles to its initial direction. Then
View SolutionA particle is fired from a point on the ground with speed u making an angle θ with the horizontal.Then
View SolutionVelocity of a particle is in negative direction with constant acceleration in positive direction. Then match the following:

View SolutionA particle is projected form ground with velocity u ar angle θ from horizontal. Match the following two columns.

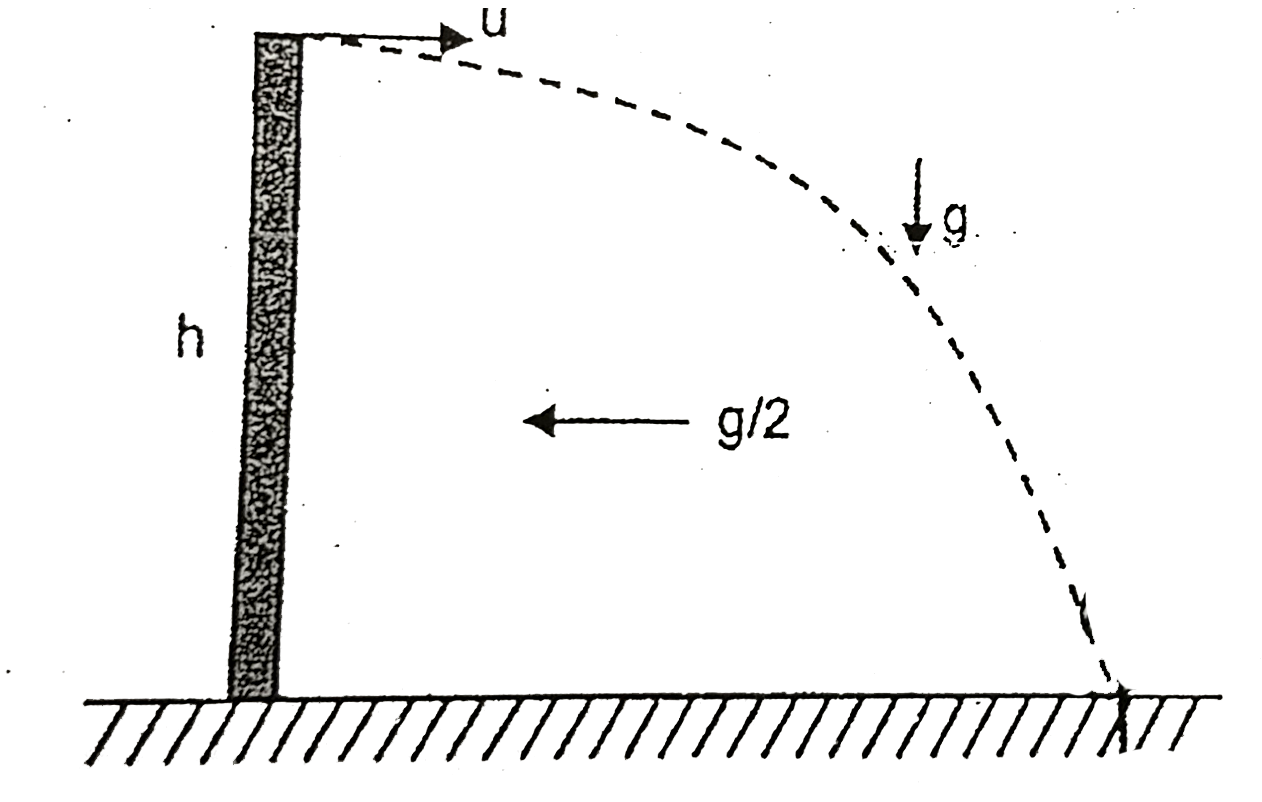
View SolutionA particle is projected horizontally from a tower with velocity u towards east. Wind provides constant horizontal acceleration g2 towards west. If particle strikes the ground vertically the then :
View SolutionA particle of mass m is moving along the line y-b with constant acceleration a. The areal velocity of the position vector of the particle at time t is (u=0)
View SolutionA particle is projected at t=0 with velocity u at angle θ with the horizontal. Then the ratio of the tangential acceleration and the radius of curvature at the point of projection is :
View Solution