The acceleration of a particle moving in straight line is defined by the relation a=−4x(−1+14x2), where a is acceleration in m/s2 and x is position in meter. If velocity v=17m/s when x=0, then the velocity of the particle when x=4 meter is :
12m/s
15m/s
20m/s
25m/s
The correct Answer is:B
To solve the problem, we need to find the velocity of a particle when its position x=4 meters, given the acceleration function and the initial conditions.
1. Understand the given acceleration equation:
The acceleration a is given by:
a=−4x(−1+14x2)
This can be rewritten as:
a=−4x+x3
2. Relate acceleration to velocity:
We know that acceleration a can also be expressed as:
a=vdvdx
where v is the velocity of the particle. Therefore, we can set up the equation:
vdvdx=−4x+x3
3. Separate variables and integrate:
Rearranging gives:
vdv=(−4x+x3)dx
Now, we integrate both sides. The left side integrates to:
∫vdv=12v2
The right side integrates as follows:
∫(−4x+x3)dx=−2x2+14x4+C
4. Set up the equation after integration:
After integration, we have:
12v2=−2x2+14x4+C
5. Use initial conditions to find C:
We know that when x=0, v=17m/s:
12(17)2=−2(0)2+14(0)4+C
This simplifies to:
12×289=C⟹C=144.5
6. Substitute C back into the equation:
Now our equation becomes:
12v2=−2x2+14x4+144.5
7. Find the velocity when x=4:
Substitute x=4:
12v2=−2(4)2+14(4)4+144.5
Calculate the right side:
=−2(16)+14(256)+144.5=−32+64+144.5=176.5
So,
12v2=176.5
Multiplying both sides by 2 gives:
v2=353
Therefore, taking the square root:
v=√353≈18.79m/s
Final Answer
The velocity of the particle when x=4 meters is approximately 18.79m/s.
The acceleration a is given by:
a=−4x(−1+14x2)
This can be rewritten as:
a=−4x+x3
We know that acceleration a can also be expressed as:
a=vdvdx
where v is the velocity of the particle. Therefore, we can set up the equation:
vdvdx=−4x+x3
Rearranging gives:
vdv=(−4x+x3)dx
Now, we integrate both sides. The left side integrates to:
∫vdv=12v2
The right side integrates as follows:
∫(−4x+x3)dx=−2x2+14x4+C
After integration, we have:
12v2=−2x2+14x4+C
We know that when x=0, v=17m/s:
12(17)2=−2(0)2+14(0)4+C
This simplifies to:
12×289=C⟹C=144.5
Now our equation becomes:
12v2=−2x2+14x4+144.5
Substitute x=4:
12v2=−2(4)2+14(4)4+144.5
Calculate the right side:
=−2(16)+14(256)+144.5=−32+64+144.5=176.5
So,
12v2=176.5
Multiplying both sides by 2 gives:
v2=353
Therefore, taking the square root:
v=√353≈18.79m/s
The velocity of the particle when x=4 meters is approximately 18.79m/s.
Topper's Solved these Questions
- TEST PAPERSBook:RESONANCE ENGLISHChapter:TEST PAPERSExercise:PART - II PHYSICS
Explore 106 Videos
- SIMPLE HARMONIC MOTION Book:RESONANCE ENGLISHChapter:SIMPLE HARMONIC MOTION Exercise:Advanced Level Problems
Explore 13 Videos
- TEST SERIESBook:RESONANCE ENGLISHChapter:TEST SERIESExercise:PHYSICS
Explore 130 Videos
Similar Questions
The motion of a particle along a straight line is described by the function x=(2t−3)2, where x is in metres and t is in seconds. Find
(a) the position, velocity and acceleration at t=2s.
(b) the velocity of the particle at origin.
View SolutionThe motion of a particle along a straight line is described by the function x=(2t−3)2, where x is in metres and t is in seconds. Find
(a) the position, velocity and acceleration at t=2s.
(b) the velocity of the particle at origin.
View SolutionThe position of an object moving on a straight line is defined by the relation x=t3−2t2−4t, where x is expressed in meter and t in second. Determine
(a) the average velocity during the interval of 1 second to 4 second.
(b) the velocity at t = 1 s and t = 4 s,
(c) the average acceleration during the interval of 1 second to 4 second.
(d) the acceleration at t = 4 s and t = 1 s.
View SolutionAt time t , the displacement of a particle moving in a straight line by x=−4t2+2t . Find the velocity and acceleration a when t = (1/2) s .
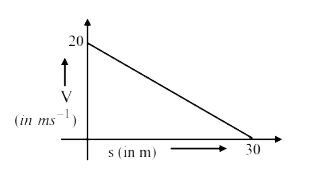
View SolutionThe velocity –position graph of a particle moving along a straight line is shown. Then acceleration of the particle at s = 15 m is :
View SolutionThe speed v of a particle moving along a straight line is given by a+bv2=x2 where x is its distance from the origin. The acceleration of the particle is
View SolutionThe motion of a particle along a straight line is described by equation : x=8+12t−t3 where x is in meter and t in second. The retardation of the particle when its velocity becomes zero is.
View SolutionThe displacement 'x' and time of travel 't' for a particle moving an a straight line are related as t=√(x+1)(x−1). Its acceleration at a time t is
View SolutionThe velocity of a particle moving on the x-axis is given by v=x2+x, where x is in m and v in m/s. What is its position (in m) when its acceleration is 30m/s2.
View SolutionIf v=x2−5x+4, find the acceleration of the particle when velocity of the particle is zero.
View Solution