A ball of mass 'm' is suspended from a point with a massless string of length l in form of a pendulum. This ball is given a horizontal velocity √4gl at bottom most point. When string makes an angle 60∘ from lower vertical, acat=p. write the value of p2. (g=10m/s2)
To solve the problem, we will follow these steps:
Step 1: Analyze the System
We have a pendulum with a mass m suspended from a point with a massless string of length l. The ball is given an initial horizontal velocity of v0=√4gl at the lowest point.
Step 2: Determine the Velocity at 60∘
When the pendulum makes an angle of 60∘ with the vertical, we need to find the velocity of the ball at this position. We can use the conservation of mechanical energy.
The initial kinetic energy at the lowest point is:
KEi=12mv20=12m(4gl)=2mgl
The potential energy at the lowest point is zero.
At the angle 60∘:
- The height h of the ball above the lowest point can be calculated using:
h=l−lcos(60∘)=l−l2=l2
- The potential energy at this height is:
PE=mgh=mg(l2)=mgl2
Using conservation of energy:
KEi+PEi=KEf+PEf
2mgl+0=12mv2+mgl2
Cancelling m from all terms:
2gl=12v2+gl2
Multiplying through by 2:
4gl=v2+gl
Rearranging gives:
v2=4gl−gl=3gl
Step 3: Calculate the Centripetal Acceleration
The centripetal acceleration ac at this point is given by:
ac=v2l=3gll=3g
Step 4: Calculate the Tangential Acceleration
The tangential acceleration at is due to the component of gravitational force acting along the direction of motion when the pendulum is at 60∘:
at=gsin(60∘)=g⋅√32=√3g2
Step 5: Find the Ratio acat
Now we can find the ratio p:
p=acat=3g√3g2=3g⋅2√3g=6√3=2√3
Step 6: Calculate p2
Finally, we calculate p2:
p2=(2√3)2=4⋅3=12
Final Answer
Thus, the value of p2 is:
12
We have a pendulum with a mass m suspended from a point with a massless string of length l. The ball is given an initial horizontal velocity of v0=√4gl at the lowest point.
When the pendulum makes an angle of 60∘ with the vertical, we need to find the velocity of the ball at this position. We can use the conservation of mechanical energy.
KEi=12mv20=12m(4gl)=2mgl
The potential energy at the lowest point is zero.
- The height h of the ball above the lowest point can be calculated using:
h=l−lcos(60∘)=l−l2=l2
- The potential energy at this height is:
PE=mgh=mg(l2)=mgl2
KEi+PEi=KEf+PEf
2mgl+0=12mv2+mgl2
Cancelling m from all terms:
2gl=12v2+gl2
Multiplying through by 2:
4gl=v2+gl
Rearranging gives:
v2=4gl−gl=3gl
The centripetal acceleration ac at this point is given by:
ac=v2l=3gll=3g
The tangential acceleration at is due to the component of gravitational force acting along the direction of motion when the pendulum is at 60∘:
at=gsin(60∘)=g⋅√32=√3g2
Now we can find the ratio p:
p=acat=3g√3g2=3g⋅2√3g=6√3=2√3
Finally, we calculate p2:
p2=(2√3)2=4⋅3=12
Thus, the value of p2 is:
12
Topper's Solved these Questions
Similar Questions
A bob of mass m suspended by a light inextensible string of length 'l' from a fixed point. The bob is given a speed of √6gl. Find the tension in the string when string deflects through an angle 120∘ from the vertical.
View SolutionAn object of mass 10kg is connected to the lower end of a massless string of length 4m hanging from the ceiling. If a force F is applied horizontally at the mid-point of the string, the top half of the string makes an angle of 45∘ with the vertical , then the magnitude of F is
View SolutionA uniform rod of mass m and length L is suspended with two massless strings as shown in the figure. If the rod is at rest in a horizontal position the ratio of tension in the two strings T1T2 is:

View SolutionA bob of mass 2kg is suspended from point O of a core with an inextensible string strinng of length √3m. It is moving in horizontal circle over the surface of cone as shown in the figure. Then :(g=10m/s2)
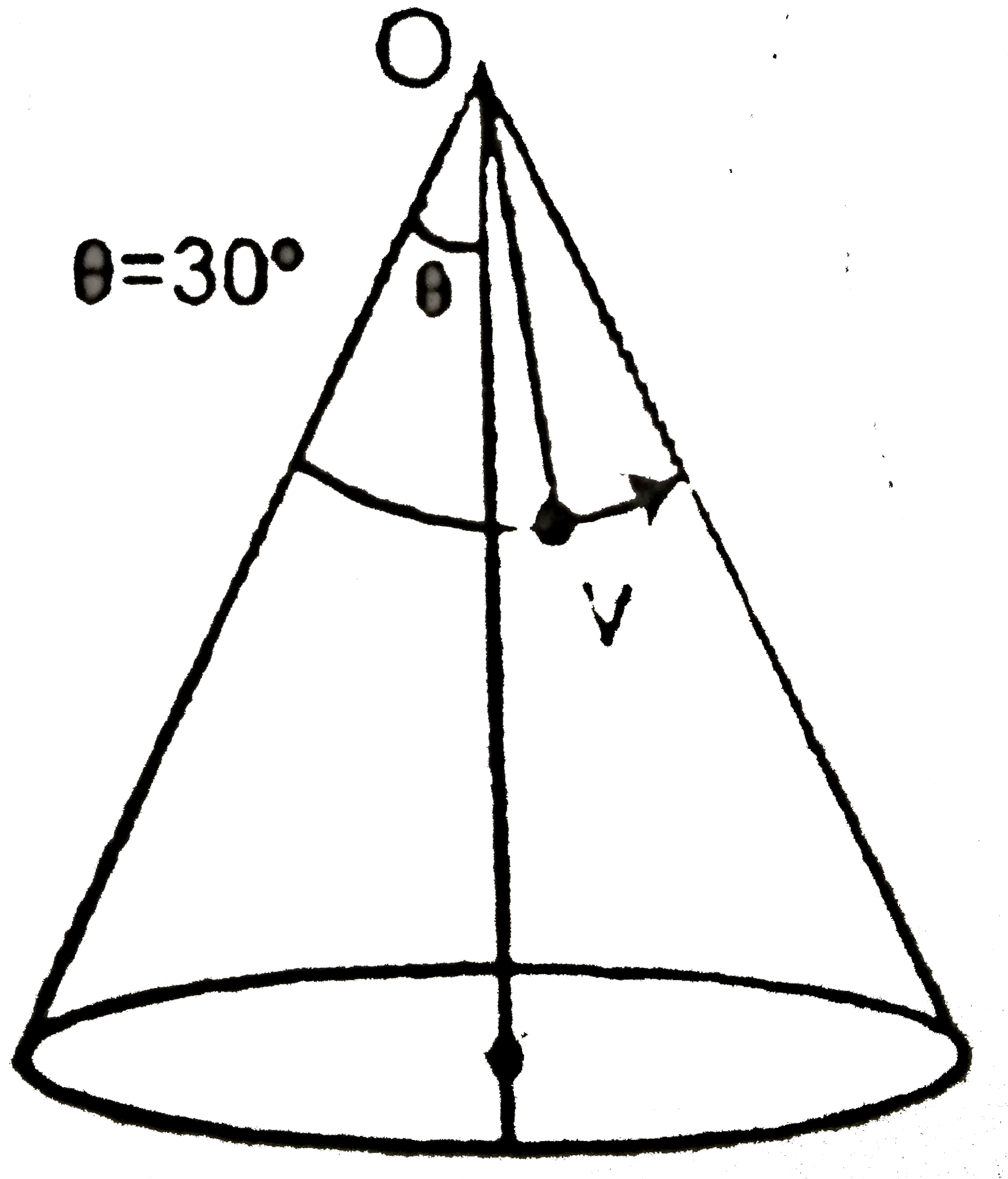
View SolutionA bob of mass 2kg is suspended from point O of a core with an inextensible string strinng of length √3m. It is moving in horizontal circle over the surface of cone as shown in the figure. Then :(g=10m/s2)

View SolutionA particle of mass 200 g , is whirled into a vertical circle of radius 80 cm uisig a massless string The speed of the particle when the string makes an angle of 60∘ with the vertical line is 1.5ms−1. The tension in the string at this position is
View SolutionA particle of mass m is suspended by a string of length l from a fixed rigid support. A sufficient horizontal velocity =√3gl is imparted to it suddenly. Calculate the angle made by the string with the vertical when the accekleration of the particle is inclined to the string by 45∘ .
View SolutionA smooth ball of mass m is suspended from a light string of length 1m. Another ball of mass 2m strikes the ball of mass in horizontally with a speed of u=√35m/s. The coefficient of restitution for the collision is e. The string becomes loose, when it makes an angle of 30∘ with the horizontal, find the value of e.

View SolutionA particle suspended by a light inextensible thread of length l is projected horizontally from its lowest position with velocity √7gl/2. The string will slack after swinging through an angle equal to
View SolutionA bob of mass 1 kg is suspended from an inextensible string of length 1 m. When the string makes an angle 60∘ with vertical, speed of the bob is 4 m/s
Net acceleration of the bob at this instant is
View Solution
