A circle, with centre O, circumscribs a pentagon ABCDE. IF AB−BC=CDand∠BCD=126∘, find:
∠AOC
The correct Answer is:108∘
To solve the problem step by step, we will find the angle AOC in the given pentagon ABCDE circumscribed by a circle with center O.
1. Identify Given Information:
- The pentagon ABCDE is circumscribed by a circle with center O.
- We know that AB−BC=CD.
- We are given that ∠BCD=126∘.
2. Establish Relationships in Triangles:
- In triangles BOC and COD, we note that:
- OC is common to both triangles.
- OB=OD (radii of the circle).
- Since AB−BC=CD, we can infer that BC=CD.
3. Use the Given Angle:
- Since ∠BCD=126∘, we can find angles OCB and OCD:
- By the properties of the circle, ∠OCB=∠OCD=12∠BCD.
- Thus, ∠OCB=∠OCD=126∘2=63∘.
4. Establish Angles in Triangle BOC:
- In triangle BOC:
- OB=OC (both are radii).
- Therefore, ∠OCB=∠OBC (angles opposite to equal sides).
- Hence, ∠OBC=63∘.
5. Apply the Angle Sum Property:
- The sum of angles in triangle BOC gives us:
∠OBC+∠OCB+∠BOC=180∘
- Substituting the known values:
63∘+63∘+∠BOC=180∘
- This simplifies to:
126∘+∠BOC=180∘
- Therefore,
∠BOC=180∘−126∘=54∘
6. Relate Angles AOB and COD:
- Since AB=BC=CD, we have:
∠AOB=∠BOC=∠COD=54∘
7. Find Angle AOC:
- Now, we can find ∠AOC:
∠AOC=∠AOB+∠BOC=54∘+54∘=108∘
Final Answer:
∠AOC=108∘
- The pentagon ABCDE is circumscribed by a circle with center O.
- We know that AB−BC=CD.
- We are given that ∠BCD=126∘.
- In triangles BOC and COD, we note that:
- OC is common to both triangles.
- OB=OD (radii of the circle).
- Since AB−BC=CD, we can infer that BC=CD.
- Since ∠BCD=126∘, we can find angles OCB and OCD:
- By the properties of the circle, ∠OCB=∠OCD=12∠BCD.
- Thus, ∠OCB=∠OCD=126∘2=63∘.
- In triangle BOC:
- OB=OC (both are radii).
- Therefore, ∠OCB=∠OBC (angles opposite to equal sides).
- Hence, ∠OBC=63∘.
- The sum of angles in triangle BOC gives us:
∠OBC+∠OCB+∠BOC=180∘
- Substituting the known values:
63∘+63∘+∠BOC=180∘
- This simplifies to:
126∘+∠BOC=180∘
- Therefore,
∠BOC=180∘−126∘=54∘
- Since AB=BC=CD, we have:
∠AOB=∠BOC=∠COD=54∘
- Now, we can find ∠AOC:
∠AOC=∠AOB+∠BOC=54∘+54∘=108∘
∠AOC=108∘
Topper's Solved these Questions
Similar Questions
A circle, with centre O, circumscribs a pentagon ABCDE. IF AB−BC=CDand∠BCD=126∘, find:
∠AEB
View SolutionA circle, with centre O, circumscribs a pentagon ABCDE. IF AB−BC=CDand∠BCD=126∘, find:
∠AED
View SolutionIn the given AB=BC=DCand∠AOB=50∘
∠AOC
View SolutionIn cyclic quadrilateral ABCD , AD = BC, ∠BAC=30∘and∠CBD=70∘ , find : angle ADC .
View SolutionABCDE is a cyclic pentagon with centre of its circumcircle alt point O such that AB=BC=CD and angle ABC=120∘
Calculate
(i) ∠BEC (ii) ∠BED
View SolutionIn the given 'O' is the centre of the circle, Arc AB=ArcBC=Cd.If∠OAB=48∘, find:
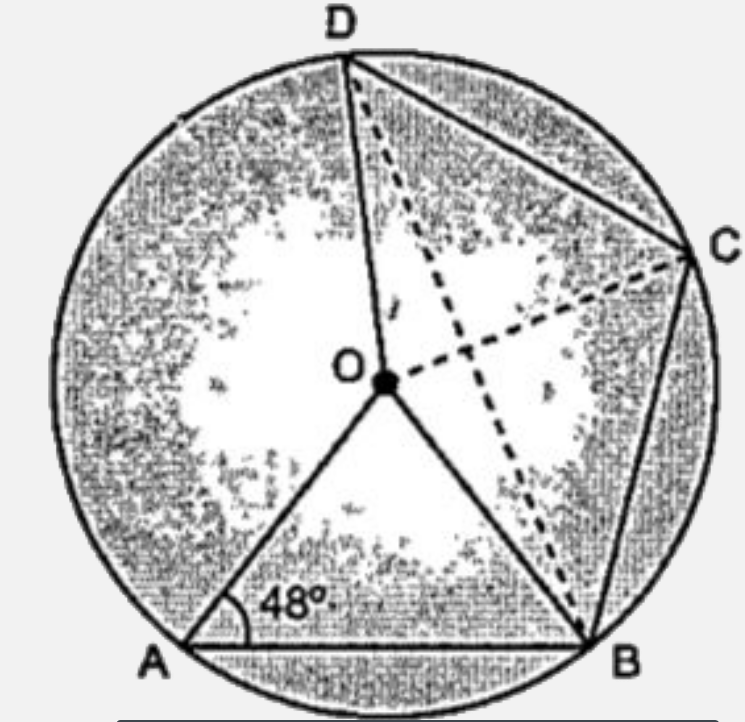
∠OBD
View SolutionIn cyclic quadrilateral ABCD , AD = BC ,∠BAC=30∘and∠CBD=70∘ , find :
∠BCD
View SolutionIn cyclic quadrilateral ABCD , AD = BC ,∠BAC=30∘and∠CBD=70∘ , find :
∠BCA
View SolutionIn the figure given alongside ,AB and CD are straight lines thorugh the centre O of a circle. If ∠AOC=80∘and∠CDE=40∘ , find ∠DCE

View SolutionIn circle given below . O is its centre and lengths of chords AB and CD are in the ratio 5 :3
If angle AOB = 100∘, find :
(i)∠COD

View Solution