The angle of elevation of the top of a tower. from a point on the ground and at a distance of 160 m from its foot, is find to be 60∘ . Find the height of the tower .
277.12 m
377.12 m
477.12 m
None
The correct Answer is:A
To find the height of the tower given the angle of elevation and the distance from the tower, we can follow these steps:
1. Draw a Diagram:
- Let the tower be represented as line segment PQ, where P is the top of the tower and Q is the base of the tower.
- Let point A be the point on the ground from where the angle of elevation is measured.
- The distance from point A to the foot of the tower (point Q) is given as 160 meters.
2. Identify the Angle of Elevation:
- The angle of elevation from point A to the top of the tower (point P) is given as 60∘.
3. Set Up the Right Triangle:
- In triangle PQA, we have:
- PQ as the height of the tower (which we need to find).
- AQ as the distance from point A to the base of the tower, which is 160 m.
- Angle PAQ (angle of elevation) is 60∘.
4. Use the Tangent Function:
- The tangent of an angle in a right triangle is defined as the ratio of the opposite side (height of the tower, PQ) to the adjacent side (distance from the tower, AQ).
- Therefore, we can write:
tan(60∘)=PQAQ
- Substituting the known values:
tan(60∘)=H160
5. Substitute the Value of tan(60∘):
- We know that tan(60∘)=√3≈1.732.
- Thus, the equation becomes:
√3=H160
6. Solve for H (Height of the Tower):
- Rearranging the equation to solve for H:
H=160×√3
- Now, substituting the approximate value of √3≈1.732:
H=160×1.732
- Calculating the height:
H≈277.12 meters
7. Final Answer:
- The height of the tower is approximately 277.12 meters.
- Let the tower be represented as line segment PQ, where P is the top of the tower and Q is the base of the tower.
- Let point A be the point on the ground from where the angle of elevation is measured.
- The distance from point A to the foot of the tower (point Q) is given as 160 meters.
- The angle of elevation from point A to the top of the tower (point P) is given as 60∘.
- In triangle PQA, we have:
- PQ as the height of the tower (which we need to find).
- AQ as the distance from point A to the base of the tower, which is 160 m.
- Angle PAQ (angle of elevation) is 60∘.
- The tangent of an angle in a right triangle is defined as the ratio of the opposite side (height of the tower, PQ) to the adjacent side (distance from the tower, AQ).
- Therefore, we can write:
tan(60∘)=PQAQ
- Substituting the known values:
tan(60∘)=H160
- We know that tan(60∘)=√3≈1.732.
- Thus, the equation becomes:
√3=H160
- Rearranging the equation to solve for H:
H=160×√3
- Now, substituting the approximate value of √3≈1.732:
H=160×1.732
- Calculating the height:
H≈277.12 meters
- The height of the tower is approximately 277.12 meters.
Topper's Solved these Questions
- HEIGHTS AND DISTANCES BOOK - ICSECHAPTER - HEIGHTS AND DISTANCES EXERCISE - Exercise 22 B
19 Videos
- HEIGHTS AND DISTANCES BOOK - ICSECHAPTER - HEIGHTS AND DISTANCES EXERCISE - Exercise 22 C
28 Videos
- HEIGHTS AND DISTANCES BOOK - ICSECHAPTER - HEIGHTS AND DISTANCES EXERCISE - Exercise 22 C
28 Videos
- GST [GOODS AND SERVICES TAX] BOOK - ICSECHAPTER - GST [GOODS AND SERVICES TAX] EXERCISE - Exercise 1(B)
16 Videos
- LINEAR INEQUATIONSBOOK - ICSECHAPTER - LINEAR INEQUATIONSEXERCISE - Competency Based Questions
15 Videos
Similar Questions
The angle elevation of the top of a tower from a point C on the ground. Which is 30 m away from the foot of the tower is 30∘ . Find the height of the tower.

View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 40 m away from the foot of the tower is 30∘. Find the height of the tower.
View Solution
Knowledge Check
The angle of elevation of the top of a tower. from a point on the ground and at a distance of 160 m from its foot, is find to be 60∘ . Find the height of the tower .
A277.12 mB377.12 mC477.12 mDNone
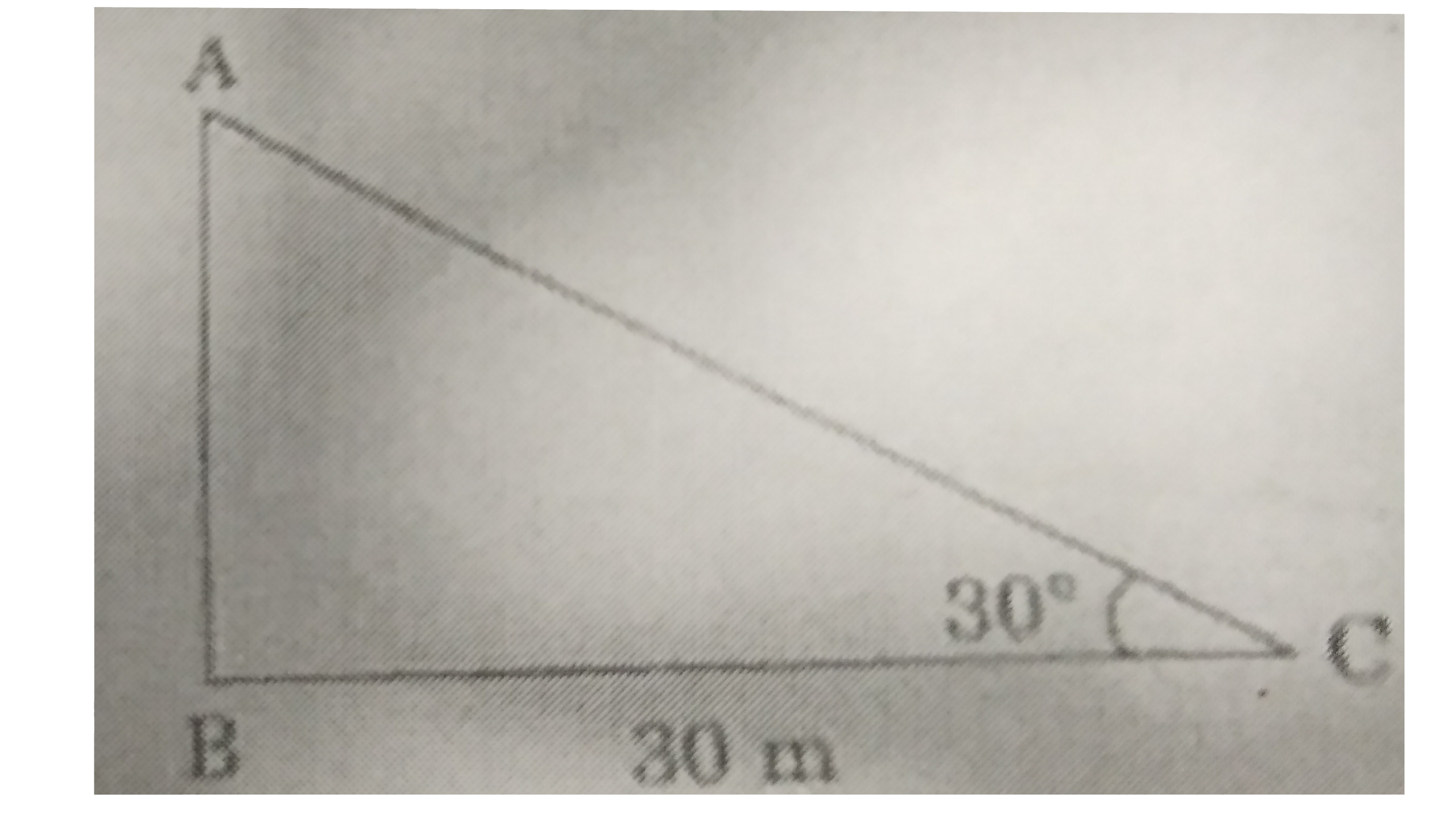
If figure, the angle of elevation of the top of a tower from a point C on the ground which is 30 m away from the foot of the tower, is 30∘. Find th height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30m away from the foot of the tower is 30∘ . Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30m away from the foot of the tower, is 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground, which is 30 m away from the foot of the tower is 30∘. Find the height of the tower.
View SolutionThe angle of elevation of the top of a tower from a point on the ground is 60∘. which is 25 m away from the foot of the tower. Find the height of the tower
View SolutionThe angle of elevation of the top of a tower from the foot of a house, situated at a distance of 20 m from the tower is 60∘. From the top of the top of the house the angle of elevation of the top of the tower os 45∘. Find the height of house and tower.
View SolutionThe angle of elevation of the of a tower at a distance of 120 m from its foot on a horizontal plane is found to be 30∘ . Find the height of the tower.
View Solution
