In the following figure , angle B=90∘∠ADB=30∘and∠ACB=60∘
If ∠CD=m find AB .
(##SEL_RKB_ICSE_MAT_IX_CR_02_E01_162_Q01.png" width="80%">
The correct Answer is:20√3m=34.64m
To solve for the length of AB in the given triangle configuration, we will follow these steps:
Step 1: Identify the given information
We have:
- ∠B=90∘
- ∠ADB=30∘
- ∠ACB=60∘
- CD=40meters
Step 2: Set up the triangle dimensions
Let:
- BC=X
- AB=Y
Step 3: Use the tangent function in triangle ABD
In triangle ABD, we can use the tangent of angle ADB:
tan(30∘)=ABBD
Since BD=BC+CD=X+40:
tan(30∘)=YX+40
We know that tan(30∘)=1√3, so we have:
1√3=YX+40
Cross-multiplying gives us:
Y=1√3(X+40)
This can be rewritten as:
√3Y=X+40(Equation 1)
Step 4: Use the tangent function in triangle ABC
In triangle ABC, we can use the tangent of angle ACB:
tan(60∘)=ABBC
Thus:
tan(60∘)=YX
Since tan(60∘)=√3, we have:
√3=YX
Cross-multiplying gives us:
Y=√3X(Equation 2)
Step 5: Substitute Equation 2 into Equation 1
Substituting Y from Equation 2 into Equation 1:
√3(√3X)=X+40
This simplifies to:
3X=X+40
Subtracting X from both sides:
2X=40
Dividing by 2:
X=20
Step 6: Find Y using Equation 2
Now substituting X back into Equation 2 to find Y:
Y=√3×20=20√3
Step 7: Calculate the numerical value of Y
Calculating Y:
Y≈20×1.732=34.64meters
Final Answer
Thus, the length of AB is:
AB≈34.64meters
---
We have:
- ∠B=90∘
- ∠ADB=30∘
- ∠ACB=60∘
- CD=40meters
Let:
- BC=X
- AB=Y
In triangle ABD, we can use the tangent of angle ADB:
tan(30∘)=ABBD
Since BD=BC+CD=X+40:
tan(30∘)=YX+40
We know that tan(30∘)=1√3, so we have:
1√3=YX+40
Cross-multiplying gives us:
Y=1√3(X+40)
This can be rewritten as:
√3Y=X+40(Equation 1)
In triangle ABC, we can use the tangent of angle ACB:
tan(60∘)=ABBC
Thus:
tan(60∘)=YX
Since tan(60∘)=√3, we have:
√3=YX
Cross-multiplying gives us:
Y=√3X(Equation 2)
Substituting Y from Equation 2 into Equation 1:
√3(√3X)=X+40
This simplifies to:
3X=X+40
Subtracting X from both sides:
2X=40
Dividing by 2:
X=20
Now substituting X back into Equation 2 to find Y:
Y=√3×20=20√3
Calculating Y:
Y≈20×1.732=34.64meters
Thus, the length of AB is:
AB≈34.64meters
Topper's Solved these Questions
- CHAPTER REVISION (STAGE 2) Book:ICSEChapter:CHAPTER REVISION (STAGE 2) Exercise:CO- ORDINATE GEOMETRY
Explore 5 Videos
- CHAPTER REVISION (STAGE 2) Book:ICSEChapter:CHAPTER REVISION (STAGE 2) Exercise:GRAPHICAL SOLUTIONS
Explore 3 Videos
- CHAPTER REVISION (STAGE 2) Book:ICSEChapter:CHAPTER REVISION (STAGE 2) Exercise:SOLIDS
Explore 7 Videos
- AREA THEOREMSBook:ICSEChapter:AREA THEOREMSExercise:Exercies 16(C )
Explore 22 Videos
- CHAPTERWISE REVISION (STAGE 1) Book:ICSEChapter:CHAPTERWISE REVISION (STAGE 1) Exercise:Graphical solution
Explore 10 Videos
Similar Questions
In the given figure ,I is the incentre of triangle ABC . AI produced meets the circumcircle of the triangle ABC at point D. if ∠BAC=50∘and∠ABC=70∘, find :
(i) ∠BCD(ii)∠ICD(iii)∠BIC
(##SEL_RKB_ICSE_MAT_X_C17_E01_015_Q01.png" width="80%">
View SolutionIn the following figure .
(i) if ∠BAD=96∘, find ∠BCDand∠BFE.
(ii) Prove that AD is parallel to PE.
(##SEL_RKB_ICSE_MAT_X_C17_E02_019_Q01.png" width="80%">
(b) ABCD is a parallelogram. A circle through vertices A and B meets side BC at point P and side AD at point Q . Show that quadrilateral PCDQ is cyclic.
View SolutionIn the given figure ∠BAD=60∘,∠ABD=70∘∠BDC=45∘
(i) prove that AC is a diameter of the circle
(ii) Find ∠ACB
(##SEL_RKB_ICSE_MAT_X_C17_E02_002_Q01.png" width="80%">
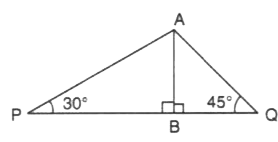
View SolutionFind PQ, if AB=150 m, ∠P=30∘and∠Q=45∘ (ii)
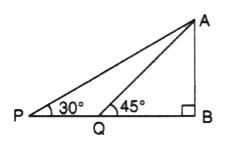
View SolutionFind PQ, if AB=150 m, ∠P=30∘and∠Q=45∘ (i)
View SolutionIn the following figure, AB = EF, BC = DE and ∠B=∠E=90∘.
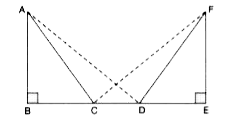
Pove that AD = FC.
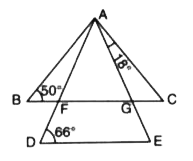
View SolutionIn the following figure , AB = BC and AD = DE.
if ∠B=50∘,∠D=66∘and∠GAC=18∘ find the measure of angles DAE, BAF and AGF.
View SolutionCalculate the angles x,y and z if
x3=y4=z5
(##SEL_RKB_ICSE_MAT_X_C17_E02_042_Q01.png" width="80%">
View SolutionIn the followoing figure , AD is the diameter of the circle with centre O. Chords AB,BC and CD are equal .If ∠DEF=110∘, Calculate :
(i) ∠AEF.
(ii) ∠FAB
(##SEL_RKB_ICSE_MAT_X_C17_E03_002_Q01.png" width="80%">
View SolutionTwo unequal circles with centres A and B intersect each other at points C and D. The centre B of the smaller circles lies on the circumference of the bigger circle with centre A. If ∠CMD=x∘, find in terms of x, the measure of angle DAC .
(##SEL_RKB_ICSE_MAT_X_C17_E01_013_Q01.png" width="80%">
View Solution
