Express 3.−2−5−3 as a fractions in the form xy where x,y∈Iandy≠0
The correct Answer is:3221990
To express the repeating decimal 3.¯¯¯¯¯¯¯¯253 as a fraction in the form xy, where x and y are integers and y≠0, we can follow these steps:
Step 1: Define the repeating decimal
Let x=3.¯¯¯¯¯¯¯¯253.
Step 2: Multiply by a power of 10
Since the repeating part "253" has 3 digits, multiply both sides of the equation by 1000 (which is 103):
1000x=3253.¯¯¯¯¯¯¯¯253
Step 3: Set up the equation
Now, we have two equations:
1. x=3.¯¯¯¯¯¯¯¯253
2. 1000x=3253.¯¯¯¯¯¯¯¯253
Step 4: Subtract the first equation from the second
Subtract the first equation from the second:
1000x−x=3253.¯¯¯¯¯¯¯¯253−3.¯¯¯¯¯¯¯¯253
This simplifies to:
999x=3253−3
Step 5: Simplify the right side
Calculate 3253−3:
999x=3250
Step 6: Solve for x
Now, divide both sides by 999:
x=3250999
Step 7: Final expression
Thus, the repeating decimal 3.¯¯¯¯¯¯¯¯253 can be expressed as the fraction:
3250999
Let x=3.¯¯¯¯¯¯¯¯253.
Since the repeating part "253" has 3 digits, multiply both sides of the equation by 1000 (which is 103):
1000x=3253.¯¯¯¯¯¯¯¯253
Now, we have two equations:
1. x=3.¯¯¯¯¯¯¯¯253
2. 1000x=3253.¯¯¯¯¯¯¯¯253
Subtract the first equation from the second:
1000x−x=3253.¯¯¯¯¯¯¯¯253−3.¯¯¯¯¯¯¯¯253
This simplifies to:
999x=3253−3
Calculate 3253−3:
999x=3250
Now, divide both sides by 999:
x=3250999
Thus, the repeating decimal 3.¯¯¯¯¯¯¯¯253 can be expressed as the fraction:
3250999
Topper's Solved these Questions
- CHAPTER REVISION (STAGE 2) Book:ICSEChapter:CHAPTER REVISION (STAGE 2) Exercise:COMPOUND INTEREST
Explore 27 Videos
- CHAPTER REVISION (STAGE 2) Book:ICSEChapter:CHAPTER REVISION (STAGE 2) Exercise:FACTRORISATIONS
Explore 10 Videos
- AREA THEOREMSBook:ICSEChapter:AREA THEOREMSExercise:Exercies 16(C )
Explore 22 Videos
- CHAPTERWISE REVISION (STAGE 1) Book:ICSEChapter:CHAPTERWISE REVISION (STAGE 1) Exercise:Graphical solution
Explore 10 Videos
Similar Questions
Knowledge Check
SiCl4H2O−−→XHeat−−→YNaOH−−−→Z
find X,Y,Z.ASiO2SiNaSiBSi(OH)4SiO2Na2SiO3CSi(OH)4SiSiO2DSiO2SiCI4Na2SiO3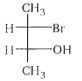 Hbr−−→(X)Nal−−−→acetone(Y)(Major), Product (Y) is :Acis-2-buteneBtrans - 2- buteneC1-buteneDIso-butene
Hbr−−→(X)Nal−−−→acetone(Y)(Major), Product (Y) is :Acis-2-buteneBtrans - 2- buteneC1-buteneDIso-butene
C2H4Br2−−→XKCN−−→Y,Y is
View SolutionSolve for xandy :
2x+3y=13,5x−4y=−2(x≠0andy≠0)
View SolutionSolve for xandy :
2x+3y=13,5x−4y=−2(x≠0andy≠0)
View SolutionSolve for xandy :
2x+3y=13,5x−4y=−2(x≠0andy≠0)
View SolutionColourless salt (X)Δ−−→(Y)Cu2+,Δ−−−−−→ coloured bead (Z). (X) can be
View SolutionSolve: 3(2x+y)=7xy
3(x+3y)=11xy,x≠0,y≠0
View Solution
