The points A(-1,2),B(x,y)and C = (4,5) are such that BA = BC . Find a linear relations between x and y .
The correct Answer is:5x+3y=18
To solve the problem where the points A(-1,2), B(x,y), and C(4,5) are such that the distance from B to A is equal to the distance from B to C (BA = BC), we will follow these steps:
Step 1: Use the Distance Formula
The distance between two points (x1,y1) and (x2,y2) is given by the formula:
d=√(x2−x1)2+(y2−y1)2
Step 2: Calculate Distance BA
For points A(-1, 2) and B(x, y), the distance BA is:
BA=√(x−(−1))2+(y−2)2=√(x+1)2+(y−2)2
Step 3: Calculate Distance BC
For points B(x, y) and C(4, 5), the distance BC is:
BC=√(4−x)2+(5−y)2
Step 4: Set Distances Equal
Since BA = BC, we set the two distances equal:
√(x+1)2+(y−2)2=√(4−x)2+(5−y)2
Step 5: Square Both Sides
To eliminate the square roots, we square both sides:
(x+1)2+(y−2)2=(4−x)2+(5−y)2
Step 6: Expand Both Sides
Now we will expand both sides:
- Left side:
(x+1)2=x2+2x+1
(y−2)2=y2−4y+4
So,
x2+2x+1+y2−4y+4=x2+y2+2x−4y+5
- Right side:
(4−x)2=16−8x+x2
(5−y)2=25−10y+y2
So,
16−8x+x2+25−10y+y2=x2+y2−8x−10y+41
Step 7: Set the Expanded Equations Equal
Now we have:
x2+2x−4y+5=x2+y2−8x−10y+41
Step 8: Cancel x2 and y2 and Rearrange
Cancel x2 and y2 from both sides:
2x−4y+5=−8x−10y+41
Rearranging gives:
2x+8x+10y−4y=41−5
10x+6y=36
Step 9: Simplify the Equation
Dividing the entire equation by 2 gives:
5x+3y=18
Final Result
The linear relation between x and y is:
5x+3y=18
The distance between two points (x1,y1) and (x2,y2) is given by the formula:
d=√(x2−x1)2+(y2−y1)2
For points A(-1, 2) and B(x, y), the distance BA is:
BA=√(x−(−1))2+(y−2)2=√(x+1)2+(y−2)2
For points B(x, y) and C(4, 5), the distance BC is:
BC=√(4−x)2+(5−y)2
Since BA = BC, we set the two distances equal:
√(x+1)2+(y−2)2=√(4−x)2+(5−y)2
To eliminate the square roots, we square both sides:
(x+1)2+(y−2)2=(4−x)2+(5−y)2
Now we will expand both sides:
- Left side:
(x+1)2=x2+2x+1
(y−2)2=y2−4y+4
So,
x2+2x+1+y2−4y+4=x2+y2+2x−4y+5
(4−x)2=16−8x+x2
(5−y)2=25−10y+y2
So,
16−8x+x2+25−10y+y2=x2+y2−8x−10y+41
Now we have:
x2+2x−4y+5=x2+y2−8x−10y+41
Cancel x2 and y2 from both sides:
2x−4y+5=−8x−10y+41
Rearranging gives:
2x+8x+10y−4y=41−5
10x+6y=36
Dividing the entire equation by 2 gives:
5x+3y=18
The linear relation between x and y is:
5x+3y=18
Topper's Solved these Questions
- CHAPTER REVISION (STAGE 2) BOOK - ICSECHAPTER - CHAPTER REVISION (STAGE 2) EXERCISE - GRAPHICAL SOLUTIONS
3 Videos
- AREA THEOREMSBOOK - ICSECHAPTER - AREA THEOREMSEXERCISE - Exercies 16(C )
22 Videos
- CHAPTERWISE REVISION (STAGE 1) BOOK - ICSECHAPTER - CHAPTERWISE REVISION (STAGE 1) EXERCISE - Graphical solution
10 Videos
Similar Questions
If the point (x,y) is equidistant from the points (2,3)and(6,−1), find the relation between xandy.
View SolutionWhat is the difference between 2.0m and 2.00m.
View SolutionThe st. lines 3x+4y=5and4x−3y=15 interrect at a point A(3,−1). On these linepoints B and C are chosen so that AB=AC. Find the possible eqns of the line BC pathrough the point (1,2)
View SolutionDraw the graph obtained from the table below:
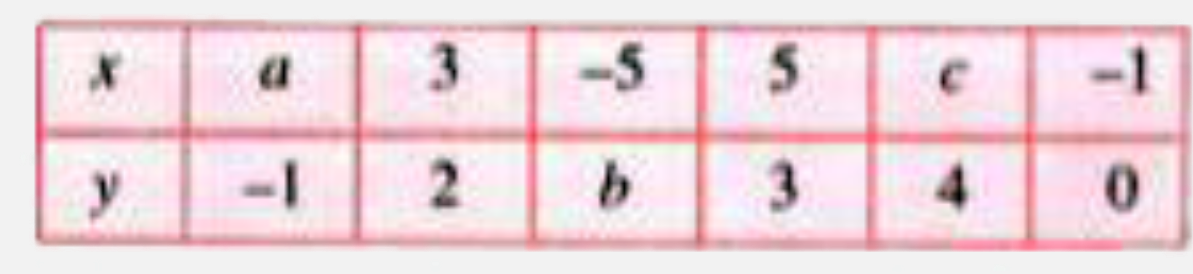
Use the graph to find the values of a,b and c. State a linear relation between the variables x and y.
View SolutionDraw the graph obtained from the table below:
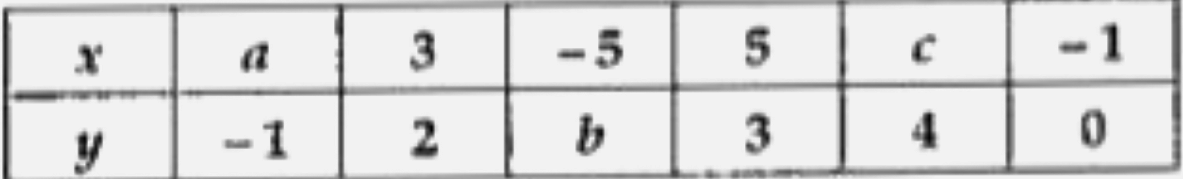
Use the graph to find the value of a,b and c. State a linear relation between the variables x and y.
View SolutionIf the points A (x,y), B (1,4) and C (-2,5) are collinear, then shown that x + 3y = 13.
View SolutionIn parallelogram ABCD , AB = (3x- 4) cm, BC = ( y- 1) cm , CD = ( y+ 5) cm and AD = (2x+ 5) cm. find the ratio AB : BC.
Find the values of x and y.
View SolutionIf the lines 3x+by−1=0 and ax−5y+2=0 are parallel, then find the relation between a and b.
View SolutionFind the linear factors of 2x2−y2−x+xy+2y−1.
View SolutionLet a, b, c be parameters. Then the equation ax+by+c=0 will represent a family of straight lines passikng through a fixed point iff there exists a linear relation between a,b, and c.
View Solution
