Calculate the pressure due to a water column of height 100 m. (Take g=10ms−2 and density of water =103kgm−3).
To calculate the pressure due to a water column of height 100 m, we can use the formula for pressure in a fluid column:
P=ρ⋅g⋅h
Where:
- P is the pressure at the bottom of the column (in Pascals or Newtons per square meter).
- ρ is the density of the fluid (in kg/m³).
- g is the acceleration due to gravity (in m/s²).
- h is the height of the fluid column (in meters).
1. Identify the given values:
- Height of the water column, h=100m
- Density of water, ρ=103kg/m3
- Acceleration due to gravity, g=10m/s2
2. Substitute the values into the pressure formula:
P=ρ⋅g⋅h
P=(103kg/m3)⋅(10m/s2)⋅(100m)
3. Perform the multiplication:
- First, calculate ρ⋅g:
ρ⋅g=103⋅10=104kg m/s2m−3
- Now multiply by the height h:
P=104⋅100=104⋅102=106N/m2
4. Final result:
P=106N/m2
Conclusion:
The pressure due to a water column of height 100 m is 106N/m2 or 1,000,000 Pascals.
- P is the pressure at the bottom of the column (in Pascals or Newtons per square meter).
- ρ is the density of the fluid (in kg/m³).
- g is the acceleration due to gravity (in m/s²).
- h is the height of the fluid column (in meters).
- Height of the water column, h=100m
- Density of water, ρ=103kg/m3
- Acceleration due to gravity, g=10m/s2
P=ρ⋅g⋅h
P=(103kg/m3)⋅(10m/s2)⋅(100m)
- First, calculate ρ⋅g:
ρ⋅g=103⋅10=104kg m/s2m−3
- Now multiply by the height h:
P=104⋅100=104⋅102=106N/m2
P=106N/m2
The pressure due to a water column of height 100 m is 106N/m2 or 1,000,000 Pascals.
Topper's Solved these Questions
- PRESSURE IN FLUIDS AND ATMOSPHERIC PRESSUREBOOK - ICSECHAPTER - PRESSURE IN FLUIDS AND ATMOSPHERIC PRESSUREEXERCISE - Exercise-4(A)
31 Videos
- PRESSURE IN FLUIDS AND ATMOSPHERIC PRESSUREBOOK - ICSECHAPTER - PRESSURE IN FLUIDS AND ATMOSPHERIC PRESSUREEXERCISE - Exercise-4(A)multiple choice
4 Videos
- MOTION IN ONE DIMENSION BOOK - ICSECHAPTER - MOTION IN ONE DIMENSION EXERCISE - EXERCISE -2 (C) ( Multiple choice type :)
17 Videos
- PROPAGATION OF SOUND WAVES BOOK - ICSECHAPTER - PROPAGATION OF SOUND WAVES EXERCISE - EXERCISE -8(B) (Multiple choice type)
3 Videos
Similar Questions
A water pump raises 50 litres of water through a height of 25 m in 5 .Calculate the power of the pump required . (Take g=10 N kg−1 and density of water =1000 kg m−3)
View SolutionAn air bubble rises from the bottom of a lake of depth 10.34m to its surface. Compare the pressure on bubble at the bottom to that on surface. (Atmospheric pressure =0.76mofHg, density of Hg=13.6×103Kgm−3 and density of water =103kgm−3).
View SolutionWater is filled in a flask up to a height of 20 cm. The bottom of the flask is circular with radius 10 cm. If the atmospheric pressure is 1.01×105 Pa, find the force exerted by the water on the bottom. Take g=10ms−2 and density of water =1000 kgm^-3.
View SolutionAn iron casting containing a number of cavities weight 6000N in air and 4000N in water. What is the volume of the cavities in the casting? Density of iron is 7.87g/cm3. Take g=9.8m/s2 and density of water =103kg/m3.
View SolutionAn iron casting containing a number of cavities weight 6000N in air and 4000N in water. What is the volume of the cavities in the casting? Density of iron is 7.87g/cm3. Take g=9.8m/s2 and density of water =103kg/m3.
View SolutionAt what depth below the surface of water will pressure be equal to twice the atmospheric pressure ? The atmospheric pressure is 10Ncm−2 , density of water is 103kgm−3 and g=9.8ms−2.
View SolutionA metallic sphere of radius 1.0×10−3m and density 1.0×104kg/m3 enters a tank of water, after a free fall through a distance of h in the earth's gravitational field. If its velocity remains unchanged after entering water, determine the value of h. Given: coefficient of viscosity of water =1.0×10−3Ns/m2,g=10ms−12 and density of water =1.0×103kg/m3.
View SolutionThe U-tube acts as a water siphon. The bend in the tube is 1m above the water surface. The tube outlet is 7m below the water surface. The water issues from the bottom of the siphon as a free jet at atmospheric pressure. Determine the speed of the free jet and the minimum absolute pressure of the water in the bend. Given atmospheric pressure =1.01×105N/m2,g=9.8m/s2 and density of water =103kg/m3
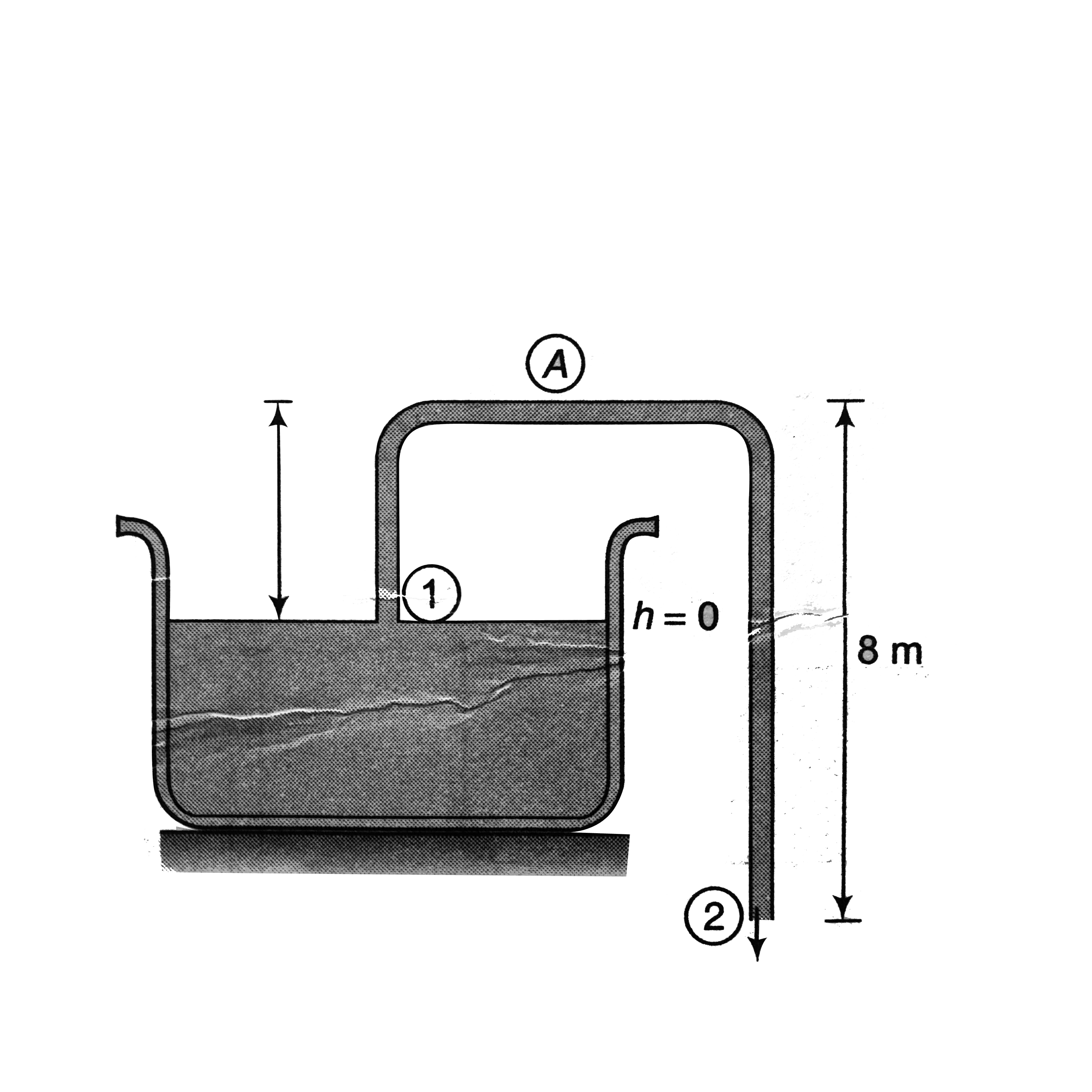 .
.
View SolutionCalculate the height to which water will rise in a capillary tube of diameter 1×10−3m [given surface tension of water is 0.072Nm−1 angle of contact is 0∘,g=9.8ms−2 and density of water =1000kgm−3]
View SolutionThe density of water is ........ kg/m3.
View Solution
