A cell develops the same power across two resistances R1 and R2 separately. The internal resistance of the cell is
To find the internal resistance of a cell that develops the same power across two resistances R1 and R2, we can follow these steps:
Step 1: Understand the Power Formula
The power P across a resistor R connected to a cell with electromotive force ϵ and internal resistance r can be expressed as:
P=I2R
where I is the current flowing through the resistor.
Step 2: Express Current in Terms of Resistance
The current I flowing through the resistor R can be calculated using Ohm's law:
I=ϵR+r
Thus, the power across R1 can be written as:
P1=I2R1=(ϵR1+r)2R1
Similarly, for resistance R2:
P2=I2R2=(ϵR2+r)2R2
Step 3: Set the Powers Equal
Since it is given that the power across both resistances is the same, we can set P1=P2:
(ϵR1+r)2R1=(ϵR2+r)2R2
Step 4: Simplify the Equation
Cancel ϵ2 from both sides:
R1(R1+r)2=R2(R2+r)2
Step 5: Cross Multiply
Cross multiplying gives us:
R1(R2+r)2=R2(R1+r)2
Step 6: Expand Both Sides
Expanding both sides:
R1(R22+2R2r+r2)=R2(R21+2R1r+r2)
Step 7: Rearranging Terms
Rearranging the equation leads to:
R1R22+2R1R2r+R1r2=R2R21+2R2R1r+R2r2
Step 8: Collect Like Terms
Collecting like terms gives:
R1R22−R2R21+(2R1R2−2R2R1)r+(R1−R2)r2=0
This simplifies to:
(R1−R2)(R1+R2)+(R1−R2)r2=0
Step 9: Factor Out Common Terms
Factoring out (R1−R2):
(R1−R2)(R1+R2+r2)=0
Step 10: Solve for Internal Resistance
Since R1≠R2, we can conclude:
R1+R2+r2=0
Thus, solving for r:
r=√R1R2
Final Answer
The internal resistance of the cell is:
r=√R1R2
The power P across a resistor R connected to a cell with electromotive force ϵ and internal resistance r can be expressed as:
P=I2R
where I is the current flowing through the resistor.
The current I flowing through the resistor R can be calculated using Ohm's law:
I=ϵR+r
Thus, the power across R1 can be written as:
P1=I2R1=(ϵR1+r)2R1
P2=I2R2=(ϵR2+r)2R2
Since it is given that the power across both resistances is the same, we can set P1=P2:
(ϵR1+r)2R1=(ϵR2+r)2R2
Cancel ϵ2 from both sides:
R1(R1+r)2=R2(R2+r)2
Cross multiplying gives us:
R1(R2+r)2=R2(R1+r)2
Expanding both sides:
R1(R22+2R2r+r2)=R2(R21+2R1r+r2)
Rearranging the equation leads to:
R1R22+2R1R2r+R1r2=R2R21+2R2R1r+R2r2
Collecting like terms gives:
R1R22−R2R21+(2R1R2−2R2R1)r+(R1−R2)r2=0
This simplifies to:
(R1−R2)(R1+R2)+(R1−R2)r2=0
Factoring out (R1−R2):
(R1−R2)(R1+R2+r2)=0
Since R1≠R2, we can conclude:
R1+R2+r2=0
Thus, solving for r:
r=√R1R2
The internal resistance of the cell is:
r=√R1R2
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Try Yourself
34 Videos
- CURRENT ELECTRICITYBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - ASSIGNMENT(SECTION-A(OBJECTIVE TYPE QUESTIONS))
69 Videos
- COMMUNICATION SYSTEMS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - COMMUNICATION SYSTEMS EXERCISE - ASSIGNMENT SECTION D (Assertion-Reason)
10 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - ASSIGNMENT (SECTION-D)
10 Videos
Similar Questions
A cell supplies a current i1 trhough a resistnace R1 and a current i2 through a resistance R2. The internal resistance of this cell is
View SolutionN identical cells whether joined together in series or in parallel, give the same current, when connected to an external resistance of 'R'. The internal resistance of each cell is:-
View SolutionWhen two identical cells are connected either in series or in parallel across a 4 ohm resistor, they send the same current through it. The internal resistance of the cell in ohm is
View SolutionA battery delivers equal power individually across 4Ω and 16Ω . Internal resistance of the cell is
View SolutionA cell to emf. E and internal resistance r supplies currents for the same time t through external resistanceR1andR2 respectively. If the heat produced in both cases is the same, then the internal resistance is given by :
View SolutionWhen a celll is connected to an external resistance R1, current through it is i1. When the same cell is connected to an external resistance R2, current through it is i2. Calculate the emf and internal resisance of the cell.
View SolutionPower transfer by a non-ideal cell to an external resistor can be same for two different values. Out of which on value (K1) is less than internal resistance of cell and another (R2) is greater than internal resistance of cell then:
View SolutionTwo cells of the same e.mf. e but different internal resistances r1andr2 are connected in series with an external resistance 'R'. The potential drop across the first cell is found to be zero. The external resistance Ris
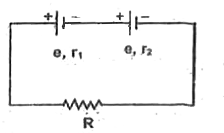
View SolutionA cell sends a current through a resistance R1 for time t, next the same cell sends current through another resistance R2 for the time t If the same amount of heat is developed in both the resistance then find the internal resistance of the cell
View SolutionTwo cells of same emf E, but different internal resistances r1andr2 are connected to an external
resistance R as shown in the figure given above. The voltmeter V reads zero. Obtain an expression for R in terms of r1andr2. Calculate the voltage across the cell of internal resistance r2. (Assume that the voltmeter V is of infinite resistance).
View Solution
