For a diatomic gas having 3 translational and 2 rotational degree of freedom ,the energy is given by ?
To find the energy associated with a diatomic gas having 3 translational and 2 rotational degrees of freedom, we can follow these steps:
1. Identify the Degrees of Freedom:
- For a diatomic gas, there are 3 translational degrees of freedom (movement in x, y, and z directions).
- There are also 2 rotational degrees of freedom (rotation about two axes perpendicular to the molecular axis).
2. Total Degrees of Freedom:
- The total degrees of freedom (dof) can be calculated by adding the translational and rotational degrees of freedom:
Total dof=Translational dof+Rotational dof=3+2=5
3. Energy per Degree of Freedom:
- The energy associated with each degree of freedom at temperature T is given by:
E=12kBT
where kB is the Boltzmann constant.
4. Total Energy Calculation:
- The total energy Etotal for all degrees of freedom is:
Etotal=Total dof×(12kBT)
- Substituting the total degrees of freedom:
Etotal=5×(12kBT)=52kBT
5. Final Result:
- Therefore, the energy associated with the diatomic gas is:
Etotal=52kBT
- For a diatomic gas, there are 3 translational degrees of freedom (movement in x, y, and z directions).
- There are also 2 rotational degrees of freedom (rotation about two axes perpendicular to the molecular axis).
- The total degrees of freedom (dof) can be calculated by adding the translational and rotational degrees of freedom:
Total dof=Translational dof+Rotational dof=3+2=5
- The energy associated with each degree of freedom at temperature T is given by:
E=12kBT
where kB is the Boltzmann constant.
- The total energy Etotal for all degrees of freedom is:
Etotal=Total dof×(12kBT)
- Substituting the total degrees of freedom:
Etotal=5×(12kBT)=52kBT
- Therefore, the energy associated with the diatomic gas is:
Etotal=52kBT
Topper's Solved these Questions
- OSCILLATIONSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - OSCILLATIONSEXERCISE - EXAMPLE
21 Videos
- OSCILLATIONSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - OSCILLATIONSEXERCISE - Exercise
18 Videos
- OSCILLATIONSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - OSCILLATIONSEXERCISE - ASSIGNMENT ( SECTION-I(SUBJECTIVE TYPE QUESTIONS) )
7 Videos
- NUCLEIBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - NUCLEIEXERCISE - ASSIGNMENT (SECTION-D)
10 Videos
- PHYSICAL WORLDBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PHYSICAL WORLDEXERCISE - ASSIGNMENT (Section-B)
4 Videos
Similar Questions
A gas have 3 translation and 2 Rotational degree of freedom. Find CpCv?
View SolutionStatement-1: A reas gas nearly behaves like an ideal gas at low pressure and high temperature.
Statement-2: If the ratio of translational and rotational degree of freedom is 1.5 the gas must be diatomic
Statement-3: Most probable speed of a gas is proportional to absolute temperature of the gas.
View SolutionMolecules of an ideal gas are known to have three translational degrees of freedom and two rotational degrees of freedom . The gas is maintained at a temperature of T. The total internal energy , U of a mole of this gas, and the value of γ(=CPCv) are given, respectively , by :
View SolutionAt ordinary temperatures, the molecules of an ideal gas have only translational and rotational kinetic energies. At high temperatures they may also have vibrational energy.
As a result of this, at higher temperature
View SolutionAt ordinary temperatures, the molecules of an ideal gas have only translational and rotational kinetic energies. At high temperatures they may also have vibrational energy.
As a result of this, at higher temperature
View SolutionThe total energy of molecules is divided equally amongst the various degrees of freedom of a molecule. The distribution of kinetic energy along x, y, z axis are EKx,EKy,EKz
Total K.e =EKx+EKy+EKz
Since the motion of molecule is equally probable in all the three directions, therefore
EKx=EKy=EKz=13EK=13×32kT=12kT, where k=RNA = Botzman constant.
K.E.=12kT per molecule or =12RT per mole.
In vibration motion, molecules possess both kinetic energy as well as potential energy. This means energy of vibration involves two degrees of fiuedom.
Vibration energy =2×12kT=2×12RT [∴ two degrees of freedom per mole]
If the gas molecules have n1 translational degrees of freedom, n2 rotational degrees of freedom and n3 vibrational degrees of freedom, that total energy = n1[kT2]+n2[kT2]+n3[kT2]×2
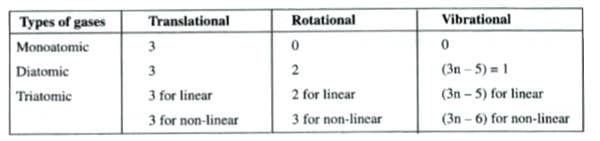
Where 'n' is atomicity of gas.
How many total degrees of freedom are present in H2 molecules in all types of motions ?
View SolutionThe total energy of molecules is divided equally amongst the various degrees of freedom of a molecule. The distribution of kinetic energy along x, y, z axis are EKx,EKy,EKz
Total K.e =EKx+EKy+EKz
Since the motion of molecule is equally probable in all the three directions, therefore
EKx=EKy=EKz=13EK=13×32kT=12kT, where k=RNA = Botzman constant.
K.E.=12kT per molecule or =12RT per mole.
In vibration motion, molecules possess both kinetic energy as well as potential energy. This means energy of vibration involves two degrees of fiuedom.
Vibration energy =2×12kT=2×12RT [∴ two degrees of freedom per mole]
If the gas molecules have n1 translational degrees of freedom, n2 rotational degrees of freedom and n3 vibrational degrees of freedom, that total energy = n1[kT2]+n2[kT2]+n3[kT2]×2
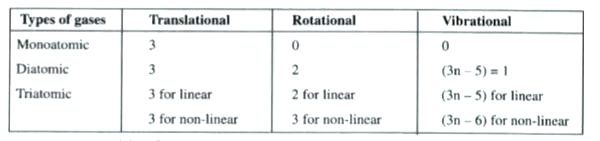
Where 'n' is atomicity of gas.
The rotational kinetic energy of H20 molecule is equal to
View SolutionThe total energy of molecules is divided equally amongst the various degrees of freedom of a molecule. The distribution of kinetic energy along x, y, z axis are EKx,EKy,EKz
Total K.e =EKx+EKy+EKz
Since the motion of molecule is equally probable in all the three directions, therefore
EKx=EKy=EKz=13EK=13×32kT=12kT, where k=RNA = Botzman constant.
K.E.=12kT per molecule or =12RT per mole.
In vibration motion, molecules possess both kinetic energy as well as potential energy. This means energy of vibration involves two degrees of fiuedom.
Vibration energy =2×12kT=2×12RT [∴ two degrees of freedom per mole]
If the gas molecules have n1 translational degrees of freedom, n2 rotational degrees of freedom and n3 vibrational degrees of freedom, that total energy = n1[kT2]+n2[kT2]+n3[kT2]×2
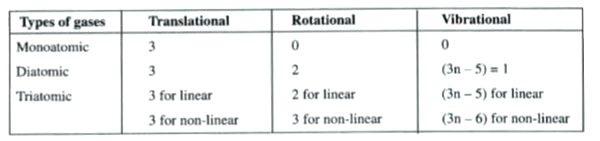
Where 'n' is atomicity of gas.
The vibrational kinetic energy of CO2 molecule is
View SolutionAt ordinary temperatures, the molecules of an ideal gas have only translational and rotational kinetic energies. At high temperatures, they may also have vibrational energy. As a result of this, at higher temperatures, molar specific heat capacity at constant volume, CV is
View SolutionA polyatomic gas with (n) degress of freedom has a mean energy per molecule given by.
View Solution
