Does represent periodic motion ? Ifyes, then find the period is any positive constant )
Hint (Think , Does it repeat itself)
To determine whether the function eωt represents periodic motion, we need to analyze its behavior over time.
Step 1: Understanding the Function
The function given is y=eωt, where ω is a positive constant.
Step 2: Behavior of the Function
As time t increases, the value of eωt also increases. Specifically, since ω is positive, the exponential function grows without bound as t approaches infinity.
Step 3: Definition of Periodic Motion
A function is considered periodic if it repeats its values at regular intervals. Mathematically, a function f(t) is periodic with period T if:
f(t+T)=f(t)
for all t.
Step 4: Checking for Periodicity
Let's check if eωt is periodic by assuming there exists a period T:
eω(t+T)=eωt
This simplifies to:
eωt⋅eωT=eωt
For this equality to hold for all t, it must be that:
eωT=1
The only way this can happen is if ωT=0. Since ω is a positive constant, the only solution is T=0.
Step 5: Conclusion
Since there is no positive period T for which eωt repeats its values, we conclude that eωt does not represent periodic motion.
Final Answer
No, eωt does not represent periodic motion.
---
The function given is y=eωt, where ω is a positive constant.
As time t increases, the value of eωt also increases. Specifically, since ω is positive, the exponential function grows without bound as t approaches infinity.
A function is considered periodic if it repeats its values at regular intervals. Mathematically, a function f(t) is periodic with period T if:
f(t+T)=f(t)
for all t.
Let's check if eωt is periodic by assuming there exists a period T:
eω(t+T)=eωt
This simplifies to:
eωt⋅eωT=eωt
For this equality to hold for all t, it must be that:
eωT=1
The only way this can happen is if ωT=0. Since ω is a positive constant, the only solution is T=0.
Since there is no positive period T for which eωt repeats its values, we conclude that eωt does not represent periodic motion.
No, eωt does not represent periodic motion.
Topper's Solved these Questions
- OSCILLATIONSBook:AAKASH INSTITUTE ENGLISHChapter:OSCILLATIONSExercise:ASSIGNMENT ( SECTION -A)
Explore 58 Videos
- OSCILLATIONSBook:AAKASH INSTITUTE ENGLISHChapter:OSCILLATIONSExercise:ASSIGNMENT (SECTION-B )
Explore 25 Videos
- OSCILLATIONSBook:AAKASH INSTITUTE ENGLISHChapter:OSCILLATIONSExercise:Assignment (Section D) (ASSERTION-REASON TYPE QUESTIONS)
Explore 13 Videos
- NUCLEIBook:AAKASH INSTITUTE ENGLISHChapter:NUCLEIExercise:ASSIGNMENT (SECTION-D)
Explore 10 Videos
- PHYSICAL WORLDBook:AAKASH INSTITUTE ENGLISHChapter:PHYSICAL WORLDExercise:ASSIGNMENT (Section-B)
Explore 4 Videos
Similar Questions
Does cosωt+sin2ωt+cos4ωt represent periodic motion ? If yes, then find the period(ω is any positive constant) .
Hint : ( Each term represents a periodic function with different angular freuency . Find the smallest interval of time after which the sum of three terms repeats ) .
View SolutionWhich of the following functions of time represented (a) periodic and (b) non-periodic motion ? Given the period for each case of periodic motion (ω is any positive constant(i)sinωt−cosωt(ii)log(2ω)
View SolutionWhich of the following functions of time represent (a) simple harmonic (b) periodic but not simple harmonic and (c ) non periodic motion? Give period for each case of periodic motion (ω is nay positive constant)
(1) Sin3ωt (2) 3cos(π/4−2ωt)
(3) cosωt+cos3ωt+cos5ωt
(4) e−ω2t2 (5) 1+ωt+ω2t2
View SolutionCategorise the following function of time eω2t as
(a) S.H.M.
(b) Periodic but not S.H.M. Also , give period if it is periodic motion.
Hint : Doest it repeat itself ?
View SolutionConsider the section of the periodic table given below.
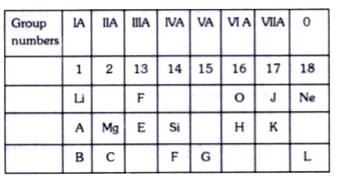
Note : In this table B does not represent boron
C does not represent carbon
F does not represent fluorine
H does not represent hydrogen
K does not represent potassium
You must see the position of the element in the periodic table.
Some elements are given in their own symbol and position in the periodic table, while others are shown with a letter. With reference to the table:
How many valence electrons are present in G?
View SolutionConsider the section of the periodic table given below.
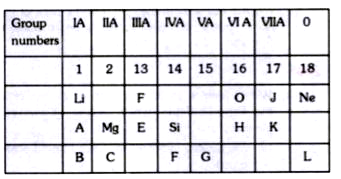
Note : In this table B does not represent boron
C does not represent carbon
F does not represent fluorine
H does not represent hydrogen
K does not represent potassium
You must see the position of the element in the periodic table.
Some elements are given in their own symbol and position in the periodic table, while others are shown with a letter. With reference to the table:
Draw the electron dot structure for the compound formed between C and K.
View SolutionConsider the section of the periodic table given below.
Note: In this table, B does not represent boron
C does not represent carbon
F does not represent fluorine
H does not represent hydrogen
K does not represent potassium
You must see the position of the element in the periodic table.
Some elements are given in their own symbol and position in the periodic table, while others are shown with a letter. With reference to the table:
In the compound between F and J, what type of bond will be formed?
View SolutionConsider the section of the periodic table given below.
Note: In this table, B does not represent boron
C does not represent carbon
F does not represent fluorine
H does not represent hydrogen
K does not represent potassium
You must see the position of the element in the periodic table.
Some elements are given in their own symbol and position in the periodic table, while others are shown with a letter. With reference to the table:
Write the formula of the compound between B and H.
View SolutionConsider the section of the periodic table given below.
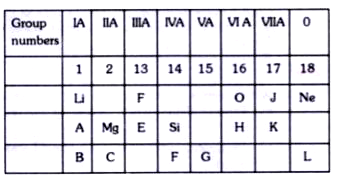
Note : In this table B does not represent boron
C does not represent carbon
F does not represent fluorine
H does not represent hydrogen
K does not represent potassium
You must see the position of the element in the periodic table.
Some elements are given in their own symbol and position in the periodic table, while others are shown with a letter. With reference to the table:
In the compound between F and J, what type of bond will be formed ?
View SolutionConsider the section of the periodic table given below.
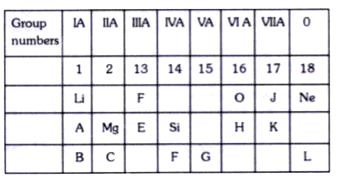
Note : In this table B does not represent boron
C does not represent carbon
F does not represent fluorine
H does not represent hydrogen
K does not represent potassium
You must see the position of the element in the periodic table.
Some elements are given in their own symbol and position in the periodic table, while others are shown with a letter. With reference to the table:
Write the formula of the compound between B and H.
View Solution