Plot the corresponding reference circle for given SHM x=2cos(π3−t) .Indicate the initial (t−0) position of the particle , the radius of the circle and the angular speed of the rotating particle . Consider anticlockwise direction for rotation.
Hint : x=2cos(π3−t)=2cos(t−π3) as cos(−θ)=cosθ . Compare with x=Acos(ωt+ϕ)
x=2cos(π3−t)
=2cos(t−π3) as cos(−θ)=cosθ
Compare with x=Acos(ωt+ϕt)
A=2,ω=1,ϕt=−π3
The reference circle is as shown in figure

Topper's Solved these Questions
- OSCILLATIONSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - OSCILLATIONSEXERCISE - ASSIGNMENT ( SECTION -A)
58 Videos
- OSCILLATIONSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - OSCILLATIONSEXERCISE - ASSIGNMENT (SECTION-B )
25 Videos
- OSCILLATIONSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - OSCILLATIONSEXERCISE - Assignment (Section D) (ASSERTION-REASON TYPE QUESTIONS)
13 Videos
- NUCLEIBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - NUCLEIEXERCISE - ASSIGNMENT (SECTION-D)
10 Videos
- PHYSICAL WORLDBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PHYSICAL WORLDEXERCISE - ASSIGNMENT (Section-B)
4 Videos
Similar Questions
Plot the corresponding reference circle for given SHM, indicate the initial (t=0) position of the paritcle, the radius of the circle , and the angular speed of the rotating paritcle. Consider anticlockwise direction for rotation. x=−3sin(2t+π4) (Express in the form , x= Acos ( omega t + phi)).
View SolutionThe corresponding reference circle for a given SHM has been shown in the figure. Given π=7π4 . Write the corresponding equation of SHM. Consider anticlockwise direction for rotation.
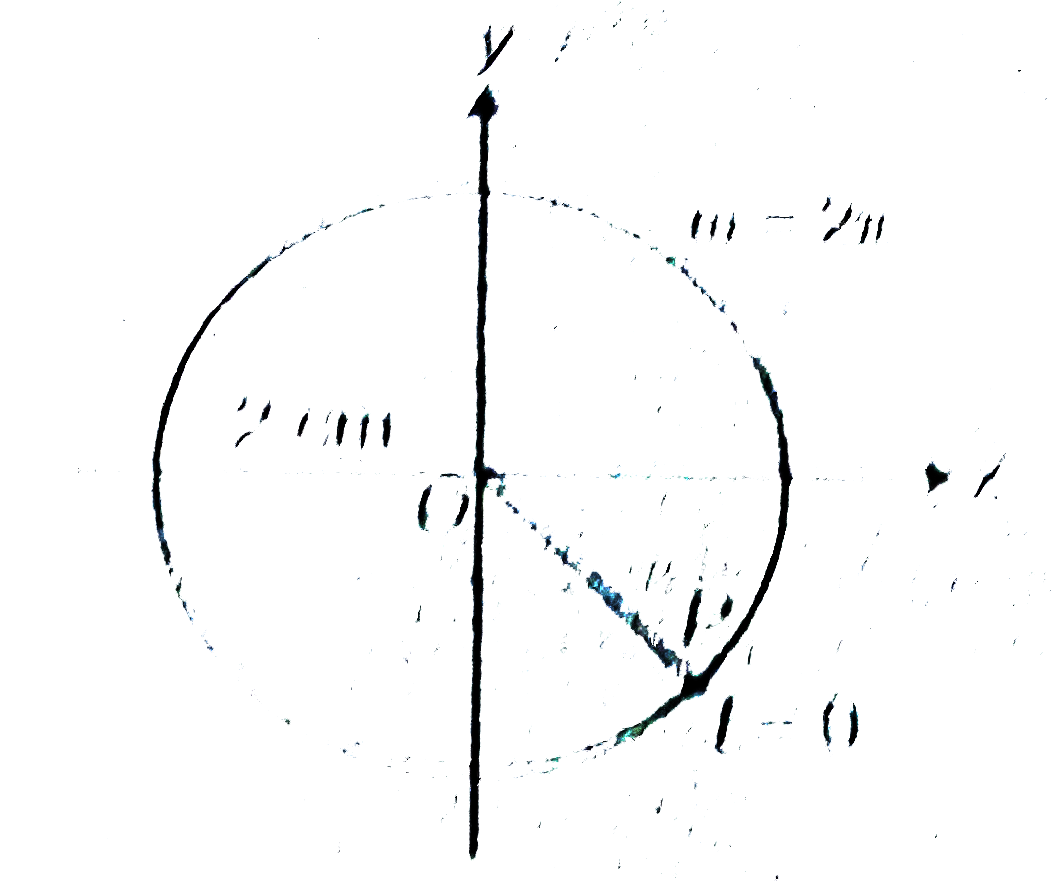
Hint :x=2cos(2πt+7π4)
2cos(3π2+(2πt+π4))
View SolutionThe corresponding reference circle for a given SHM has been shown in the figure. Given ϕ=7π4 . Write the corresponding equation of SHM. Consider anticlockwise direction for rotation.

Hint :x=2cos(2πt+7π4)
2cos(3π2+(2πt+π4))
View SolutionIf tan(π2sinθ)=cot(π2cosθ), then sinθ+cosθ is equal to
View SolutionProve that cosθ+cos(120∘+θ)+cos(120∘−θ)=0.
Hence deduce that cos3θ+cos3(120∘+θ)+cos3(120∘−θ)=34cos3θ.
View SolutionSolve cosθ⋅cos2θ⋅cos3θ=14,0≤θ≤π
View SolutionIf sin(πcosθ)=cos(πsinθ), then the value of cos(θ±π4) is
View SolutionProve that cos2x+cos2(x+π3)+cos2(x−π3)=32.
View SolutionProve that: 4 cosθcos (π3+θ)cos(π3−θ)=cos3θ
View SolutionProve that cos(π+θ)cos(−θ)cos(π−θ)cos(π2+θ)=−cotθ
View Solution
