The displacement s of an object is given as a function of time t by the following equation s=2t+5t2+3t3. Calculate the instantaneous velocity of the object at t = 1 s.
The correct Answer is:21 m/s.
To find the instantaneous velocity of the object at t=1 second given the displacement function s(t)=2t+5t2+3t3, we will follow these steps:
Step 1: Differentiate the displacement function
The instantaneous velocity v(t) is defined as the derivative of the displacement s(t) with respect to time t. We will differentiate the given function:
s(t)=2t+5t2+3t3
Differentiating term by term:
- The derivative of 2t is 2.
- The derivative of 5t2 is 10t.
- The derivative of 3t3 is 9t2.
Thus, the derivative of the displacement function is:
v(t)=dsdt=2+10t+9t2
Step 2: Substitute t=1 into the velocity function
Now, we will substitute t=1 second into the velocity function to find the instantaneous velocity at that moment:
v(1)=2+10(1)+9(1)2
Calculating this step by step:
1. 10(1)=10
2. 9(1)2=9
Now, substituting these values back into the equation:
v(1)=2+10+9=21
Step 3: Conclusion
The instantaneous velocity of the object at t=1 second is:
21 m/s
---
The instantaneous velocity v(t) is defined as the derivative of the displacement s(t) with respect to time t. We will differentiate the given function:
- The derivative of 5t2 is 10t.
- The derivative of 3t3 is 9t2.
Now, we will substitute t=1 second into the velocity function to find the instantaneous velocity at that moment:
2. 9(1)2=9
The instantaneous velocity of the object at t=1 second is:
Topper's Solved these Questions
- MOTION IN STRAIGHT LINEBook:AAKASH INSTITUTE ENGLISHChapter:MOTION IN STRAIGHT LINEExercise:Assignment (SECTION - A)
Explore 50 Videos
- MOTION IN STRAIGHT LINEBook:AAKASH INSTITUTE ENGLISHChapter:MOTION IN STRAIGHT LINEExercise:Assignment (SECTION - B)
Explore 19 Videos
- MOTION IN STRAIGHT LINEBook:AAKASH INSTITUTE ENGLISHChapter:MOTION IN STRAIGHT LINEExercise:Assignment (SECTION - J)
Explore 2 Videos
- MOTION IN A STRAIGHT LINEBook:AAKASH INSTITUTE ENGLISHChapter:MOTION IN A STRAIGHT LINEExercise:ASSIGNMENT (SECTION - D)
Explore 15 Videos
- MOVING CHARGE AND MAGNESIUMBook:AAKASH INSTITUTE ENGLISHChapter:MOVING CHARGE AND MAGNESIUMExercise:SECTION D
Explore 16 Videos
Similar Questions
The displacement s of an object is given as a function of time t
s=5t2+9t
Calculate the instantaneous velocity of object at t = 0.
View SolutionThe displacement x of an object is given as a function of time x=2t+3t2
Calculate the instantaneous velocity of the object at t = 2 s
View SolutionThe velocity of an object is changing with time and relation is given by the following equation.
v=2t+3t2
Calculate the position of the object from the origin at t = 2 s.
Assume particle to be at origin at t = 0
View SolutionThe displacement x of an object is given as a funstion of time, x=2t+3t2. The instantaneous velocity of the object at t = 2 s is
View SolutionThe displacement (in metre) of a particle moving along x-axis is given by x=18t+5t2. Calculate (i) the instantaneous velocity t=2s (ii) average velocity between t=2s to t=3s (iii) instantaneous acceleration.
View SolutionA particle starts from origin with uniform acceleration. Its displacement after t seconds is given in meter by the relation x=2+5t+7t2. Calculate the magnitude of its
a. Initial velocity
b. Velocity at t=4s
c. Uniform acceleration
d. Displacement at t=5s
View SolutionThe velocity of an object moving rectilinearly is given as a function of time by v=4t−3t2 where v is in m/s and t is in seconds. The average velocity if particle between t=0 to t=2 seconds is
View SolutionA particle is moving on a straight line with velocity (v) as a function of time (t) according to relation v=(5t2−3t+2)m/s . Now give the answer of following questions :
Velocity of particle at t = 3 sec. is :
View SolutionIn the s−t equations (s=10+20t−5t2) match the following
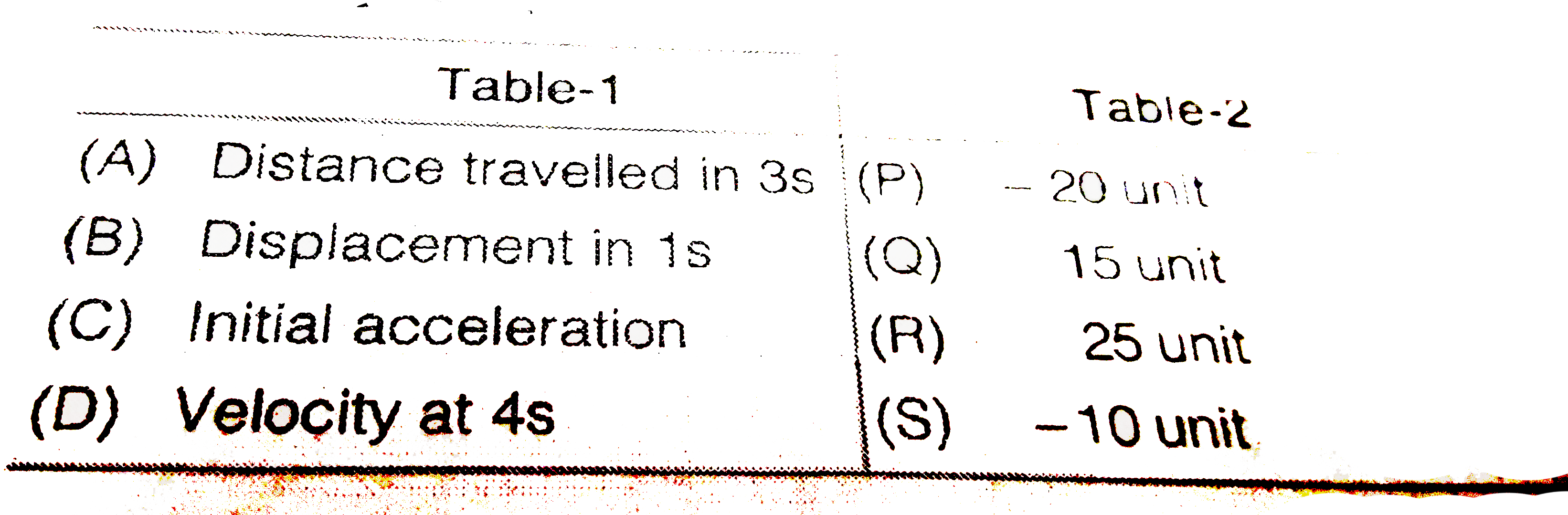
View SolutionThe displacement of an object of mass 3 kg is given by the relation S=13t2, where t is time in seconds. If the work done by the net force on the object in 2 s is pq joule, where p and q are smallest integer values, then what is the value of p+q?
View Solution