Following results are observed when sodium metal is irradiated with different wavelength. Calculate (a) threshold wavelength and (b) Planck's constant
λ(nm)500450400v×10−5(cms−1)2.554.355.35
To solve the problem, we will follow these steps:
Step 1: Understand the Given Data
We have the following data for sodium metal when irradiated with different wavelengths:
- Wavelengths (in nm): λ1=500, λ2=450, λ3=400
- Velocities (in cm/s×10−5): v1=2.55, v2=4.35, v3=5.35
Step 2: Convert Wavelengths to Meters
We need to convert the wavelengths from nanometers to meters for our calculations:
- λ1=500nm=500×10−9m
- λ2=450nm=450×10−9m
- λ3=400nm=400×10−9m
Step 3: Use the Photoelectric Equation
The photoelectric equation relates the energy of the incident photons to the kinetic energy of the emitted electrons:
Ek=hν−hν0
Where:
- Ek is the kinetic energy of the emitted electrons.
- h is Planck's constant.
- ν is the frequency of the incident light.
- ν0 is the threshold frequency.
We can express frequency in terms of wavelength:
ν=cλ
Where c is the speed of light.
Step 4: Set Up the Equations
Using the above relationships, we can write:
hcλ−hcλ0=12mv2
For each wavelength, we can set up three equations:
1. For λ1=500:
hc500×10−9−hcλ0=12m(2.55×10−5)2
2. For λ2=450:
hc450×10−9−hcλ0=12m(4.35×10−5)2
3. For λ3=400:
hc400×10−9−hcλ0=12m(5.35×10−5)2
Step 5: Solve for Threshold Wavelength λ0
To find λ0, we can eliminate h and c by dividing the equations. For example, divide equation 2 by equation 1:
hc450−hcλ0hc500−hcλ0=12m(4.35×10−5)212m(2.55×10−5)2
This simplifies to:
λ0−450λ0−500=450500⋅(4.352.55)2
Calculating the right side gives us a numerical value. We can then cross-multiply and solve for λ0.
Step 6: Substitute λ0 into One of the Equations to Find h
Once we have λ0, we can substitute it back into one of the original equations (e.g., equation 3) to solve for Planck's constant h.
Step 7: Final Calculation
After substituting and simplifying, we will arrive at the values for λ0 and h.
Final Answers
- Threshold Wavelength λ0≈531nm
- Planck's Constant h≈6.66×10−34J s
We have the following data for sodium metal when irradiated with different wavelengths:
- Wavelengths (in nm): λ1=500, λ2=450, λ3=400
- Velocities (in cm/s×10−5): v1=2.55, v2=4.35, v3=5.35
We need to convert the wavelengths from nanometers to meters for our calculations:
- λ1=500nm=500×10−9m
- λ2=450nm=450×10−9m
- λ3=400nm=400×10−9m
The photoelectric equation relates the energy of the incident photons to the kinetic energy of the emitted electrons:
Ek=hν−hν0
Where:
- Ek is the kinetic energy of the emitted electrons.
- h is Planck's constant.
- ν is the frequency of the incident light.
- ν0 is the threshold frequency.
ν=cλ
Where c is the speed of light.
Using the above relationships, we can write:
hcλ−hcλ0=12mv2
1. For λ1=500:
hc500×10−9−hcλ0=12m(2.55×10−5)2
2. For λ2=450:
hc450×10−9−hcλ0=12m(4.35×10−5)2
3. For λ3=400:
hc400×10−9−hcλ0=12m(5.35×10−5)2
To find λ0, we can eliminate h and c by dividing the equations. For example, divide equation 2 by equation 1:
hc450−hcλ0hc500−hcλ0=12m(4.35×10−5)212m(2.55×10−5)2
λ0−450λ0−500=450500⋅(4.352.55)2
Once we have λ0, we can substitute it back into one of the original equations (e.g., equation 3) to solve for Planck's constant h.
After substituting and simplifying, we will arrive at the values for λ0 and h.
- Threshold Wavelength λ0≈531nm
- Planck's Constant h≈6.66×10−34J s
Topper's Solved these Questions
- STRUCTURE OF ATOMBOOK - ICSECHAPTER - STRUCTURE OF ATOMEXERCISE - Numerical Problems
15 Videos
- STATES OF MATTER : GASES AND LIQUIDSBOOK - ICSECHAPTER - STATES OF MATTER : GASES AND LIQUIDSEXERCISE - NCERT TEXT-BOOK EXERCISES (With Hints and Solutions)
23 Videos
- THE s - BLOCK ELEMENTSBOOK - ICSECHAPTER - THE s - BLOCK ELEMENTSEXERCISE - NCERT TEXT-BOOK. EXERCISES (WITH HINTS AND SOLUTIONS)
55 Videos
Similar Questions
Knowledge Check
Calculate the energy in joule corresponding to light of wavelength 45 nm : (Planck's constant h=6.63×10−34 Js: speed of light :c=3×108ms−1).
A6.67×1015B6.67×1011C4.42×10−15D4.42×10−18
When a metallic surface is illuminated with radiation of wavelength λ, the stopping potential is V. If the same surface is illuminated with radiation of wavelength 2λ , the stopping potential is V4. The threshold wavelength surface is :
(a) 5λ (b) 52λ (c) 3λ (d) 4λ
View SolutionThe work function for tungsten and sodium are 4.5eV and 2.3eV respectively . If the threshold wavelength λ for sodium is 5460Å, the value of λ for tungsten is
View SolutionThe frequency of the strong yellow line in the spectrum of sodium in 5.09×1014s−1 .Calculate the wavelength of the light nanometres
View SolutionIn an experiment on photoelectric effect, the stopping potential is measured for monochromatic light beams corresponding to different wavelength. The data collected are as follows:
wavelength (nm): 350 400 450 500 550
stopping potential (V): 1.45 1.00 0.66 0.38 0.16
Plot the stopping potential against inverse of wavelength on a graph paper and find (a) the Planck constant, (b) the work function of the emitter and (c) the threshold wavelength .
View SolutionIn a historical experiment to determine Planck's constant, a metal surface was irradiated with light of different wavelengths. The emitted photoelectron energies were measured by applying a stopping potential. The relevant data for the wavelength (λ). ) of incident light and the corresponding stopping potential (V0) are given below :
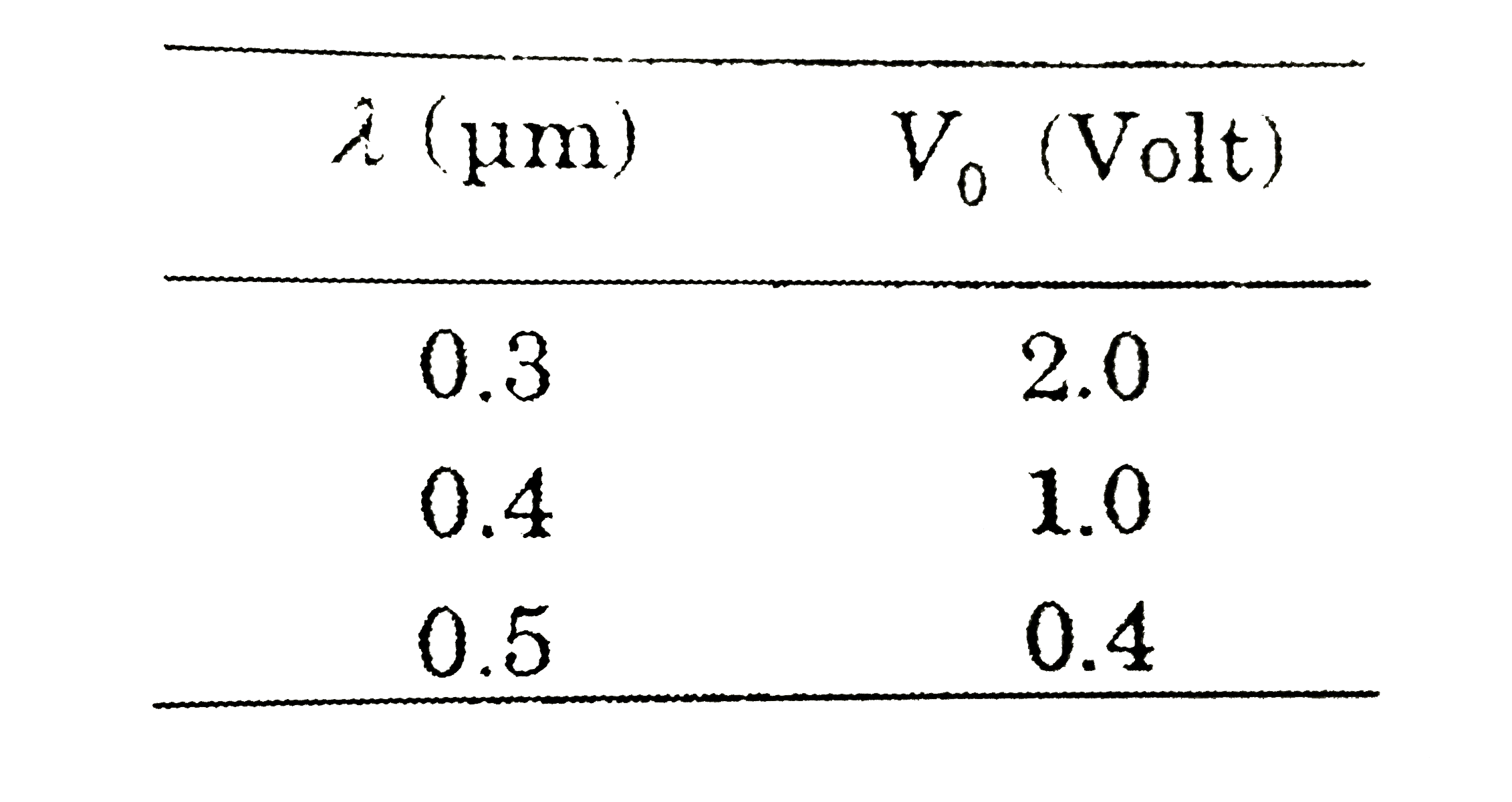
Given that c=3×108ms−1ande=1.6×10−19C Planck's constant (in units of J s) found from such an experiment is
View SolutionA slit 5 cm wide when irradiated by waves of wavelength 10 mm results in the angular spread of the central maxima on either side of incident light by about :
View SolutionThe work function of a metal is in the range of 2 eV to 5 eV. Find which of the following wavelength of light cannot be used for photoelectric effect. (Consider, Planck's constant =4×10−15 eV - s, velocity of light =3×108ms−1)
View Solution
