A body B lies on a smooth horizontal table and another body A is placed on B. The coefficient of friction between A and B is μ. What acceleration given to B will cause slipping to occur between A and B
μg
gμ
μg
√μg
The correct Answer is:A
To solve the problem, we need to analyze the forces acting on both bodies A and B and apply Newton's second law. Here’s a step-by-step solution:
Step 1: Understand the System
- Body A is placed on body B, which is on a smooth horizontal table.
- The coefficient of friction between A and B is μ.
- We need to find the acceleration of body B (denoted as \( a_B \)) that will cause body A to slip on body B.
Step 2: Free Body Diagram for Body B
- For body B, the forces acting on it are:
- A force \( F \) applied in the direction of acceleration.
- A frictional force \( F_f \) acting in the opposite direction due to body A.
- According to Newton's second law, we can write the equation for body B:
\(
F - F_f = m_B a_B
\)
Step 3: Determine the Frictional Force
- The maximum static frictional force \( F_f \) that can act between A and B is given by:
\(
F_f = \mu N_A
\)
where \( N_A \) is the normal force on body A.
- Since body A is resting on body B, the normal force \( N_A \) is equal to the weight of body A:
\(
N_A = m_A g
\)
- Thus, we can express the frictional force as:
\(
F_f = \mu m_A g
\)
Step 4: Substitute into the Equation for Body B
- Substituting \( F_f \) into the equation for body B gives:
\(
F - \mu m_A g = m_B a_B
\)
- Rearranging this equation, we find:
\(
a_B = \frac{F - \mu m_A g}{m_B}
\)
Step 5: Free Body Diagram for Body A
- For body A, the frictional force \( F_f \) is the only force causing it to accelerate. Thus, we can write:
\(
F_f = m_A a_A
\)
- Substituting for \( F_f \) gives:
\(
\mu m_A g = m_A a_A
\)
- Simplifying this, we find:
\(
a_A = \mu g
\)
Step 6: Condition for Slipping
- For slipping to occur, the acceleration of body B must be greater than the acceleration of body A:
\(
a_B > a_A
\)
- Substituting \( a_A \) into this inequality gives:
\(
a_B > \mu g
\)
Step 7: Critical Point of Slipping
- The critical point where slipping just begins is when:
\(
a_B = a_A
\)
- Therefore, at the point of slipping:
\(
a_B = \mu g
\)
Final Answer
- The acceleration given to body B that will cause slipping to occur between A and B is:
\(
a_B = \mu g
\)
- Body A is placed on body B, which is on a smooth horizontal table.
- The coefficient of friction between A and B is μ.
- We need to find the acceleration of body B (denoted as \( a_B \)) that will cause body A to slip on body B.
- For body B, the forces acting on it are:
- A force \( F \) applied in the direction of acceleration.
- A frictional force \( F_f \) acting in the opposite direction due to body A.
- According to Newton's second law, we can write the equation for body B:
\(
F - F_f = m_B a_B
\)
- The maximum static frictional force \( F_f \) that can act between A and B is given by:
\(
F_f = \mu N_A
\)
where \( N_A \) is the normal force on body A.
- Since body A is resting on body B, the normal force \( N_A \) is equal to the weight of body A:
\(
N_A = m_A g
\)
- Thus, we can express the frictional force as:
\(
F_f = \mu m_A g
\)
- Substituting \( F_f \) into the equation for body B gives:
\(
F - \mu m_A g = m_B a_B
\)
- Rearranging this equation, we find:
\(
a_B = \frac{F - \mu m_A g}{m_B}
\)
- For body A, the frictional force \( F_f \) is the only force causing it to accelerate. Thus, we can write:
\(
F_f = m_A a_A
\)
- Substituting for \( F_f \) gives:
\(
\mu m_A g = m_A a_A
\)
- Simplifying this, we find:
\(
a_A = \mu g
\)
- For slipping to occur, the acceleration of body B must be greater than the acceleration of body A:
\(
a_B > a_A
\)
- Substituting \( a_A \) into this inequality gives:
\(
a_B > \mu g
\)
- The critical point where slipping just begins is when:
\(
a_B = a_A
\)
- Therefore, at the point of slipping:
\(
a_B = \mu g
\)
- The acceleration given to body B that will cause slipping to occur between A and B is:
\(
a_B = \mu g
\)
Similar Questions
If the coefficient of friction between A and B is μ, the maximum acceleration of the wedge A for which B will remain at rest with respect to the wedge is

View SolutionIf the coefficient of friction between A and B is μ, the maximum acceleration of the wedge A for which B will remain at rest with respect to the wedge is

View SolutionBlock B rests on a smooth surface .The coefficient of static friction between A and B is μ=0.4 where F=30N then
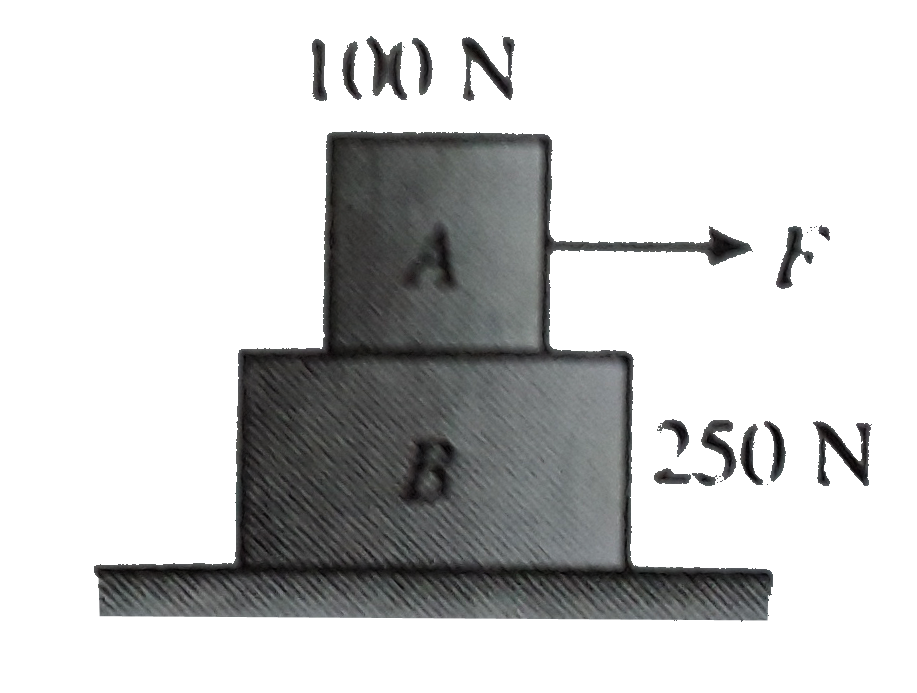
Acceleration of upper block is
View SolutionA block of mass m is stationary on a rough inclined plane making an angle θ with the horizontal. Find the contact force between block and the plane
View SolutionA block A of mass m kg lies on a block B of mass m kg. B in turn lies on smooth horizontal plane. The coefficient of friction between A and B is μ. Both the blocks are initially at rest. A horizontal force F is applied to lower block B at t = 0 till the lower block B undergoes a displacement of magnitude L. Match the statements in Table-1 with the results in Table-2.

View Solution
Two blocks A (1 kg) and B (2 kg) are connected by a string passing over a smooth pulley as shown in the figure. B rests on rough horizontal surface and A rest on B. The coefficient of friction between A and B is the same as that between B and the horizontal surface. The minimum horizontal force F required to move A to the left is 25 N. The coefficient of friction is:
View SolutionTwo block A and B of masses 5 kg. and 3 kg. respectively rest on smooth horizontal surface with B over A. The coefficient of friction between A and B. 0.5 the maximum horizontal force that can be applied to A so that there will be motion of A and B without separation is :-
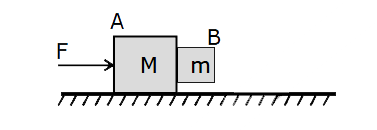
View SolutionCoefficient of friction between A and B is μ . The minimum force F with which A will be pushed such that B will not slip down is -
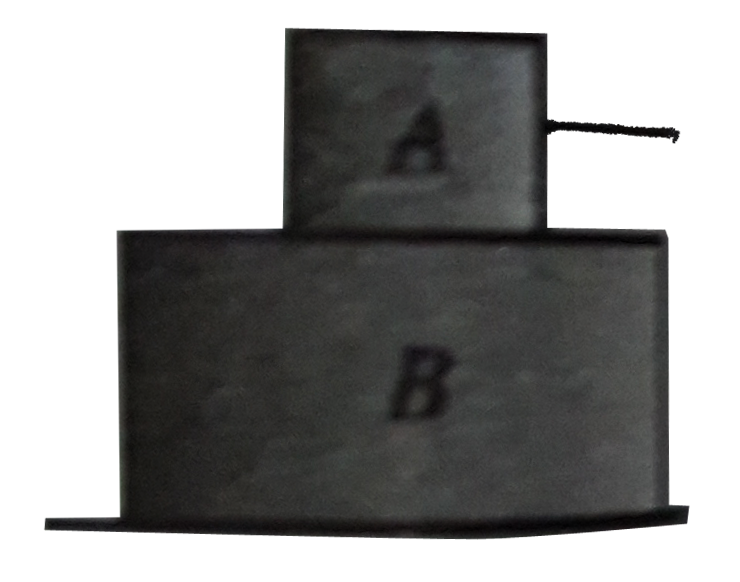
View SolutionA block A of mass 2kg is placed over smooth block B of mass 4kg which is placed over a smooth horizontal floor The coefficient of friction between A and B is 0.4 when a horizontal forces of magnitude 10N is applied on A the acceleration of block of A and B are
View SolutionA horizontal force F acts on the sphere at its centre as shown. Coefficient of friction between ground and sphere is μ. What is maximum value of F for which there is no slipping?
View Solution