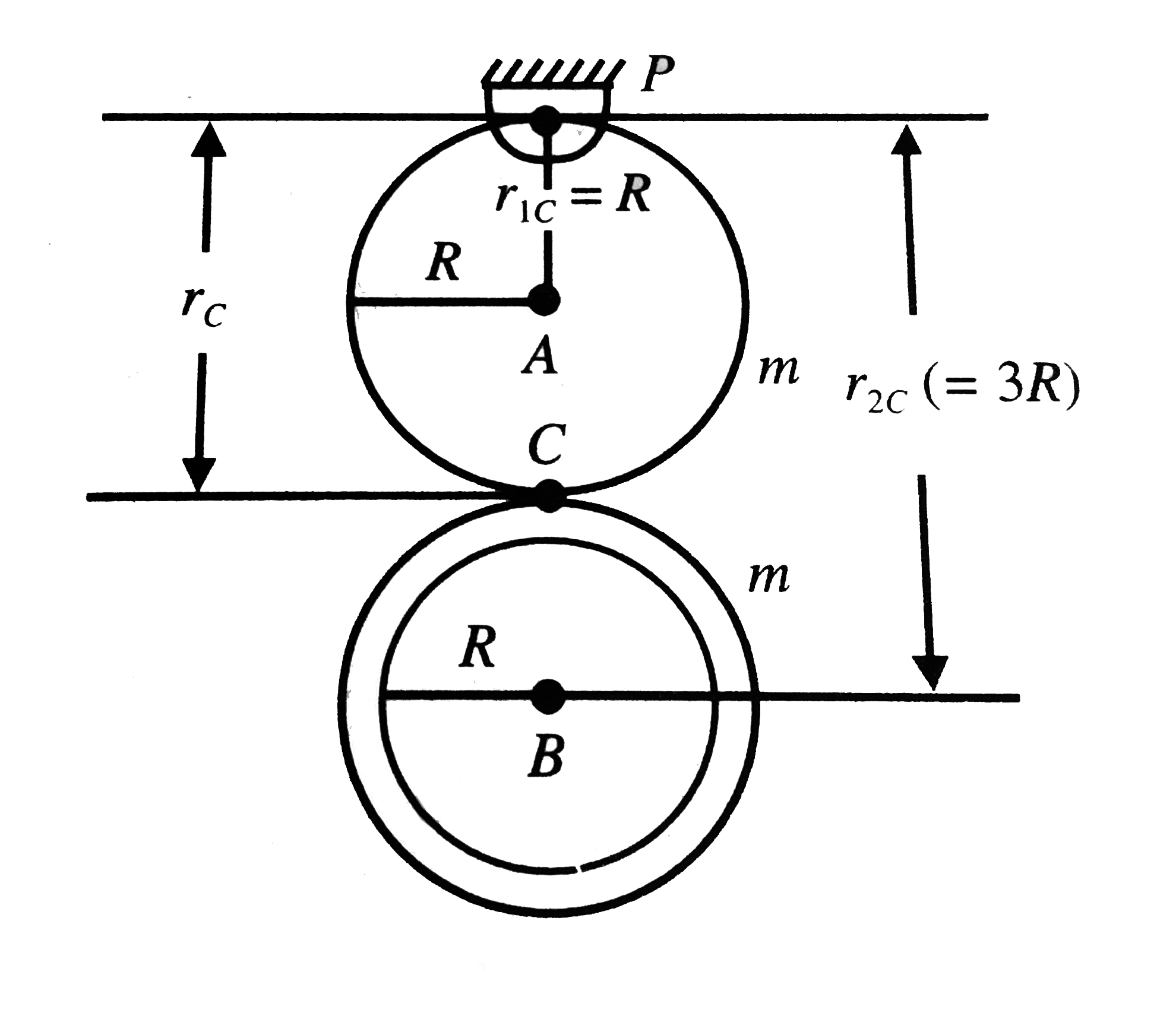
A uniform dics of mass m and radius $R = \frac{80}{23 π^{2}}$ m is pivoted smoothly at $P$ . If a uniform disc of mass $m$ and radius $R$ is welded at the lowest point of the disc, find the period of $S H M$ of the system (disc $+$ ring). (in seconds)

Video Solution

Text Solution

Verified by Experts

The time period of a physical pendulum is
$T = 2 π \sqrt{\frac{I_{P}}{M g r}}$
Here we have three quantities $I_{P}$ , m and r
Let us calculate the quentities one by one as follows: Finding $I_{P} : I_{P} = {(I_{P})}_{d i s c} + {(I_{P})}_{r i n g}$
Where ${(I_{P})}_{d i s c} = I_{A} + m {(P A)}^{2} = \frac{m R^{2}}{2} + m R^{2} = \frac{3 m R^{2}}{2}$
and ${(I_{P})}_{r i n g} = I_{b} {(P B)}^{2} = m R^{2} + m (3 R) = 10 m R^{2}$
Then, we have $I_{P} = \frac{3}{2} m R^{2} = 10 m R^{2} = \frac{23}{2} m R^{2}$
Finding $r$ .
$r = {\to r}_{C} = \frac{m_{1} {\to r}_{1} + m_{2} + {\to r}_{2 C}}{m_{1} + m_{2}}$
where $m_{1} = m$ , $m_{2} = m$
${\to r}_{1 C} = - R$ and $r_{2 C} = - 3 R$
This gives $r_{C} = 2 R$
Finding M:
$M = {(m)}_{d i s c} + {(m)}_{r i n g} = m + m = 2 m$
Substituting $I_{P} = \frac{23}{2} m R^{2}$ , $M = 2 m$ and $r = 2 R$ in the expression
$T = 2 π \sqrt{\frac{I_{P}}{M g r}}$ we have $T = π \sqrt{\frac{23 R}{g}}$
After subsituting the value we get $t = 2 s$ .

|

Updated on:21/07/2023

Class 11 PHYSICS SIMPLE HARMONIC MOTION

Topper's Solved these Questions

Similar Questions

CENGAGE PHYSICS ENGLISH-LINEAR AND ANGULAR SIMPLE HARMONIC MOTION-Integer

Home
Ask Doubt
Profile