
Three rods AB, BC and BD of same length l and cross section A are arranged as shown. The end D is immersed in ice whose mass is 440 g and is at 0∘C. The end C is maintained at 100∘C. Heat is supplied at constant rate of 200 cal/s. Thermal conductivities of AB, BC and BD are K, 2K and K/2, respectively Time after which whole ice will melt is (K=100cal/m−s∘CA=10cm2,l=1cm)
400 s
600 s
700 s
800 s
The correct Answer is:D
Let θ be the temperate of B
2KA(θ−100)l+(K2)A(θ−0)l=200
Substituting values θ=880∘C
Also from B→D,K2A(θ−0)l=4405×80
t=800s
Topper's Solved these Questions
- CALORIMETRYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Multiple Correct
25 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Comprehension
30 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Subjective
25 Videos
- BASIC MATHEMATICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - BASIC MATHEMATICSEXERCISE - Exercise 2.6
20 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - INTEGER_TYPE
1 Videos
Similar Questions
Three rods AB,BC and BD of same length l and cross-sectionsl area A are arranged as shown . The end D is immersed in ice whose mass is 440gm Heat is being supplied at constant rate of 200cal/sec from the end Time in which whole ice will melt (Latent heat of fusion of ice is 80cal/gm
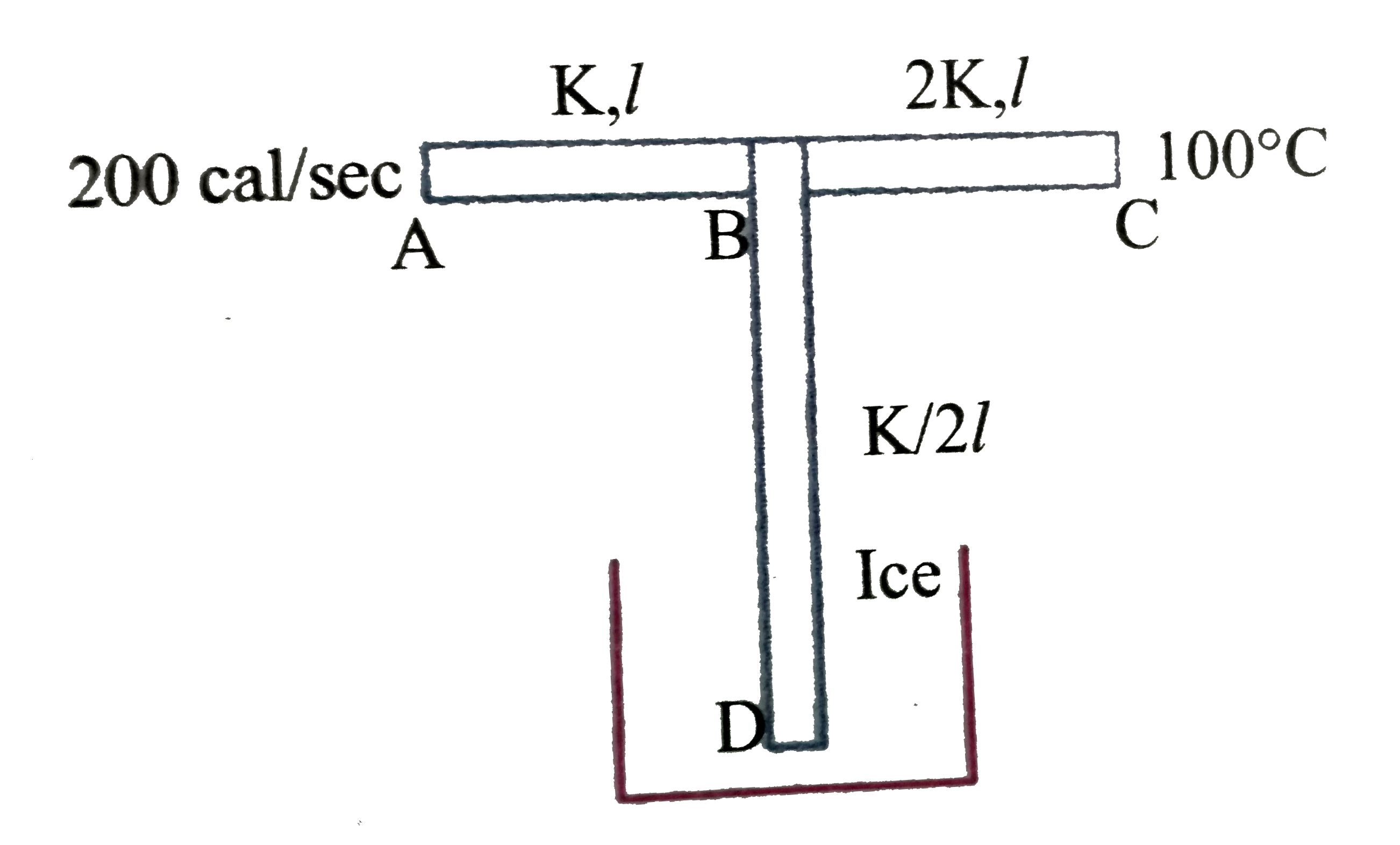 .
.
View SolutionThree rods AB,BC and BD of same length l and cross sectional area A are arranged as shown. The end D is immersed in ice whose mass is 440 gm. Heat is being supplied at constant rate of 200 cal /sec from the end A. Find out time (in sec) in which wholeice will melt. (latent heat of fusion of ice is 80 cal/gm)
Given k (thermal conductivity) =100 cal/m/sec/∘C,A=10cm2,l=1m
View SolutionThree rods A, B and C of the same length and same cross-sectional area are joined as shown. Their thermal conductivities are in the ratio 1:2:3/2. If the open ends of A and C are at 200∘C and 18∘C, respectively, then the temperature at the junction of A at steady state is
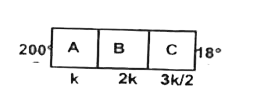
View SolutionThree rods of same dimensions have thermal conductivity 3K,2K and K They are arranged as shown in Then the temperature of the junction in steady state is
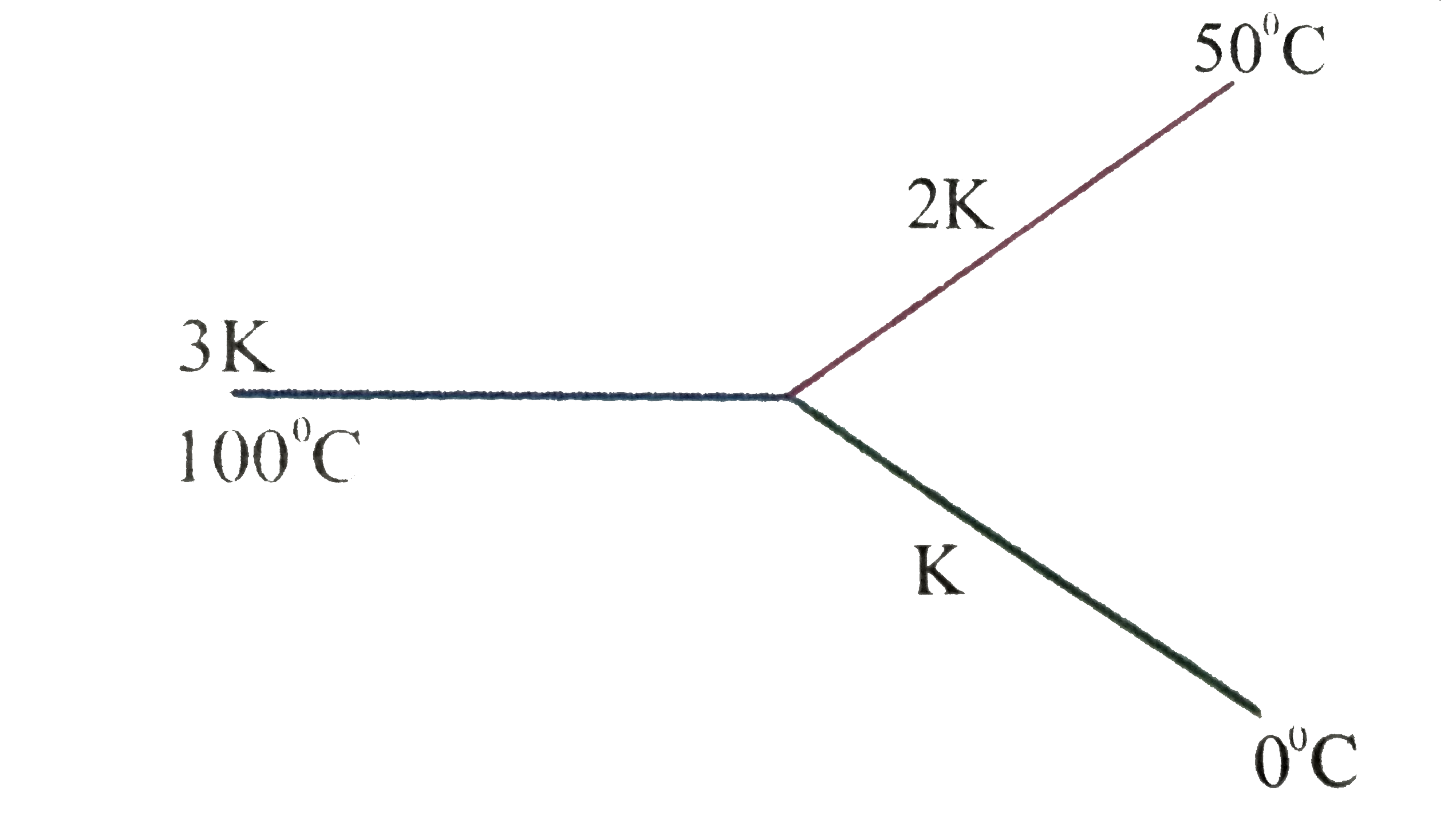 .
.
View SolutionThree rods each of same length and cross - section are joined in series. The thermal conductivity of the materials are K, 2K and 3K respectively. If one end is kept at 200∘C and the other at 100∘C. What would be the temperature of the junctions in the steady state? Assume that no heat is lost due to radiation from the sides of the rods.
View SolutionTwo rods A and B of same area of cross-section are joined together end to end The free end of the rod A is kept in melting ice at 300 K and free end of B rod at 400 K. The rods are of the same length. The conductivity of A is 3 times that of B. Calculate the temperature of the junction of the rods.
View SolutionThree rodsP,Q and R having, samearea of cross section and lengths are connected with each other as shown in figure. The rod through which no heat will flow is (Thermal conductivities of rods P,Q,andR are 2k,4k and 2k respectively)

View SolutionThree conducting rods of same material and cross-section are shown in figure. Temperatures of A,D and C are maintained at 20∘C,90∘Cand0∘C. The ratio of lengths BD and BC if there is no heat flow in AB is
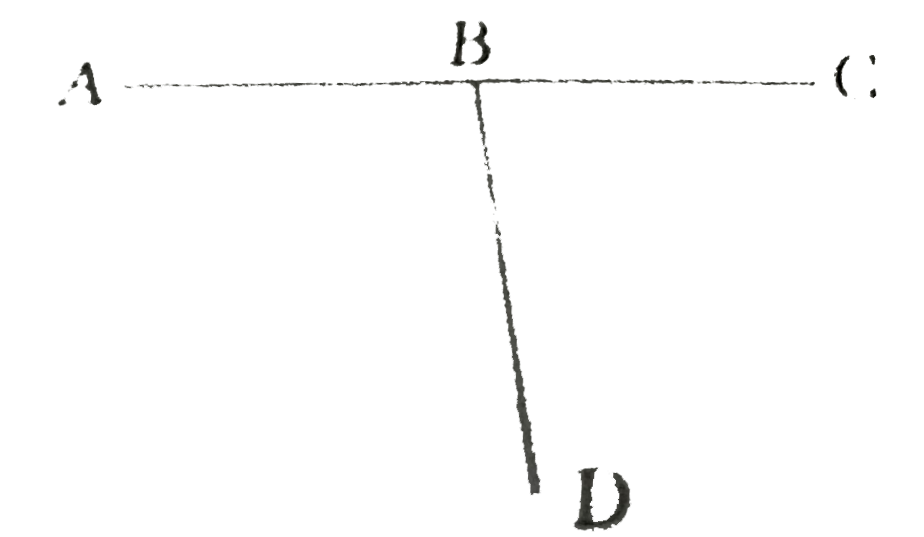
View SolutionTwo rods of similar area of cross section and length are joined as shown in the figure. The ratio of their thermal conductivity is 2:3. The free ends are maintained at 100∘C and 0∘C respectivley. THE temperature at junction in steady state is
View Solution200 g of ice at 0∘C converts into water at 0∘C in 1 minute when heat is supplied to it at a constant rate. In how much time 200 g of water at 0∘C will change to 20∘C ? Take specific latent heat of ice = 336 J g−1.
View Solution
