A +6μC point charge is moving at a constant velocity of 8×106ms−1 in the +y direction, relative to a reference frame. At the instant when the point charge is at the origin of this reference frame, what is the magnetic field vector it produces at the following points?
(a) x=0.500m,y=0,z=0, and (b) x=0,y=−0.500m,z=0.
To solve the problem of finding the magnetic field produced by a moving point charge at specific points in space, we can use the formula for the magnetic field B created by a moving charge:
B=μ04πqv×rr3
where:
- q is the charge,
- v is the velocity of the charge,
- r is the position vector from the charge to the point where we want to calculate the magnetic field,
- r is the magnitude of the position vector,
- μ0 is the permeability of free space (μ0=4π×10−7T m/A).
Given Data:
- Charge, q=+6μC=6×10−6C
- Velocity, v=8×106m/s^j
- Points to calculate B:
- (a) x=0.500m,y=0,z=0
- (b) x=0,y=−0.500m,z=0
1. Position Vector:
r=0.5^i+0^j+0^k=0.5^i
2. Magnitude of r:
r=|r|=0.5m
3. Cross Product v×r:
v=8×106^j
v×r=(8×106^j)×(0.5^i)=8×106×0.5(^j×^i)=4×106(−^k)=−4×106^k
4. Substituting into the Magnetic Field Formula:
B=μ04πqv×rr3
B=4π×10−74π(6×10−6)(−4×106^k)(0.5)3
=10−7−24×100^k0.125=−1.92×10−5^kT
Thus, the magnetic field at point (0.500 m, 0, 0) is:
B=−1.92×10−5^kT
Part (b): Point (0,−0.500m,0)
1. Position Vector:
r=0^i−0.5^j+0^k=−0.5^j
2. Magnitude of r:
r=|r|=0.5m
3. Cross Product v×r:
v×r=(8×106^j)×(−0.5^j)=0
4. Substituting into the Magnetic Field Formula:
B=μ04πq⋅0r3=0
Thus, the magnetic field at point (0, -0.500 m, 0) is:
B=0T
Final Answers:
- (a) B=−1.92×10−5^kT
- (b) B=0T
- q is the charge,
- v is the velocity of the charge,
- r is the position vector from the charge to the point where we want to calculate the magnetic field,
- r is the magnitude of the position vector,
- μ0 is the permeability of free space (μ0=4π×10−7T m/A).
- Charge, q=+6μC=6×10−6C
- Velocity, v=8×106m/s^j
- Points to calculate B:
- (a) x=0.500m,y=0,z=0
- (b) x=0,y=−0.500m,z=0
r=0.5^i+0^j+0^k=0.5^i
r=|r|=0.5m
v=8×106^j
v×r=(8×106^j)×(0.5^i)=8×106×0.5(^j×^i)=4×106(−^k)=−4×106^k
B=μ04πqv×rr3
B=4π×10−74π(6×10−6)(−4×106^k)(0.5)3
=10−7−24×100^k0.125=−1.92×10−5^kT
B=−1.92×10−5^kT
r=0^i−0.5^j+0^k=−0.5^j
r=|r|=0.5m
v×r=(8×106^j)×(−0.5^j)=0
B=μ04πq⋅0r3=0
B=0T
- (a) B=−1.92×10−5^kT
- (b) B=0T
Topper's Solved these Questions
- SOURCES OF MAGNETIC FIELDBook:CENGAGE PHYSICS ENGLISHChapter:SOURCES OF MAGNETIC FIELDExercise:Concept Exercise 2.2
Explore 10 Videos
- SOURCES OF MAGNETIC FIELDBook:CENGAGE PHYSICS ENGLISHChapter:SOURCES OF MAGNETIC FIELDExercise:Exercise (subjective )
Explore 10 Videos
- SOURCES OF MAGNETIC FIELDBook:CENGAGE PHYSICS ENGLISHChapter:SOURCES OF MAGNETIC FIELDExercise:Solved Example
Explore 20 Videos
- RAY OPTICSBook:CENGAGE PHYSICS ENGLISHChapter:RAY OPTICSExercise:DPP 1.6
Explore 12 Videos
- WAVE OPTICSBook:CENGAGE PHYSICS ENGLISHChapter:WAVE OPTICSExercise:Comprehension Type
Explore 14 Videos
Similar Questions
In a certain region of space, the electric field is along +y-direction and has a magnitude of 400 V/m . What is the potential difference from the coordinate origin to the following points?
a.x=0,y=20cm,z=0
b. x=0,y=−30cm,z=0
c.x=0,y=0,z=15cm
View SolutionA point charge q=−8.0nC is located at the origin. Find the electric field vector at the point x=1.2m,y=−1.6m.
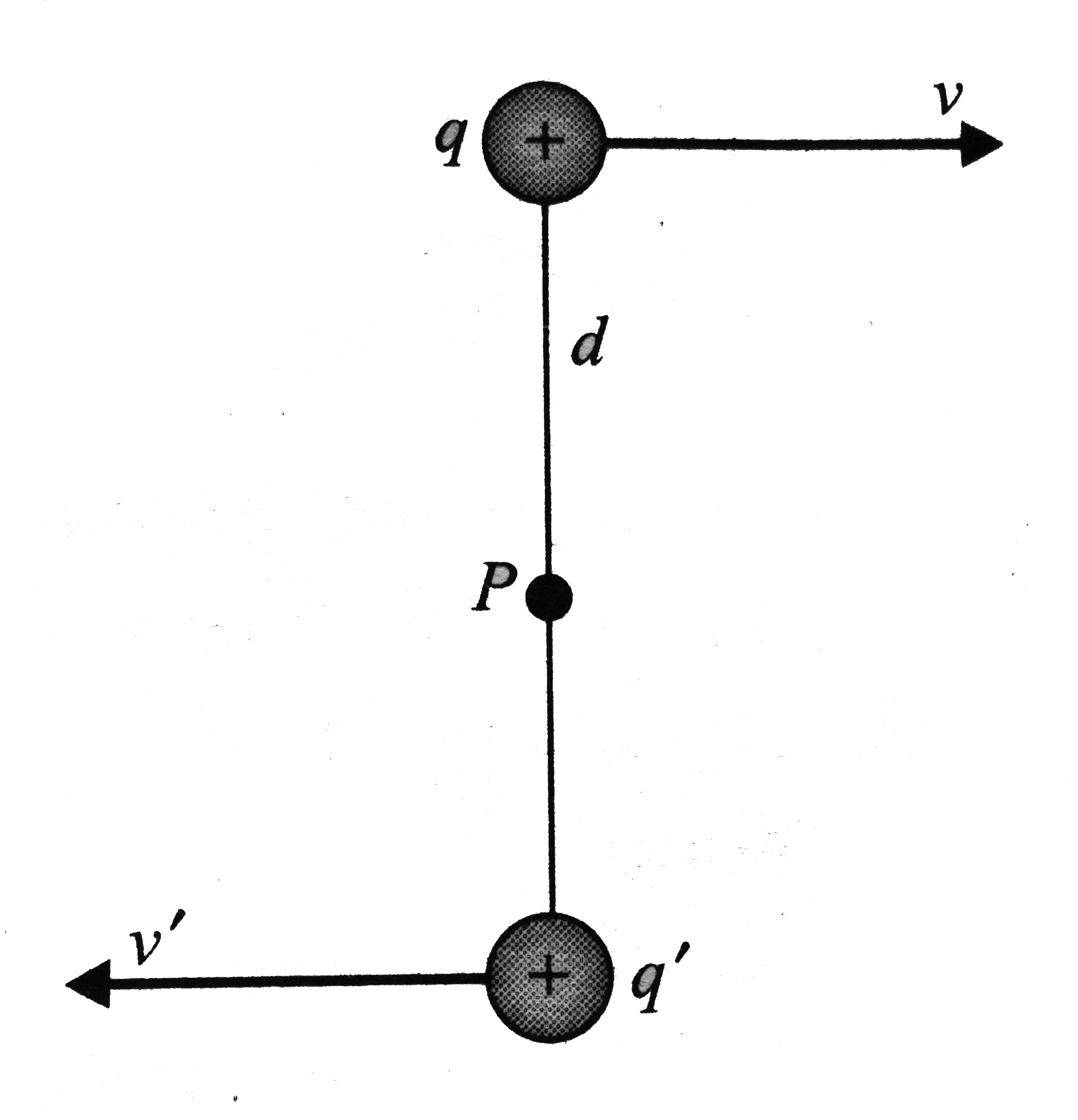
View SolutionPositive point charge q=+8.00μCandq'=+3.00μC are moving relative to an observer in Fig. The distance d is 0.120m. When the two charges are at the locations shown in the figure, what are the magnitude and direction of the net magnetic field they produce at point P? (Takev=4.50×106ms−1andv'=9.00×106ms1)
View SolutionA long straight wire along the z- axis carries a current I in the negative z−direction . The magnetic vector field →B at a point having coordinates (x,y) in the Z=0 plane is
View SolutionA long straight wire along the z- axis carries a current I in the negative z−direction . The magnetic vector field →B at a point having coordinates (x,y) in the Z=0 plane is
View SolutionIf the sum of the squares of the distances of the
point (x, y, z) from the points (a, 0, 0) and (-a, 0, 0)
is 2c2, then which are of the following is
correct?
View SolutionThere is a constant homogeneous electric field of 100Vm−1 within the region x=0andx=0.167m pointing in x-direction. There is a constant homogeneous mangetic field B within the region x=0.167mandx=0.334m pointing in the z-direction. A proton at rest at the origin is released in positive x-direction. Find the minimum strength of the magnetic field B, so that the proton is detected back at x=0,y=0.167m. (mass of proton =1.67×10−27kg)
View SolutionA particle of mass 1×10−26kg and charge +1.6×10−19C travelling with a velocity 1.28×106ms−1 in the +x direction enters a region in which uniform electric field E and a uniform magnetic field of induction B are present such that Ex=Ey=0,Ez=−102.4kVm−1,andBx=Bz=0,By=8×10−2. The particle enters this region at time t=0. Determine the location (x,y,z coordinates) of the particle at t=5×10−6s. If the electric field is switched off at this instant (with the magnetic field present), what will be the position of the particle at t=7.45×10−6s ?
View SolutionTwo point charges (Q each are placed at (0,y) and (0-y) A point charge q of the same polarity can move along the x-axis. Then
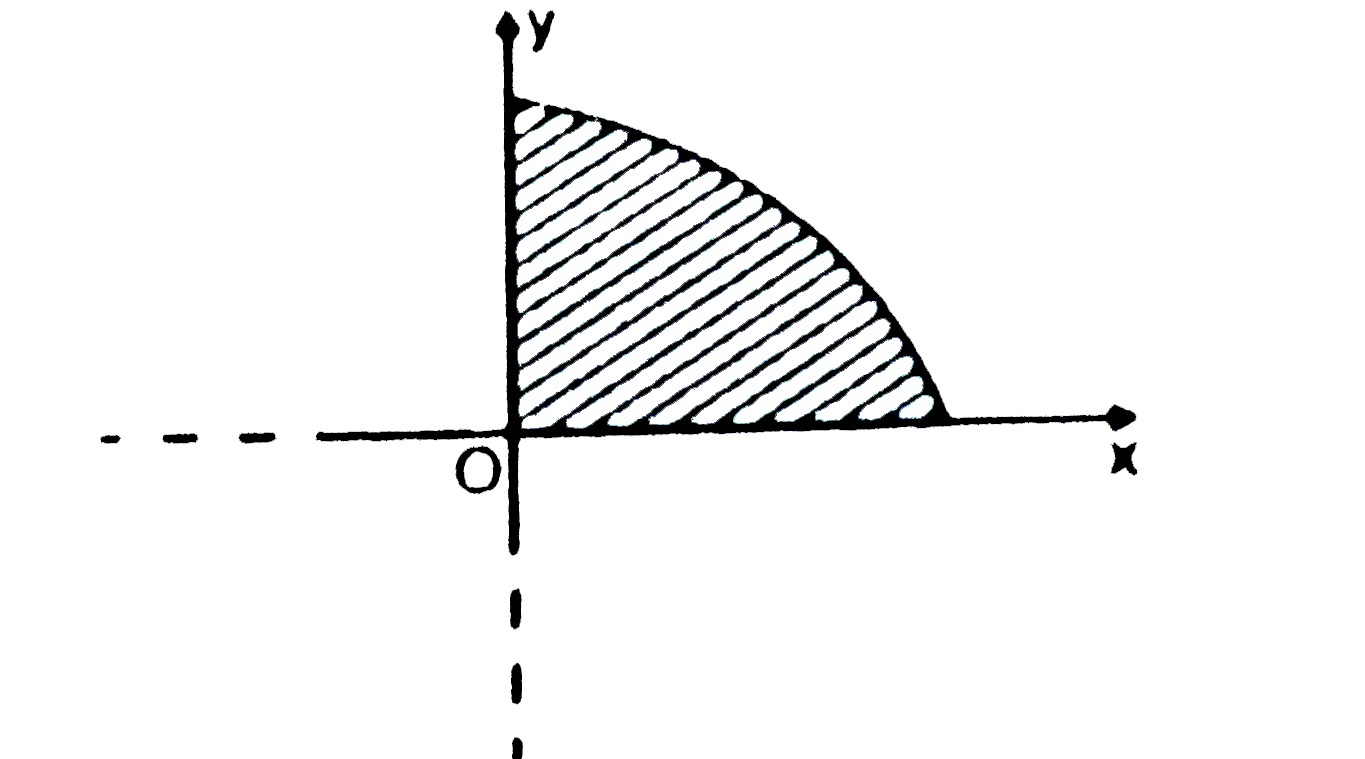
View SolutionA uniform surface charge of density σ is given to a quarter of a disc extending upto infinity in the first quadrant of x-y plane. The centre of the disc is at the origin O. Find the z-component of the electric field at the point (0,0,z) and the potential difference between the points (0,0,d) & (0,0,2d).
View Solution