Uniform electric and magnetic fields with strength E and induction B, respectively, are along y-axis as shown in Fig. A particle with specific charge q/m leaves the origin O in the direcction of x-axis with an initial non-relativistic velocity v0

The angle α between the particle's velocity vector and y-axis at and moment is
tan−1(3v0B2πnE)
tan−1(v0BπnE)
tan−1(v0B√2πnE)
tan−1(v0B2πnE)
The correct Answer is:d
Particle's acceleration in in y direction.
dvydt=qEm= constant
The motion of the particle is equivalent to circular motion in xz
plane with uniform acceleration in y-direction.
Hence, v20=v2x+v2z=constant
The magnetic force cannot change the magnitude of v0.
The y-component of velocity,
vy=qEmt
The y-coordinate at time t, y=12qEmt2
The time period of circular motion in xz plane.
T=2πmBq
Let the particle cross y-axis after n rotations, then
t=nT=2πmnqB
Thus, yn=qE2m×(2πmnqB)2=2π2mn2EqB2
As vy=ayt=(qEm)(2πmnqB)=2πnEB
Thus, tanα=v0vy=v0B2πnE⇒α=tan−1(v0B2πnE)
Topper's Solved these Questions
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Exercises Integer
7 Videos
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Archives Fill In The Blank
6 Videos
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Exercises Assertion -reasoning
8 Videos
- INDUCTANCEBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - INDUCTANCEEXERCISE - Concept Based
8 Videos
- MISCELLANEOUS VOLUME 3BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - MISCELLANEOUS VOLUME 3EXERCISE - True and False
3 Videos
Similar Questions
Uniform electric and magnetic fields with strength E and induction B, respectively, are along y-axis as shown in Fig. A particle with specific charge q/m leaves the origin O in the direcction of x-axis with an initial non-relativistic velocity v0

The coordinate yn of the particle when it crosses the y-axis for the nth time is
View SolutionUniform electric field E and magnetic field B, respectively, are along y-axis as shown in the figure. A particle with specific charge q/m leaves the origin O in the direction of x-axis with an initial non-relativistic speed v0.Thec∞rd∈ateY_(n) of the particle when it crosses the y-axis for the nth time is:
View SolutionUniform electrilcf and magnetic fields with strength E and B are directed along of x-axis. A particle with specific charge qm leves the origin in the direction of x-axis with an initial velocity v0. Find
a. the y-coordinate of the particle when it crosses the y-xis for nth time.
b. the angle α between the particle's velocity vector adn the y-axis for nth time
View SolutionElectric field and magnetic field n a region of space is given by E=E0ˆj and B=B0ˆj. A particle of specific charge alpha is released from origin with velocity v=v0ˆi. Then path of particle
View SolutionA particle mass parallel to x-axis with constant velocity v as shown in the figure. The angular velocity of the particle about the origin O

View SolutionA uniform cube of mass m and edge a moves on a horizontal surface along the positive x-axis, with initial velocity v0. Then
View SolutionA particle of charge -q and mass m enters a uniform magnetic field →B (perpendicular to paper inward) at P with a velocity v0 at an angle α and leaves the field at Q with velocity v at angle β as shown in fig.
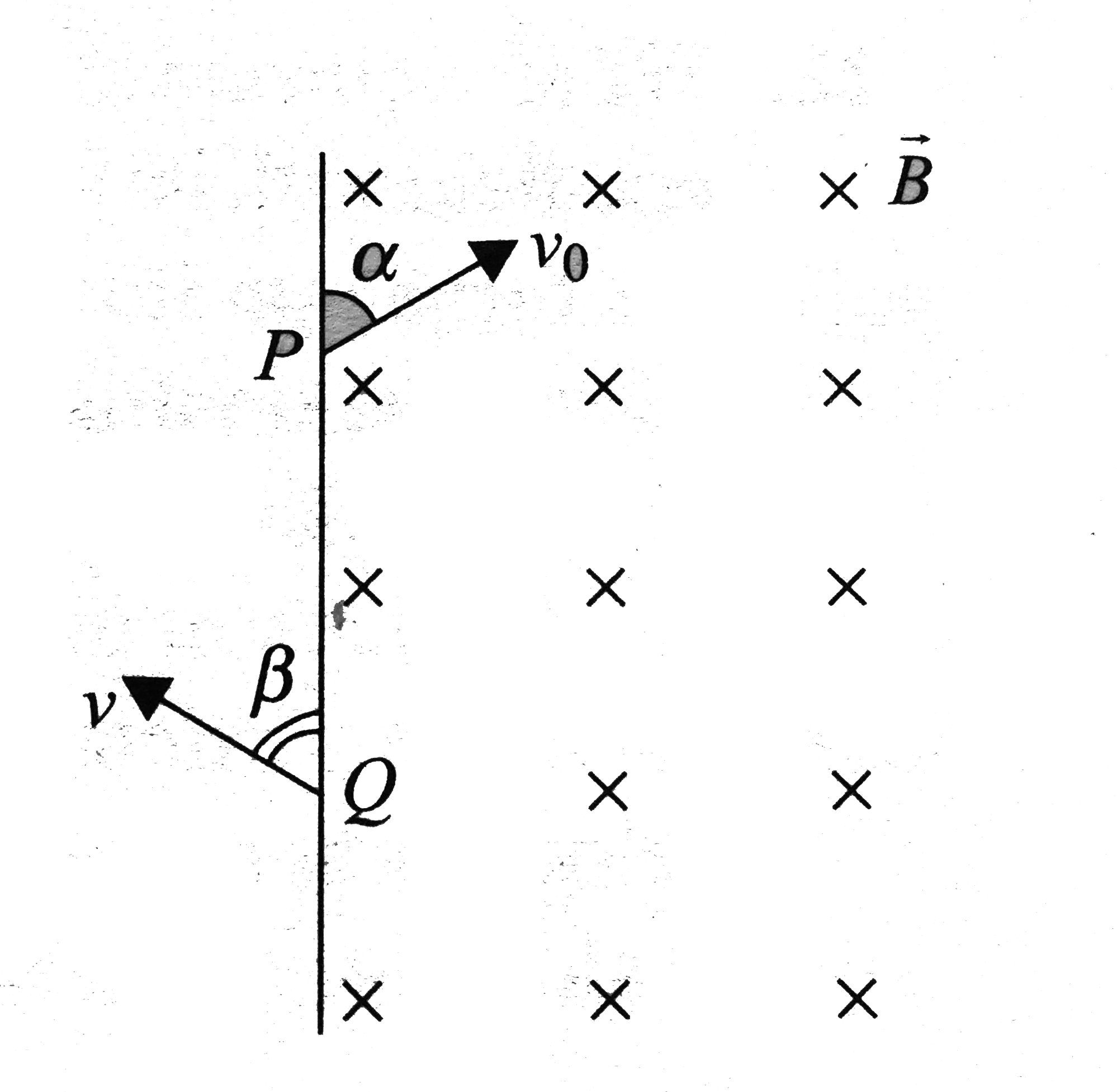
View SolutionA uniform electric field of 400V/m is directed at 45∘ to the positive X-axis, in the X−Y plane as shown in figure

View SolutionA particle of specific charge α is projected from origin with velocity v=v0ˆi−v0ˆk in a uniform magnetic field B=−B0ˆk. Find time dependence of velocity and position of the particle.
View SolutionA particle of specific charge alpha enters a uniform magnetic field B=−B0ˆk with velocity v=v0ˆi from the origin. Find the time dependence of velocity and position of the particle.

View Solution
