In a photoelectric cell, the wavelength of incident light is chaged from 4000A to 3600A. The change in stopping potential will be
0.14 V
0.24 V
0.35 V
0.44 V
The correct Answer is:C
To solve the problem of finding the change in stopping potential when the wavelength of incident light changes from 4000 Å to 3600 Å in a photoelectric cell, we will use the photoelectric effect equation derived from Einstein's theory. Here’s a step-by-step solution:
Step 1: Understand the Photoelectric Effect Equation
The energy of the incident photons can be expressed using the equation:
E=hcλ
Where:
- E is the energy of the photon,
- h is Planck's constant (6.626×10−34Js),
- c is the speed of light (3×108m/s),
- λ is the wavelength of the incident light.
The stopping potential V0 is related to the energy of the photon and the work function W of the material:
eV0=hcλ−W
Where e is the charge of an electron (1.6×10−19C).
Step 2: Set Up the Equations
For two different wavelengths λ1=4000Å and λ2=3600Å, we can write two equations:
1. For λ1:
eV01=hcλ1−W
2. For λ2:
eV02=hcλ2−W
Step 3: Find the Change in Stopping Potential
Subtract the first equation from the second:
eV02−eV01=hcλ2−hcλ1
This simplifies to:
e(V02−V01)=hc(1λ2−1λ1)
Step 4: Solve for the Change in Stopping Potential
Rearranging gives:
V02−V01=hce(1λ2−1λ1)
Step 5: Substitute the Values
Substituting the known values:
- h=6.626×10−34Js
- c=3×108m/s
- e=1.6×10−19C
- Convert wavelengths from Ångströms to meters:
- λ1=4000Å=4000×10−10m
- λ2=3600Å=3600×10−10m
Now, plug in the values:
V02−V01=(6.626×10−34)(3×108)1.6×10−19(13600×10−10−14000×10−10)
Step 6: Calculate the Values
Calculating 13600×10−10−14000×10−10:
=13.6×10−7−14.0×10−7=4−3.614.4×107=0.414.4×107=136×107
Now substituting back:
V02−V01=(6.626×10−34)(3×108)1.6×10−19×136×107
Calculating the numerical values yields:
V02−V01≈0.35V
Final Answer
The change in stopping potential is approximately 0.35V.
---
The energy of the incident photons can be expressed using the equation:
E=hcλ
Where:
- E is the energy of the photon,
- h is Planck's constant (6.626×10−34Js),
- c is the speed of light (3×108m/s),
- λ is the wavelength of the incident light.
eV0=hcλ−W
Where e is the charge of an electron (1.6×10−19C).
For two different wavelengths λ1=4000Å and λ2=3600Å, we can write two equations:
1. For λ1:
eV01=hcλ1−W
2. For λ2:
eV02=hcλ2−W
Subtract the first equation from the second:
eV02−eV01=hcλ2−hcλ1
This simplifies to:
e(V02−V01)=hc(1λ2−1λ1)
Rearranging gives:
V02−V01=hce(1λ2−1λ1)
Substituting the known values:
- h=6.626×10−34Js
- c=3×108m/s
- e=1.6×10−19C
- Convert wavelengths from Ångströms to meters:
- λ1=4000Å=4000×10−10m
- λ2=3600Å=3600×10−10m
V02−V01=(6.626×10−34)(3×108)1.6×10−19(13600×10−10−14000×10−10)
Calculating 13600×10−10−14000×10−10:
=13.6×10−7−14.0×10−7=4−3.614.4×107=0.414.4×107=136×107
V02−V01=(6.626×10−34)(3×108)1.6×10−19×136×107
V02−V01≈0.35V
The change in stopping potential is approximately 0.35V.
Topper's Solved these Questions
- PHOTOELECTRIC EFFECTBook:CENGAGE PHYSICS ENGLISHChapter:PHOTOELECTRIC EFFECTExercise:Multiple Correct
Explore 10 Videos
- PHOTOELECTRIC EFFECTBook:CENGAGE PHYSICS ENGLISHChapter:PHOTOELECTRIC EFFECTExercise:Linked Comprehension
Explore 44 Videos
- PHOTOELECTRIC EFFECTBook:CENGAGE PHYSICS ENGLISHChapter:PHOTOELECTRIC EFFECTExercise:Subjective
Explore 16 Videos
- NUCLEAR PHYSICSBook:CENGAGE PHYSICS ENGLISHChapter:NUCLEAR PHYSICSExercise:ddp.5.5
Explore 14 Videos
- RAY OPTICSBook:CENGAGE PHYSICS ENGLISHChapter:RAY OPTICSExercise:DPP 1.6
Explore 12 Videos
Similar Questions
Knowledge Check
When light of wavelength 400nm is incident on the cathode of photocell, the stopping potential recorded is 6V. If the wavelength of the incident light is to 600nm, calculate the new stopping potential. [Given h=6.6×10−34Js,c=3×108m/s,e=1.6×10−19C]
A1.03VB2.42VC4.97VD3.58V
if the wavelength is chaged from4000A.→3000A, then stopping potential will become
View SolutionIn a photoelectric experiment, the wavelength of the incident light is decreased from 6000A to 4000A. While the intensity of radiations remains the same,
View SolutionSuppose the wavelength of the incident light is increased from 3000Å to 3040Å. Find the corresponding change in the stopping potential. [Take the product hc=12.4×10−7eVm)]
View SolutionIn a photoelectric experiment, the wavelength of the light incident on a metal is changed from 300 nm to 400 nm. Choose the closest value of change in the stopping potential from given options (hce=1240nm.V)
View SolutionIn a photoelectric experiment, the wavelength of the light incident on a metal is changed from 300 nm to 400 nm. Choose the closest value of change in the stopping potential from given options (hce=1240nm.V)
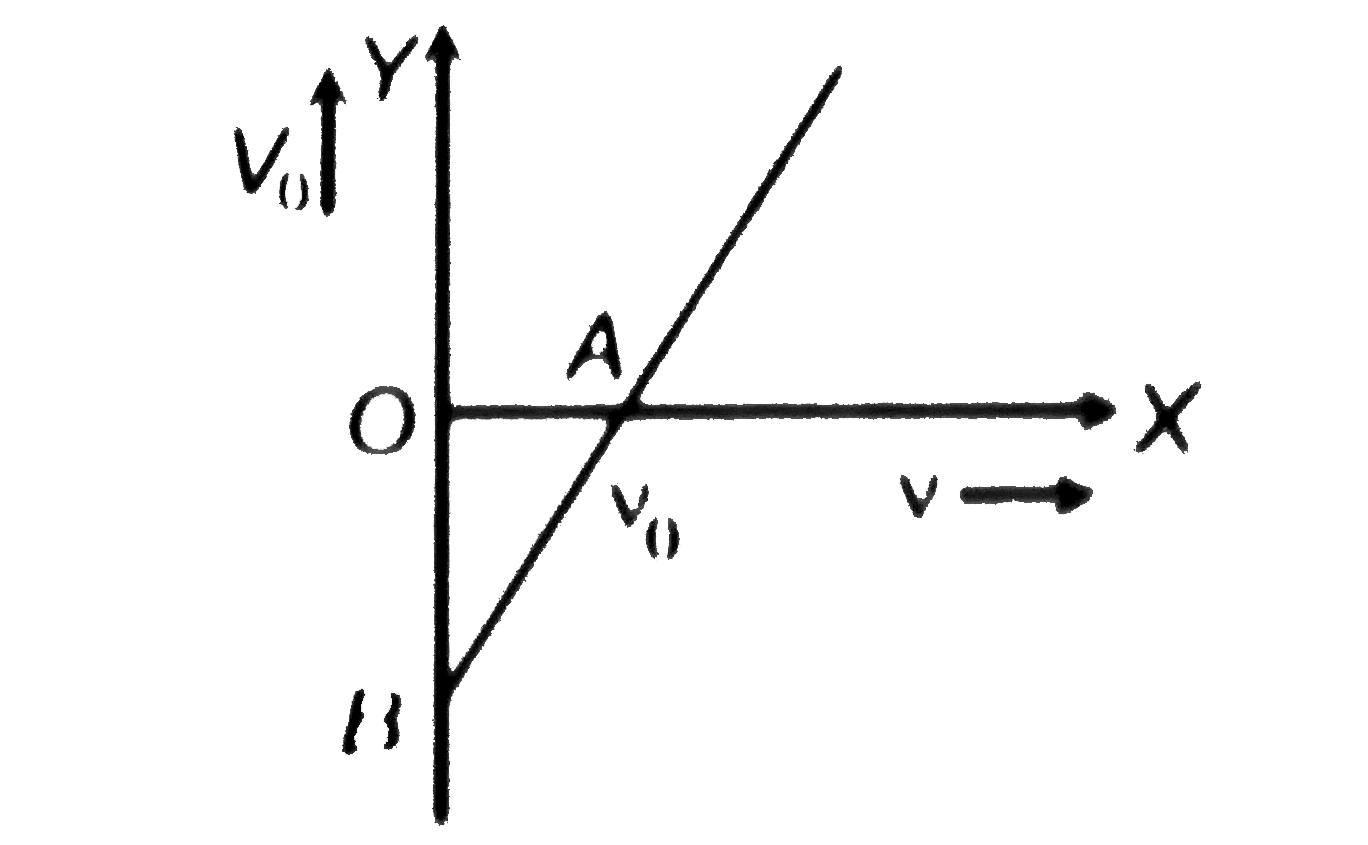
View SolutionIn an experiment on photoelectric effect the frequency f of the incident light is plotted against the stopping potential V0. The work function of the photoelectric surface is given by ( e is the electronic charge)
View Solutiona. A stopping potential of 0.82V is required to stop the emission of photoelectrons from the surface of a metal by light of eavelength 4000A. For light of wavelength 3000A, the stopping potential is 1.85V. Find the value of Planck's constant. [1 electrons volt (eV)=1.6×10−19J]
b. At stopping potential, if the wavelength of the incident light is kept fixed at 4000A, but the intensity of light increases two times, will photoelectric current be botained ? Give reasons for your answer.
View Solution