White light is used in a YDSE with D=1m and d=0.9mm. Light reaching the screen at position y=1mm us passed through a prism and its spectrum is obtained. Find the missing lines in the visible region of this spectrum.
To solve the problem step by step, we will follow the outlined procedure to find the missing lines in the visible spectrum produced by the Young's Double Slit Experiment (YDSE) using white light.
Step 1: Understand the Given Values
We have the following values:
- Distance to the screen D=1m
- Slit separation d=0.9mm=0.9×10−3m
- Position on the screen y=1mm=1×10−3m
Step 2: Calculate the Path Difference
The path difference Δx for the light reaching the screen can be calculated using the formula:
Δx=y⋅dD
Substituting the values:
Δx=(1×10−3)⋅(0.9×10−3)1
Calculating this gives:
Δx=0.9×10−6m=900nm
Step 3: Determine the Condition for Minima
For minima in the interference pattern, the path difference is given by:
Δx=(2n−1)λ2
where n is the order of the minima and λ is the wavelength of light.
Step 4: Set Up the Equation
Equating the two expressions for path difference:
900nm=(2n−1)λ2
Rearranging gives:
λ=1800nm2n−1
Step 5: Calculate Wavelengths for Different Orders
Now we will calculate λ for different values of n:
- For n=1:
λ=1800nm2⋅1−1=1800nm(Not in visible range)
- For n=2:
λ=1800nm2⋅2−1=600nm(In visible range)
- For n=3:
λ=1800nm2⋅3−1=360nm(Not in visible range)
- For n=4:
λ=1800nm2⋅4−1=720nm(Not in visible range)
Step 6: Identify the Missing Lines in the Visible Spectrum
From the calculations:
- λ=600nm (in the visible range, corresponds to orange light)
- λ=360nm (not in the visible range)
- λ=1800nm (not in the visible range)
Thus, the only wavelength in the visible spectrum is:
- 600 nm
Final Answer
The missing line in the visible region of the spectrum is 600 nm.
---
We have the following values:
- Distance to the screen D=1m
- Slit separation d=0.9mm=0.9×10−3m
- Position on the screen y=1mm=1×10−3m
The path difference Δx for the light reaching the screen can be calculated using the formula:
Δx=y⋅dD
Substituting the values:
Δx=(1×10−3)⋅(0.9×10−3)1
Calculating this gives:
Δx=0.9×10−6m=900nm
For minima in the interference pattern, the path difference is given by:
Δx=(2n−1)λ2
where n is the order of the minima and λ is the wavelength of light.
Equating the two expressions for path difference:
900nm=(2n−1)λ2
Rearranging gives:
λ=1800nm2n−1
Now we will calculate λ for different values of n:
- For n=1:
λ=1800nm2⋅1−1=1800nm(Not in visible range)
- For n=2:
λ=1800nm2⋅2−1=600nm(In visible range)
- For n=3:
λ=1800nm2⋅3−1=360nm(Not in visible range)
- For n=4:
λ=1800nm2⋅4−1=720nm(Not in visible range)
From the calculations:
- λ=600nm (in the visible range, corresponds to orange light)
- λ=360nm (not in the visible range)
- λ=1800nm (not in the visible range)
- 600 nm
The missing line in the visible region of the spectrum is 600 nm.
Topper's Solved these Questions
- WAVE OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Subjective
11 Videos
- WAVE OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Single Correct
128 Videos
- WAVE OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Exercise 2.1
12 Videos
- SOURCES OF MAGNETIC FIELDBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOURCES OF MAGNETIC FIELDEXERCISE - single correct Ansewer type
12 Videos
Similar Questions
The band of seven colours obtained on a screen when white light passes through a prism is called spectrum. ____
View SolutionIn a YDSE, D=1M,d=1mm,andλ=1/2mm.
(a) Find the distance between the first and central maxima on the screen.
(b) Find the number of maxima and minima obtained on the screen.
View Solution
Knowledge Check
When a beam of white light is passed through sodium vapours and then through a spectrometer, spectrum so obtained has two dark lines present in the yellow region. This spectrum is called :
AA) band spectrumBB) continuous spectrumCC) absorption spectrum of sodiumDD) emission spectrum of sodium
In YDSE with d=1mm and D=1m, Slabs of (t=1μm,μ=3) and (t=0.5μm,μ=2) are introduced in front of upper and lower slits, respectively. Find the shift in the fringe pattern.
View SolutionA ray of white light is passed through a glass prism and a spectrum is obtained on a screen.
(a) Name the seven colours of the spectrum in order.
(b) Do the colours have the same width in the spectrum ?
(c) Which colour of the spectrum of white light deviates (i) the most, (ii) the least?
View SolutionFind the two longest wavelength (inÅ) emitted when hydrogen atom make transition and the spectrum lines lie in the visible region (R=1.097×107m−1)
View SolutionIn a standard YDSE slit width is 1mm and distance of screen from the slit is 1m. If wavelength of light is 632nm and bright fringe formed at y = 1.270 mm. Find path difference for the point
View SolutionMonochromatic light of wavelength 5000Å is used in YDSE, with slit width, d=1mm, distance between screen and slits, D=1M. If intensites at the two slits are I1=4I0andI2=I0, find:
a. finge width β:
b. distance of 5th minima from the central maxima on the screen,
c. intensity at y=13mm,
d. distance of the 1000th maxima, and
e. distance of the 5000th maxima.
View SolutionWhite coherent light (400nm−700nm) is sent through the slits of a YDSE. d=0.5mm, D=50 cm. There is a hole in the screen at a point 1.0mm away (along the width of the fringes) from the central line.
(a) Which wavelength will be absent in the light coming from the hole?
(b) Which wavelength(s) will have a strong intensity?
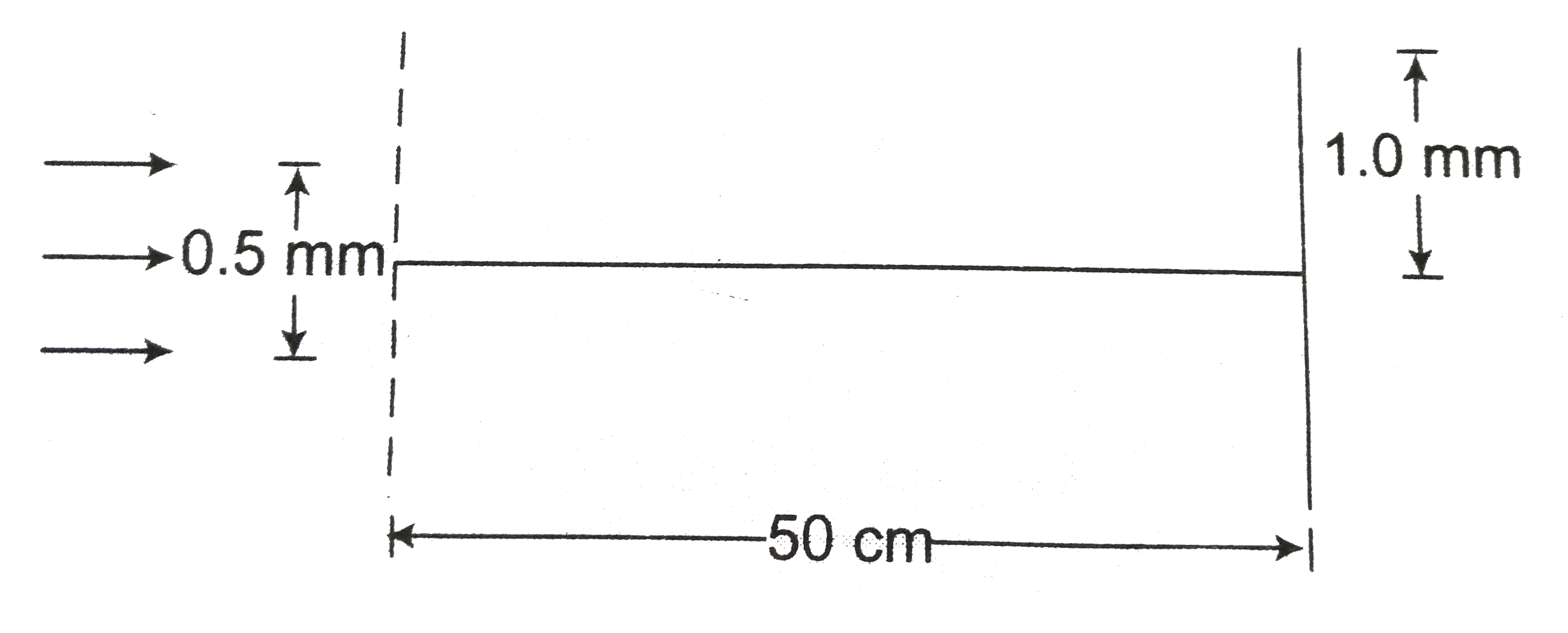
View SolutionWhite coherent light (400nm−700nm) is sent through the slits of a YDSE. d=0.5mm, D=50 cm. There is a hole in the screen at a point 1.0mm away (along the width of the fringes) from the central line.
(a) Which wavelength will be absent in the light coming from the hole?
(b) Which wavelength(s) will have a strong intensity?

View Solution
