A diverging lens of focal length is placed in front of and coaxially with a concave mirror of foacl length . Their separation is d. A parallel beam of light incident on the lens returns as a parallel beam from the arrangement. Then,
the beam diameters of the incident and reflected beams must be the same
if the entire arrrangement is immersed in water, the conditions will remain unaltered
The correct Answer is:A, B
To solve the problem, we need to analyze the optical arrangement consisting of a diverging lens and a concave mirror. The goal is to determine the relationship between the focal lengths of the lens and mirror, as well as the separation between them, given that a parallel beam of light incident on the lens returns as a parallel beam after reflection.
1. Understanding the Setup:
- We have a diverging lens (focal length f1) and a concave mirror (focal length f2).
- The distance between the lens and the mirror is d.
2. Ray Diagram:
- Draw a ray diagram to visualize the situation. A parallel beam of light strikes the diverging lens.
- The lens will cause the rays to diverge. The diverging rays appear to come from a virtual focus on the same side as the incoming light.
3. Finding the Virtual Focus of the Lens:
- For a diverging lens, the focal length f1 is negative.
- The virtual focus F1 of the lens is located at a distance |f1| from the lens on the same side as the incoming light.
4. Reflected Rays from the Mirror:
- The diverging rays from the lens will reach the concave mirror.
- The mirror will reflect these rays. The concave mirror has a focal length f2 (positive value).
5. Condition for Parallel Rays:
- For the light to return as a parallel beam after reflection, the rays must converge at the focal point of the mirror.
- The distance from the mirror to the virtual focus F1 must equal the focal length of the mirror, which means:
d−|f1|=f2
6. Rearranging the Equation:
- Rearranging the equation gives:
d=f2+|f1|
7. Conclusion:
- The separation d between the lens and the mirror is equal to the sum of the absolute value of the focal length of the diverging lens and the focal length of the concave mirror.
Final Relationship:
d=|f1|+f2
- We have a diverging lens (focal length f1) and a concave mirror (focal length f2).
- The distance between the lens and the mirror is d.
- Draw a ray diagram to visualize the situation. A parallel beam of light strikes the diverging lens.
- The lens will cause the rays to diverge. The diverging rays appear to come from a virtual focus on the same side as the incoming light.
- For a diverging lens, the focal length f1 is negative.
- The virtual focus F1 of the lens is located at a distance |f1| from the lens on the same side as the incoming light.
- The diverging rays from the lens will reach the concave mirror.
- The mirror will reflect these rays. The concave mirror has a focal length f2 (positive value).
- For the light to return as a parallel beam after reflection, the rays must converge at the focal point of the mirror.
- The distance from the mirror to the virtual focus F1 must equal the focal length of the mirror, which means:
d−|f1|=f2
- Rearranging the equation gives:
d=f2+|f1|
- The separation d between the lens and the mirror is equal to the sum of the absolute value of the focal length of the diverging lens and the focal length of the concave mirror.
d=|f1|+f2
Topper's Solved these Questions
- GEOMETRICAL OPTICSBook:CENGAGE PHYSICS ENGLISHChapter:GEOMETRICAL OPTICSExercise:Assertion-Reasoninig
Explore 2 Videos
- GEOMETRICAL OPTICSBook:CENGAGE PHYSICS ENGLISHChapter:GEOMETRICAL OPTICSExercise:Linked Comprehension
Explore 51 Videos
- GEOMETRICAL OPTICSBook:CENGAGE PHYSICS ENGLISHChapter:GEOMETRICAL OPTICSExercise:Subjective
Explore 26 Videos
- ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSBook:CENGAGE PHYSICS ENGLISHChapter:ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSExercise:dpp 3.3
Explore 15 Videos
- HEATING EFFECT OF CURRENTBook:CENGAGE PHYSICS ENGLISHChapter:HEATING EFFECT OF CURRENTExercise:Thermal Power in Resistance Connected in Circuit
Explore 27 Videos
Similar Questions
A converging lens of focal length f1 is placed in front of and coaxially with a convex mirror of focal length f2. Their separation is d. A parallel beam of light incident on the lens returns as a parallel beam from the arrangement, Then,
View SolutionA convex lens of focal length f1 is kept in contact with a concave lens of focal length f2. Find the focal length of the combination.
View SolutionA convex lens of focal length 20cm and a concave lens of focal length f are mounted coaxially 5cm apart. Parallel beam of light incident on the convex lens emerges from the concave lens as a parallel beam. Then, f in cm is
View SolutionA convex lens of focal length 100 cm and a concave lens of focal length 10 cm are placed coaxialiy at a separation of 90 cm. If a parallel beam of light is incident on convex lens, then after passing through the two lenses the beam
View SolutionA convex lens of focal length 1.0m and a concave lens of focal length 0.25m are 0.75m apart. A parallel beam of light is incident on the convex lens. The beam emerging after refraction from both lenses is
View SolutionA biconvex lens of focal length f1=10cm is placed 40 cm in front of a concave mirror of focal length f2=7.50cm, as shown in the figure. An object, 2 cm high, is placed 20 cm to the left of the lens.
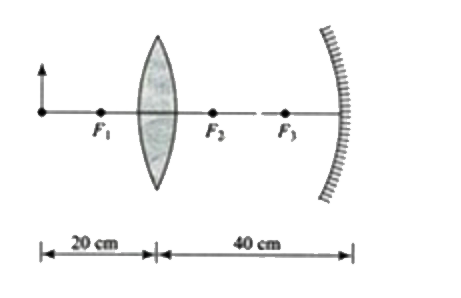
The image formed by the lens, as rays travel to the right, is
View SolutionTwo converging lenses of focal lengths f1=10cm and f2=20cm are placed at some separation. A parallel beam of light is incident on first lens. Then,
View SolutionA diverging lens of focal length 10cm is placed 10cm in front of a plane mirror as shown in Fig. Light from a very far away source falls on the lens. What is the distance of final image?
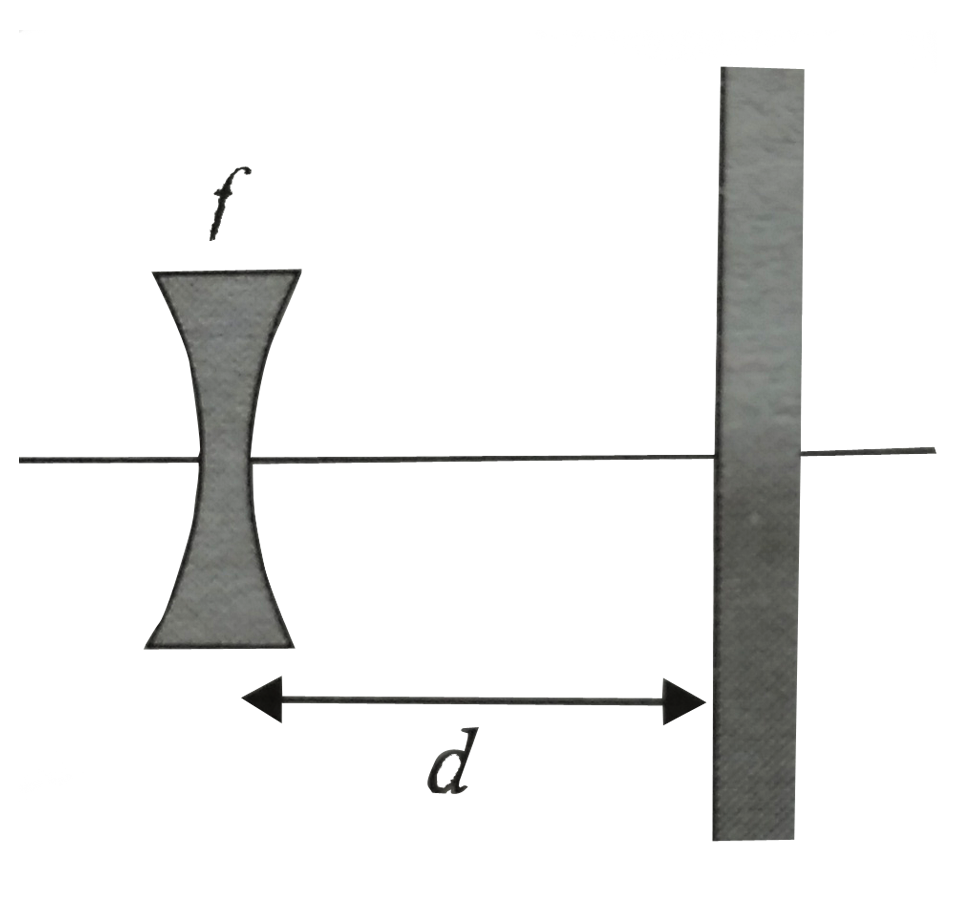
View SolutionAn object is placed in front of a concave mirror of focal length f as shown in figure. Choose the correct shape of the image.

View SolutionIn the figure shown a converging lens and a diverging lens of focal lengths 20cm and -5cm respectively are separated by a distance such that a paralel beam of light of intensity 100 wa/m2 incident on the converging lens comes out as parallel beam from the diverging lens. Find the intensity of the outgoing beam in wa/m2
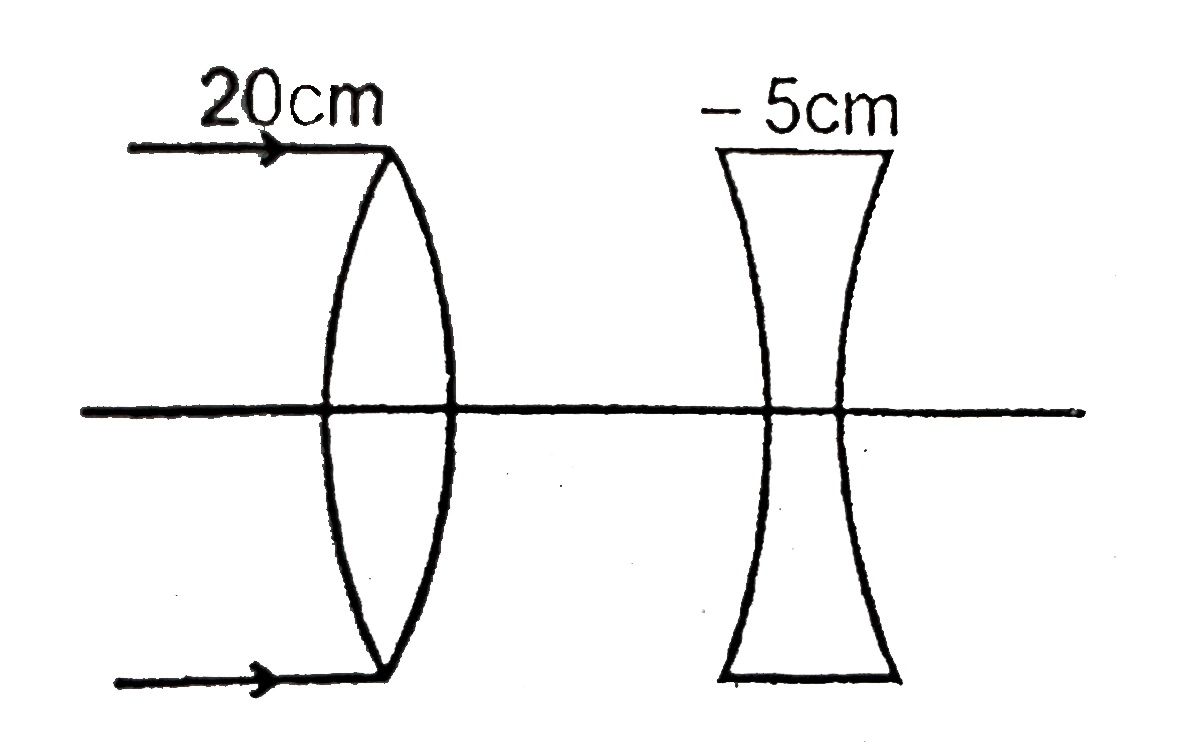
View Solution
