In the above question, if the rays were to converge beyond C, then find the nature of final image formed.
real
virtual
erect
inverted
The correct Answer is:b.,d.
To solve the problem regarding the nature of the final image formed when the rays converge beyond point C in a geometrical optics scenario, we can follow these steps:
1. Understand the Setup: In a lens or mirror system, point C is the center of curvature, and point F is the focal point. When rays converge beyond C, it implies that the object is placed between F and C.
2. Identify the Image Characteristics: When the object is placed between F and C, the rays of light that are parallel to the principal axis converge after passing through the lens or reflecting off the mirror.
3. Determine the Image Position: Since the rays converge beyond C, the image will be formed on the opposite side of the object. This indicates that the image is real.
4. Analyze the Image Orientation: The image formed in this scenario is inverted. This is a common characteristic of real images formed by converging rays.
5. Assess the Size of the Image: When the object is between F and C, the image formed is diminished, meaning it is smaller than the object.
6. Conclude the Nature of the Image: Based on the above analysis, the final image formed when the rays converge beyond C is real, inverted, and diminished.
Final Answer:
The nature of the final image formed when the rays converge beyond C is that it is real, inverted, and diminished.
The nature of the final image formed when the rays converge beyond C is that it is real, inverted, and diminished.
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Assertion-Reasoninig
2 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Linked Comprehension
51 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Subjective
26 Videos
- ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSEXERCISE - dpp 3.3
15 Videos
- HEATING EFFECT OF CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Thermal Power in Resistance Connected in Circuit
27 Videos
Similar Questions
In the above question, if the rays were to converge between F and C of mirror, then find the nature of final image formed
View SolutionIn Figure., find the position of final image formed.
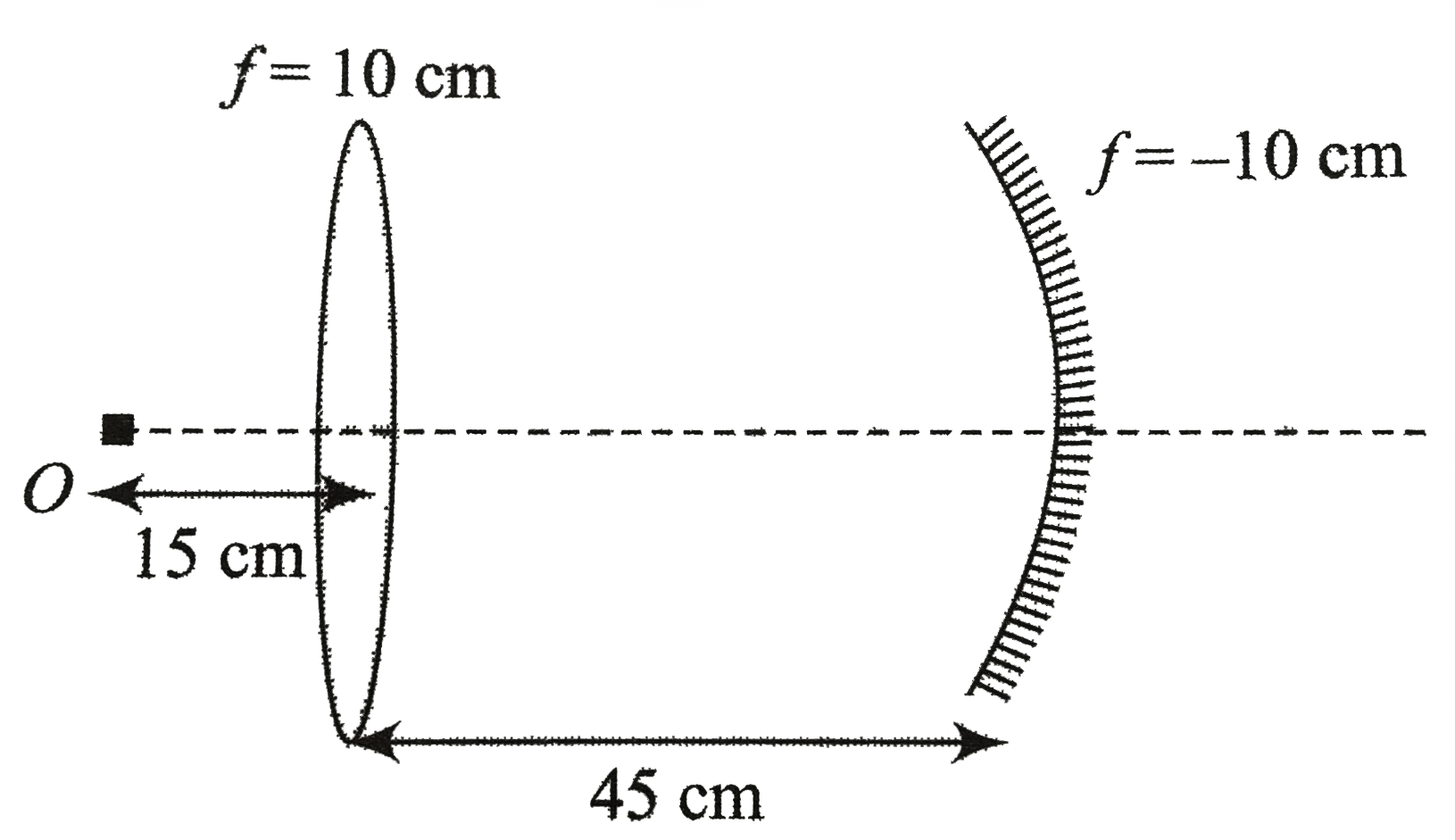
View SolutionIn Figure. , find the position of final image formed.
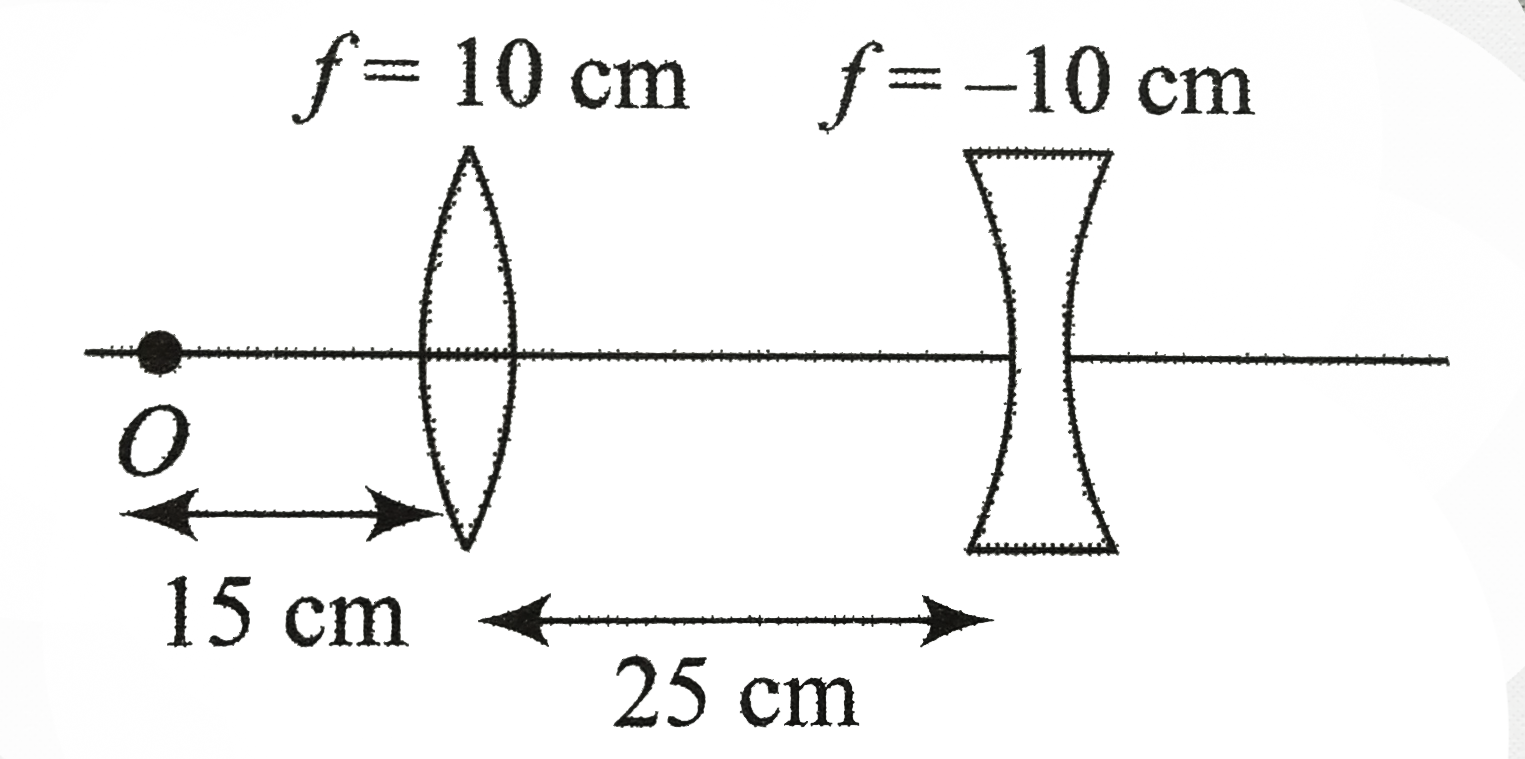
View SolutionIn Gallilean telescope, the final image formed is
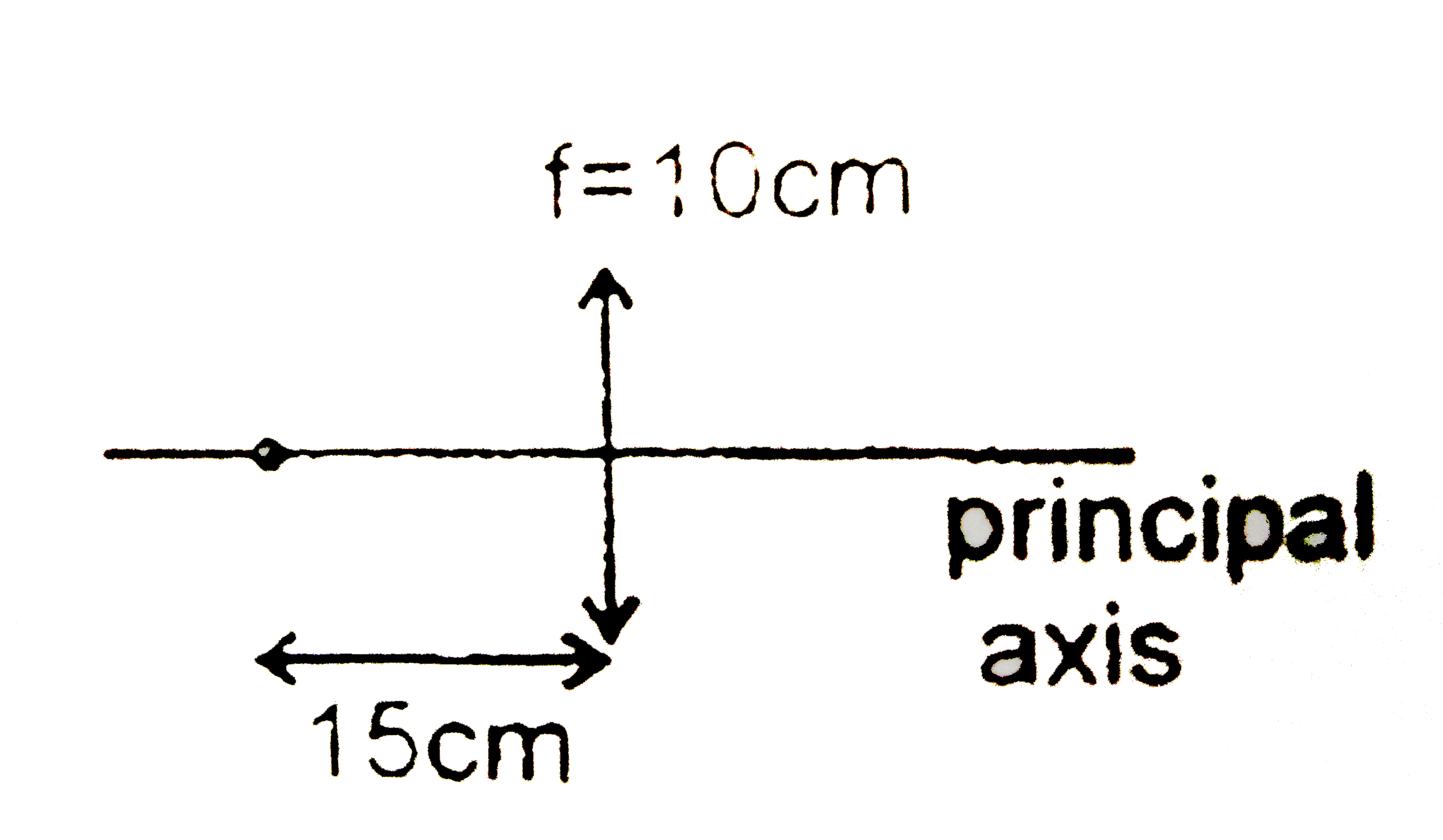
View SolutionFigure shows a point object and a converging lens. Find the final image formed.
View SolutionAll objects referred to the subsequent problems lie on the principal axis.
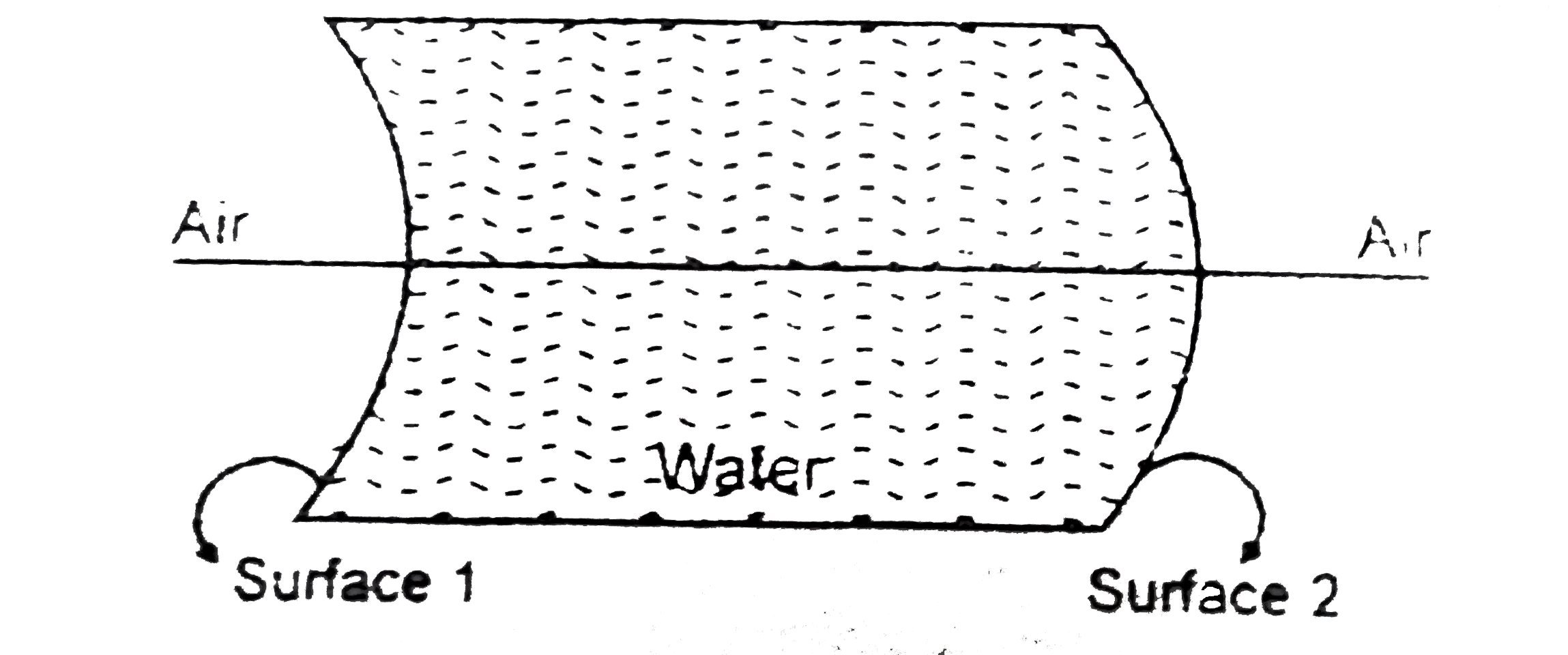
In above question if the object is real, then the final image formed after two refractions :
View SolutionFigure shows a point object and a diverging lens
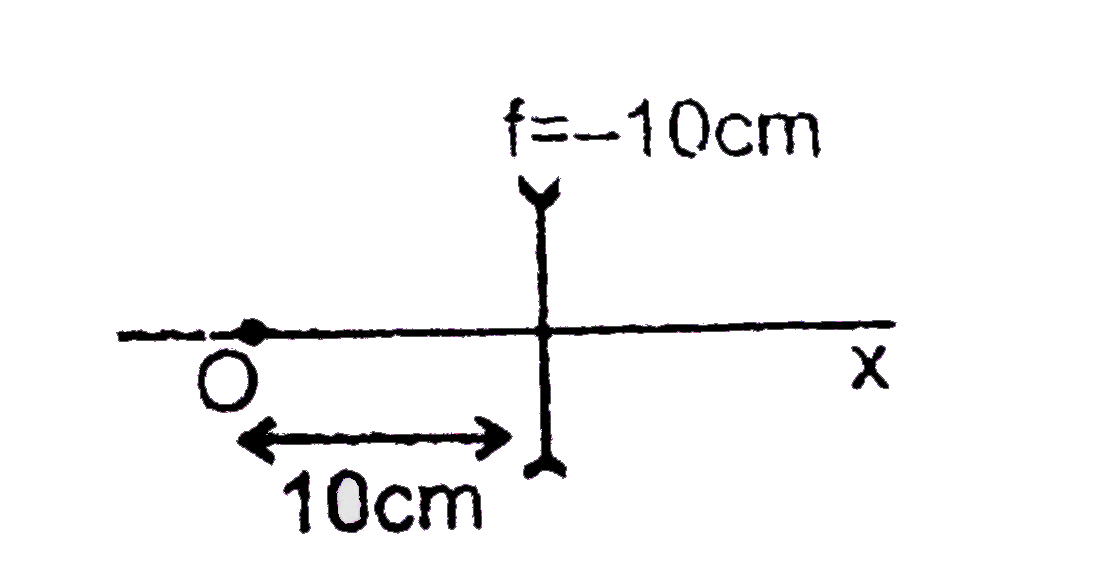
Find the final image formed.
View SolutionA parallel bean of light travelling in water (refractive index = 4/3) is refracted by a spherical bubble of radius 2 mm situation in water. Assuming the light rays to be paraxial. i. find the position of the image due to refraction at the first surface and the position of the final image, and ii. draw a ray diagram showing the positions of the images.
View SolutionAn object is kept in front of a concave lens.What is the nature of the image formed?
View SolutionIn the figure shown, find the relative speed of a approach/ separation of the two final images formed after the light rays pass through the lens, at the moment when u = 30 cm . The speed of object = 4 cm/s . The two lens halves are placed symmetrically w.r.t. the moving object
_E01_157_Q01.png)
View Solution
