A point source of light is placed at a distance h below the surface of a large and deep lake. Show that the fraction f of light that escape directly from water surface is independent of h and is given by
f=[1−√1−1/μ2]2
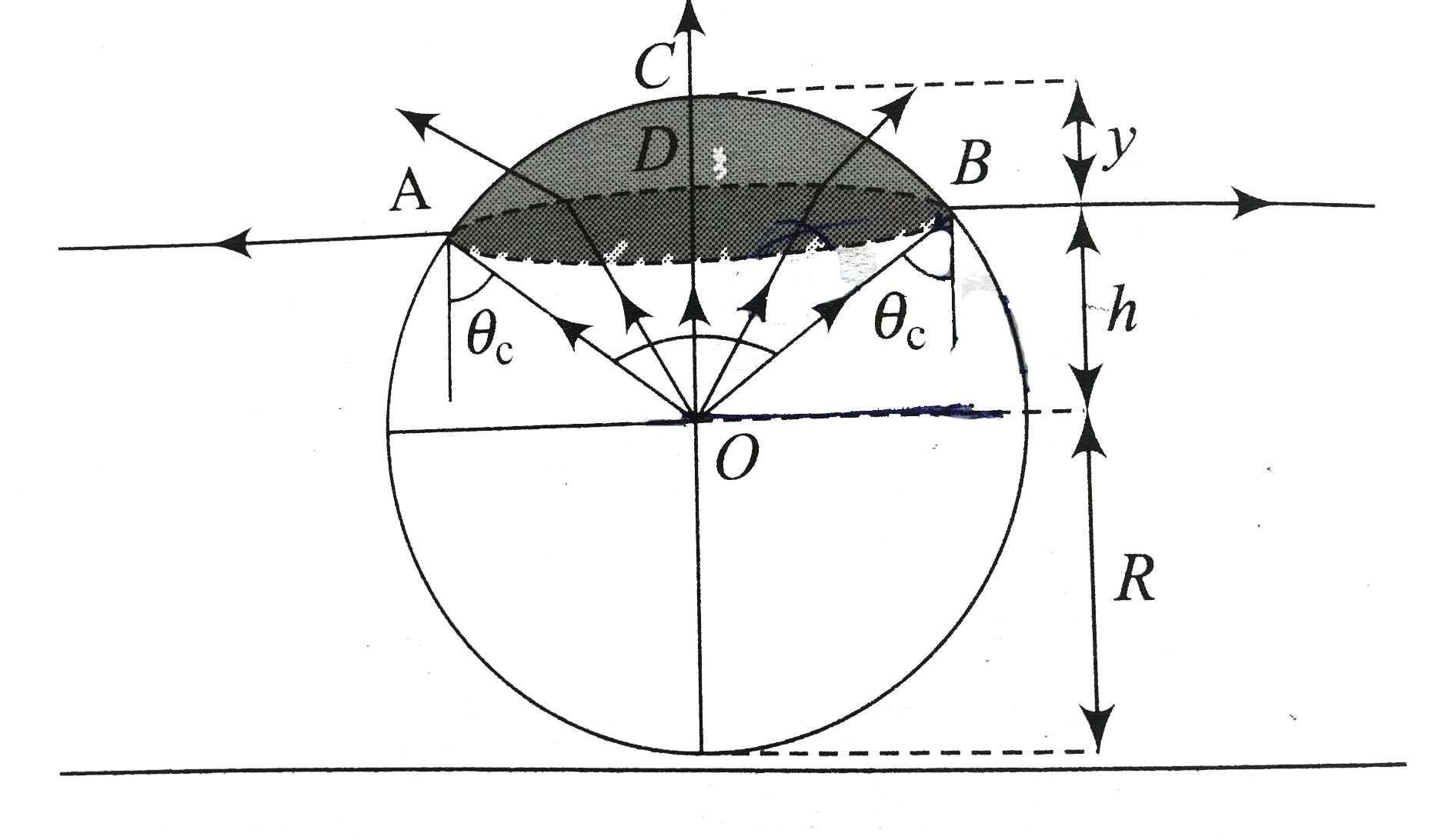
Due to total internal reflection some of the light rays incident at the interface will return back into water. So, only that portion of light will escape for which the angle of incidence at the interface of the medium is less than the critical angle.
If the critical angle is θc, then the light rays that reach beyong the base of the cone whose vertical angle is 2θc will suffer total internal reflection.
Hence, Only the light incident on the base of teh cone refracts and escapes.
Method 2:
The fraction of light escaping,
f= Area of the cap Area of the sphere =2πRy4πR2, i.e.,
f=12[yR]=12[R−hR]
Area of cap ABCD can be calculated by using mathod of integration,
i.e., f=12f=12[1−hR]=12[1−cosθc]
=12[1−√1−sin2θc]
=12[1−√1−1n2]
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Solved Examples15videos
GEOMETRICAL OPTICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Exercise1.114videos
GEOMETRICAL OPTICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSEXERCISE - dpp 3.315videos
ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Thermal Power in Resistance Connected in Circuit27videos
HEATING EFFECT OF CURRENT
Similar Questions
A point source of light is placed at a distance h below the surface of a large deep lake.
(a) Show that the fraction f of the light energy that escapes directly from the water surface is independent of h and is given by f=12−12n√n2−1 where n is the index of refraction of water.
(Note: Absorption within the water and reflection at the surface, except where it is total, have been neglected)
(b) Evaluate this ratio for n=4/3.View SolutionA point source of light is placed at a depth h=0.5 m below the surface of a liquid (μ=54) , Then , the fraction of light energy that escape directly from the liquid surface is
View Solution
Knowledge Check
- Question 1 - Select One
A point source of light is placed at a depth of h below the surface of water of refractive index μ. A floating opaque disc is placed on the surface of water so that light from the source is not visible from the surface. The minimum diameter of the disc is
A2h(μ2−1)1/2B2h(μ2−1)1/2Ch2(μ2−1)1/2Dh(μ2−1)1/2
A point source of light is placed at a distance hbelow the surface of a large deep lake.What is the percentage of light energy that escapes directly form the water surface if μof the water=4/3?(neglet partial reflection)
View SolutionA point source is placed at a depth h below the surface of water (refractive index =μ). The medium above the surface area of water is air from water.
View SolutionA point source of light is placed at a distance of 2f from a converging lens of focal length f. The intensity onteh other side of the lens is maximum at a distance
View SolutionA point source is placed at a depth h below the surface of water (refractive index = mu). (a) Show that light escapes through a circular area on the water surface with its centre directly above the point source. (b) Find the angle subtended by a radius of the area on the source.
View SolutionA point source of light is placed at the bottom of a water lake. If the area of the illuminated circle on the surface is equal to 3 times the square of the depth of the lake. The refractive index of water is
View SolutionAn isotropic point source is placed at a depth h below the water surface. A floating opaque disc is placed on the surface of water so that the source is not visible from the surface. What is the minimum radius of the disc? Take refractive index of water=μ.
View SolutionAn isotropic point source is placed at a depth h below the water surface. A floating opaque disc is placed on the surface of water so that the source is not visible from the surface. What is the minimum radius of the disc? Take refractive index of water=μ.
View Solution
