Consider two perpendicular mirrors M1 and M2 and a point object O. Taking origin at the point of intersection of the mirrros and the coordinates of object as (x,y), find the position and number of images.
To solve the problem of finding the position and number of images formed by two perpendicular mirrors M1 and M2 with a point object O located at coordinates (x,y), we can follow these steps:
Step 1: Determine the Number of Images
The formula to calculate the number of images n formed by two mirrors at an angle θ is given by:
n=360∘θ
In this case, since the mirrors are perpendicular to each other, θ=90∘.
Calculation:
n=360∘90∘=4
Step 2: Apply the Rule for Even n
When n is even, the total number of distinct images formed is given by:
Number of Images=n−1
Thus, for our case:
Number of Images=4−1=3
Step 3: Find the Positions of the Images
To find the positions of the images, we can use the concept of reflection across the mirrors.
1. First Image I1: The first image is formed by reflecting the object O across mirror M1. If the object is at (x,y), the image I1 will be at (x,−y).
2. Second Image I2: The second image is formed by reflecting the object O across mirror M2. The image I2 will be at (−x,y).
3. Third Image I3: The third image is formed by reflecting the first image I1 across mirror M2. The coordinates of I1 are (x,−y), so reflecting this across M2 gives:
I3=(−x,−y)
Summary of Image Positions
- The images formed are:
- I1=(x,−y)
- I2=(−x,y)
- I3=(−x,−y)
Final Result
The total number of images formed is 3, and their positions are:
1. I1=(x,−y)
2. I2=(−x,y)
3. I3=(−x,−y)
The formula to calculate the number of images n formed by two mirrors at an angle θ is given by:
n=360∘θ
In this case, since the mirrors are perpendicular to each other, θ=90∘.
n=360∘90∘=4
When n is even, the total number of distinct images formed is given by:
Number of Images=n−1
Thus, for our case:
Number of Images=4−1=3
To find the positions of the images, we can use the concept of reflection across the mirrors.
I3=(−x,−y)
- The images formed are:
- I1=(x,−y)
- I2=(−x,y)
- I3=(−x,−y)
The total number of images formed is 3, and their positions are:
1. I1=(x,−y)
2. I2=(−x,y)
3. I3=(−x,−y)
Topper's Solved these Questions
- GEOMETRICAL OPTICSBook:CENGAGE PHYSICS ENGLISHChapter:GEOMETRICAL OPTICSExercise:Solved Examples
Explore 15 Videos
- GEOMETRICAL OPTICSBook:CENGAGE PHYSICS ENGLISHChapter:GEOMETRICAL OPTICSExercise:Exercise1.1
Explore 14 Videos
- ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSBook:CENGAGE PHYSICS ENGLISHChapter:ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSExercise:dpp 3.3
Explore 15 Videos
- HEATING EFFECT OF CURRENTBook:CENGAGE PHYSICS ENGLISHChapter:HEATING EFFECT OF CURRENTExercise:Thermal Power in Resistance Connected in Circuit
Explore 27 Videos
Similar Questions
If the straight lines joining the origin and the points of intersection of y=mx+1andx2+y2=1 are perpendicular to each other, then find the value of m.
View SolutionA concave mirror forms an image I corresponding to a point object O . The equation of the circle intercepted by the xy plane on the mirror is
_S01_051_Q01.png)
View SolutionFind the point of intersection of the following pairs of lines: y=m1x+am1 and y=m2x+am2.
View SolutionIf the straight lines joining origin to the points of intersection of the line x+y=1 with the curve x2+y2+x−2y−m=0 are perpendicular to each other , then the value of m should be
View SolutionThe diagram shows a point object P in front of a plane mirror MM1.
In the diagram, mark the position of eye to see the image.
View SolutionFind the image of the point (1,−2) with respect to the line mirror 2x−y+1=0
View SolutionFind the locus of the point of intersection of the lines x=am2andy=2am, where m is a parameter.
View SolutionDraw the graphs of y=x and y=−x in the same graph. Also, find the coordinates of the point where the two lines intersect.
View SolutionDraw the graphs of y=x and y=−x in the same graph. Also, find the coordinates of the point where the two lines intersect.
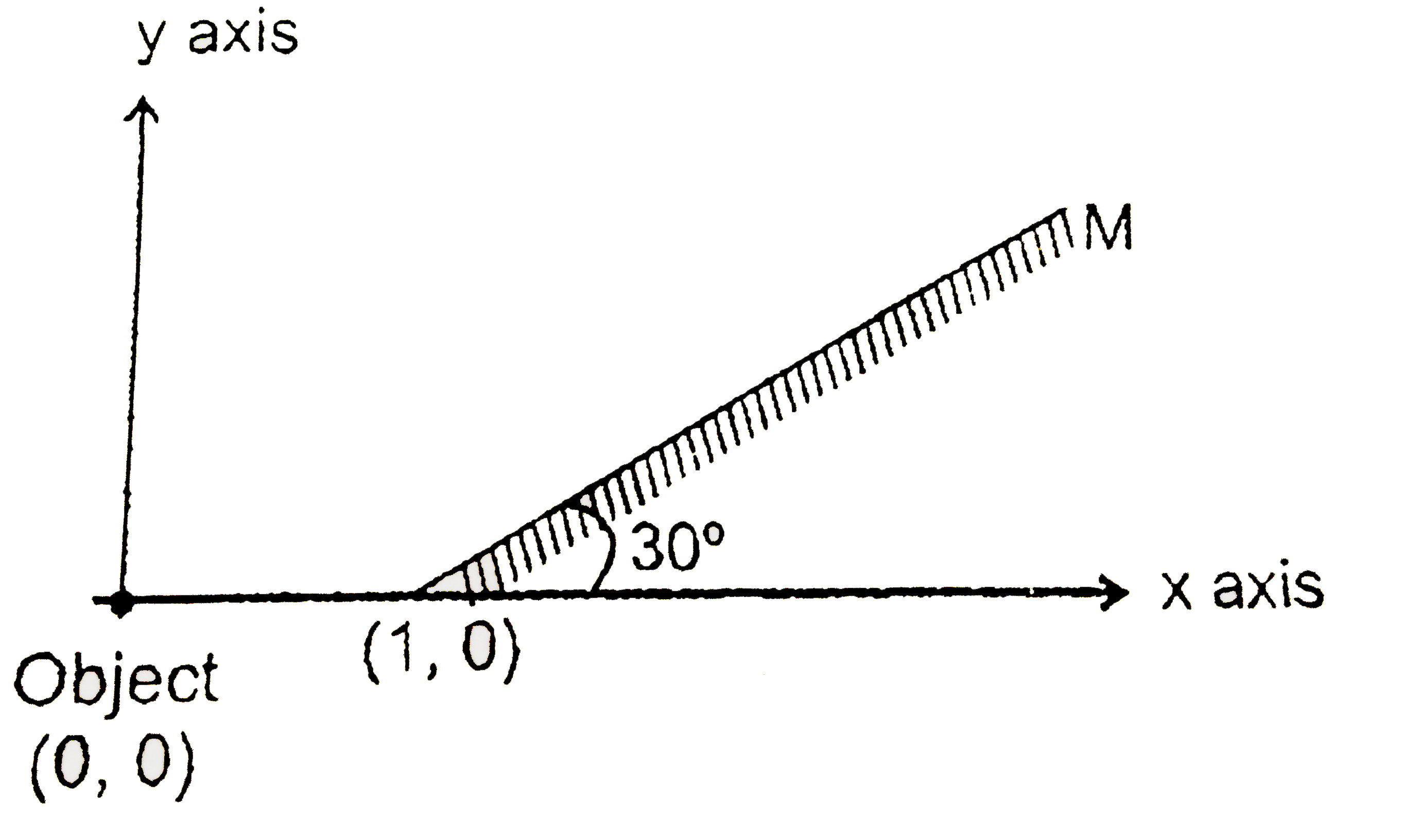
View SolutionA point object is placed (0,0) and a plane mirror 'M' is placed, inclined 30∘ with the x axis.
Find the position of image.
View Solution