When a galvanometer is shunted with a resistance, the deflection is reduced to . If the galvanometer is further shounted with a wire, the new deflection will be (assuming the main current remains the same)
of the deflection when shunted with only
of the deflection when shuntedwith only
of the deflection when shunted with only
of the deflection when shunted with only
The correct Answer is:A
To solve the problem step by step, we need to analyze the situation with the galvanometer and the shunt resistances.
Step 1: Understand the Initial Condition
When the galvanometer is shunted with a 4Ω resistance, the deflection is reduced to 15 of its original deflection. Let's denote:
- \( I_G \): the original current through the galvanometer,
- \( D \): the original deflection,
- \( D' = \frac{D}{5} \): the new deflection after shunting with 4Ω.
Step 2: Determine the Current through the Galvanometer
When the galvanometer is shunted with a 4Ω resistor, the current through the galvanometer can be expressed as:
\(
I_g' = \frac{I_G}{5}
\)
This means that the current flowing through the galvanometer is reduced to \( \frac{4I_G}{5} \) due to the shunt.
Step 3: Calculate the Resistance of the Galvanometer
Using the relationship between the currents and resistances, we can set up the equation:
\(
I_G \cdot R_G = \left(\frac{I_G}{5}\right) \cdot 4
\)
Where \( R_G \) is the resistance of the galvanometer. Simplifying gives:
\(
R_G = 4 \cdot 4 = 16Ω
\)
Step 4: Adding a Second Shunt Resistance
Now, we shunt the galvanometer with an additional 2Ω resistor. We need to find the equivalent resistance of the 4Ω and 2Ω resistors in parallel:
\(
R_{eq} = \frac{R_1 \cdot R_2}{R_1 + R_2} = \frac{4 \cdot 2}{4 + 2} = \frac{8}{6} = \frac{4}{3}Ω
\)
Step 5: Calculate the New Current through the Galvanometer
The total current \( I_G \) will now split between the equivalent resistance \( R_{eq} \) and the galvanometer. Let \( I_g'' \) be the new current through the galvanometer:
\(
I_G = I_g'' + I_{eq}
\)
Using Ohm's law:
\(
I_{eq} = \frac{V}{R_{eq}} = \frac{I_g'' \cdot R_G}{R_G} \cdot \frac{R_{eq}}{R_G + R_{eq}} = \frac{I_g'' \cdot 16}{16 + \frac{4}{3}}
\)
This leads to:
\(
16I_g'' = I_G - I_g''
\)
Solving for \( I_g'' \):
\(
12I_g'' = I_G \implies I_g'' = \frac{I_G}{12}
\)
Step 6: Relate the New Current to the New Deflection
The new deflection \( D'' \) can be expressed as:
\(
D'' = k \cdot I_g'' = k \cdot \frac{I_G}{12}
\)
Where \( k \) is a constant of proportionality. Since the original deflection \( D = k \cdot I_G \), we can express the new deflection as:
\(
D'' = \frac{D}{12}
\)
Step 7: Final Calculation of Deflection
Since the original deflection was reduced to \( \frac{D}{5} \) when shunted with 4Ω, the new deflection when shunted with 2Ω will be:
\(
D'' = \frac{D}{12}
\)
To express this in terms of the original deflection:
\(
D'' = \frac{5}{12}D
\)
Conclusion
Thus, the new deflection when the galvanometer is shunted with a 2Ω wire will be \( \frac{5}{12} \) of the original deflection.
When the galvanometer is shunted with a 4Ω resistance, the deflection is reduced to 15 of its original deflection. Let's denote:
- \( I_G \): the original current through the galvanometer,
- \( D \): the original deflection,
- \( D' = \frac{D}{5} \): the new deflection after shunting with 4Ω.
When the galvanometer is shunted with a 4Ω resistor, the current through the galvanometer can be expressed as:
\(
I_g' = \frac{I_G}{5}
\)
This means that the current flowing through the galvanometer is reduced to \( \frac{4I_G}{5} \) due to the shunt.
Using the relationship between the currents and resistances, we can set up the equation:
\(
I_G \cdot R_G = \left(\frac{I_G}{5}\right) \cdot 4
\)
Where \( R_G \) is the resistance of the galvanometer. Simplifying gives:
\(
R_G = 4 \cdot 4 = 16Ω
\)
Now, we shunt the galvanometer with an additional 2Ω resistor. We need to find the equivalent resistance of the 4Ω and 2Ω resistors in parallel:
\(
R_{eq} = \frac{R_1 \cdot R_2}{R_1 + R_2} = \frac{4 \cdot 2}{4 + 2} = \frac{8}{6} = \frac{4}{3}Ω
\)
The total current \( I_G \) will now split between the equivalent resistance \( R_{eq} \) and the galvanometer. Let \( I_g'' \) be the new current through the galvanometer:
\(
I_G = I_g'' + I_{eq}
\)
Using Ohm's law:
\(
I_{eq} = \frac{V}{R_{eq}} = \frac{I_g'' \cdot R_G}{R_G} \cdot \frac{R_{eq}}{R_G + R_{eq}} = \frac{I_g'' \cdot 16}{16 + \frac{4}{3}}
\)
This leads to:
\(
16I_g'' = I_G - I_g''
\)
Solving for \( I_g'' \):
\(
12I_g'' = I_G \implies I_g'' = \frac{I_G}{12}
\)
The new deflection \( D'' \) can be expressed as:
\(
D'' = k \cdot I_g'' = k \cdot \frac{I_G}{12}
\)
Where \( k \) is a constant of proportionality. Since the original deflection \( D = k \cdot I_G \), we can express the new deflection as:
\(
D'' = \frac{D}{12}
\)
Since the original deflection was reduced to \( \frac{D}{5} \) when shunted with 4Ω, the new deflection when shunted with 2Ω will be:
\(
D'' = \frac{D}{12}
\)
To express this in terms of the original deflection:
\(
D'' = \frac{5}{12}D
\)
Thus, the new deflection when the galvanometer is shunted with a 2Ω wire will be \( \frac{5}{12} \) of the original deflection.
Similar Questions
When a galvanometer is shunted with a 4Ω resistance, the deflection is reduced to one-fifth. If the galvanometer is further shunted with a 2Ω wire, determine current in galvanometer now if initially current in galvanometer is I0 (given main current remains same).
View SolutionTo measure the resistance of a galvanometer by half deflection method, a shunt is connected to the galvanometer . The shunt is
View SolutionWhen a galvanometer is shunted by resistance S, then its current capacity increases n times. If the same galvanometer is shunted by another resistance S', then its current capcity will increase by n' is given by
View SolutionA galvanometer has resistance 1Ω. Maximum deflection current through it is 0.1 A
View SolutionThe deflection in moving coil galvanometer is reduced to half when it is shunted with a 40Ω coil . The resistance of the galvanometer is
View SolutionA galvanometer of resistance 20Ω is shunted by a 2Ω resistor. What part of the main current flows through the galvanometer ?
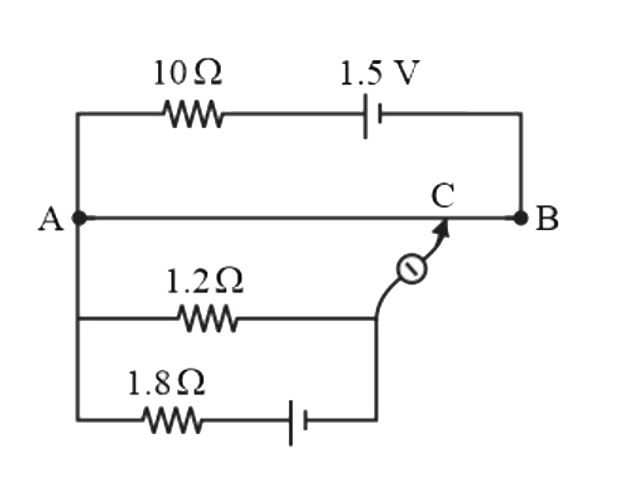
View SolutionIn the below circuit , AB is a wire of length 100 cm with 5Ω resistance . If there is no deflection in the galvanometer , the current flowing in the wire AB is
View SolutionA galvanometer with a resistance of 75Ω produces a full scale deflection with a current of 5 mA. How can this galvanometer be converted into an ammeter which has a range of 0-5A?
View SolutionA galvanometer with a resistance of 75Ω produces a full scale deflection with a current of 5 mA. How can this galvanometer be converted into an ammeter which has a range of 0-5A?
View SolutionResistance of a Galvanometer coil is 8Ω and 2Ω Shunt resistance is connected with it. If main current is 1 A then the current flow through 2Ω resistance will be :
View Solution
