Resistance of a Galvanometer coil is 8Ω and 2Ω Shunt resistance is connected with it. If main current is 1 A then the current flow through 2Ω resistance will be :
0.2 A
0.8 A
0.1 A
0.4 A
The correct Answer is:B
To solve the problem, we will follow these steps:
Step 1: Understand the Circuit Configuration
The galvanometer and the shunt resistor are connected in parallel. The total current (I) entering the parallel combination is given as 1 A.
Step 2: Define Variables
Let:
- I1 = current through the galvanometer (8 Ω)
- I2 = current through the shunt resistor (2 Ω)
- Total current I=I1+I2=1A
Step 3: Apply Ohm's Law
Since the galvanometer and the shunt are in parallel, the voltage across both must be the same. Using Ohm's law (V = I × R), we can express the voltages as:
- Voltage across the galvanometer: V=I1×8
- Voltage across the shunt: V=I2×2
Setting these equal gives us:
I1×8=I2×2
Step 4: Rearrange the Equation
From the equation I1×8=I2×2, we can express I2 in terms of I1:
I2=4×I1
Step 5: Substitute into Total Current Equation
Now substitute I2 back into the total current equation:
I=I1+I2
1=I1+4×I1
1=5×I1
Step 6: Solve for I1
Now, solve for I1:
I1=15=0.2A
Step 7: Calculate I2
Now substitute I1 back into the equation for I2:
I2=4×I1=4×0.2=0.8A
Conclusion
The current flowing through the 2 Ω shunt resistance is 0.8A.
---
The galvanometer and the shunt resistor are connected in parallel. The total current (I) entering the parallel combination is given as 1 A.
Let:
- I1 = current through the galvanometer (8 Ω)
- I2 = current through the shunt resistor (2 Ω)
- Total current I=I1+I2=1A
Since the galvanometer and the shunt are in parallel, the voltage across both must be the same. Using Ohm's law (V = I × R), we can express the voltages as:
- Voltage across the galvanometer: V=I1×8
- Voltage across the shunt: V=I2×2
I1×8=I2×2
From the equation I1×8=I2×2, we can express I2 in terms of I1:
I2=4×I1
Now substitute I2 back into the total current equation:
I=I1+I2
1=I1+4×I1
1=5×I1
Now, solve for I1:
I1=15=0.2A
Now substitute I1 back into the equation for I2:
I2=4×I1=4×0.2=0.8A
The current flowing through the 2 Ω shunt resistance is 0.8A.
Similar Questions
Knowledge Check
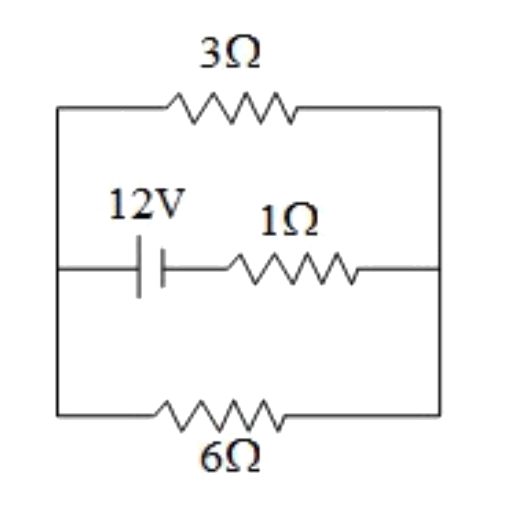
In the shown figure, bridge is balanced, the current flowing through 2Ω resistance is
 A108AB117AC137AD87A
A108AB117AC137AD87A
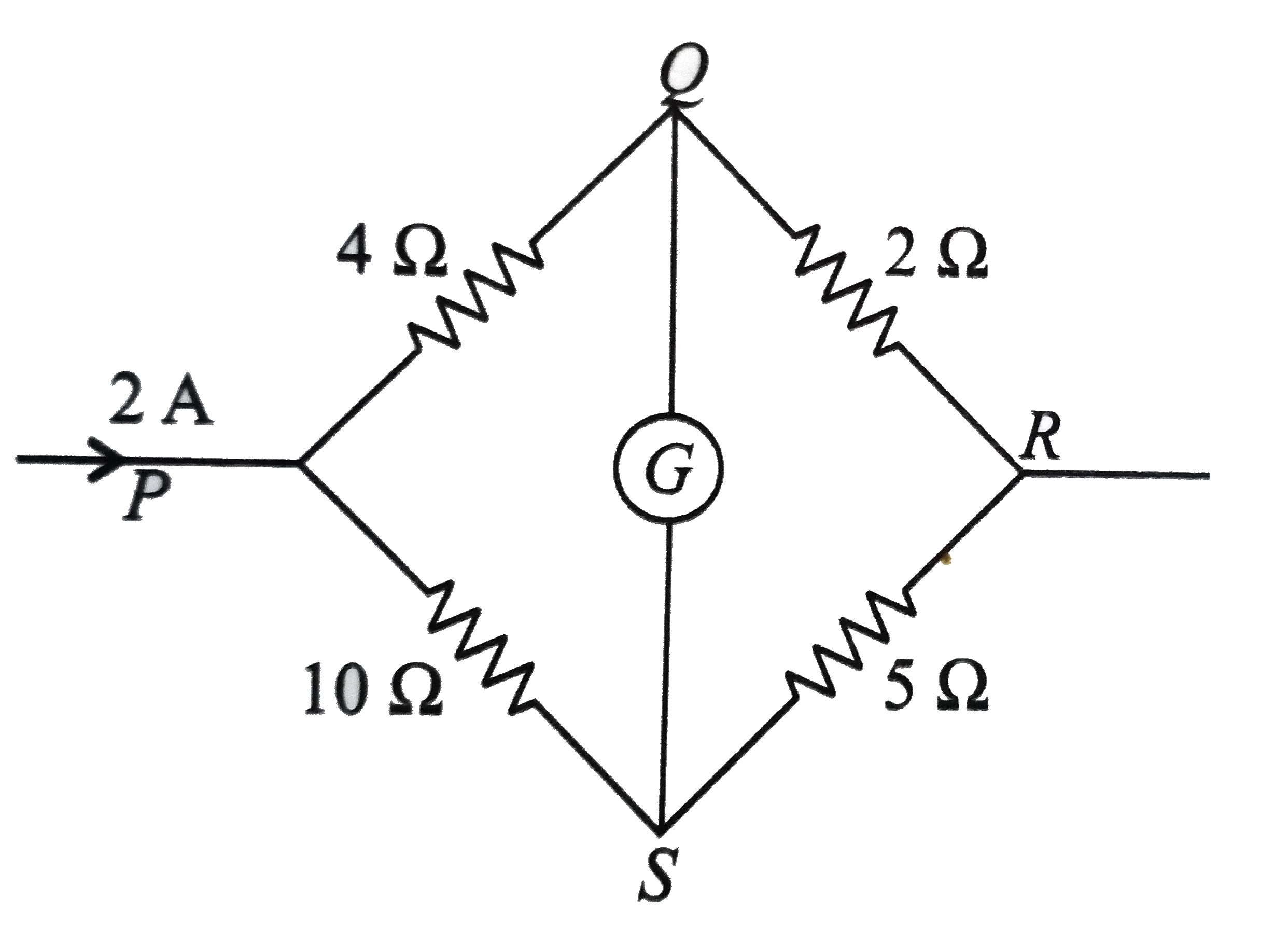
In the circuit, the current flowing through 10Ω resistance is
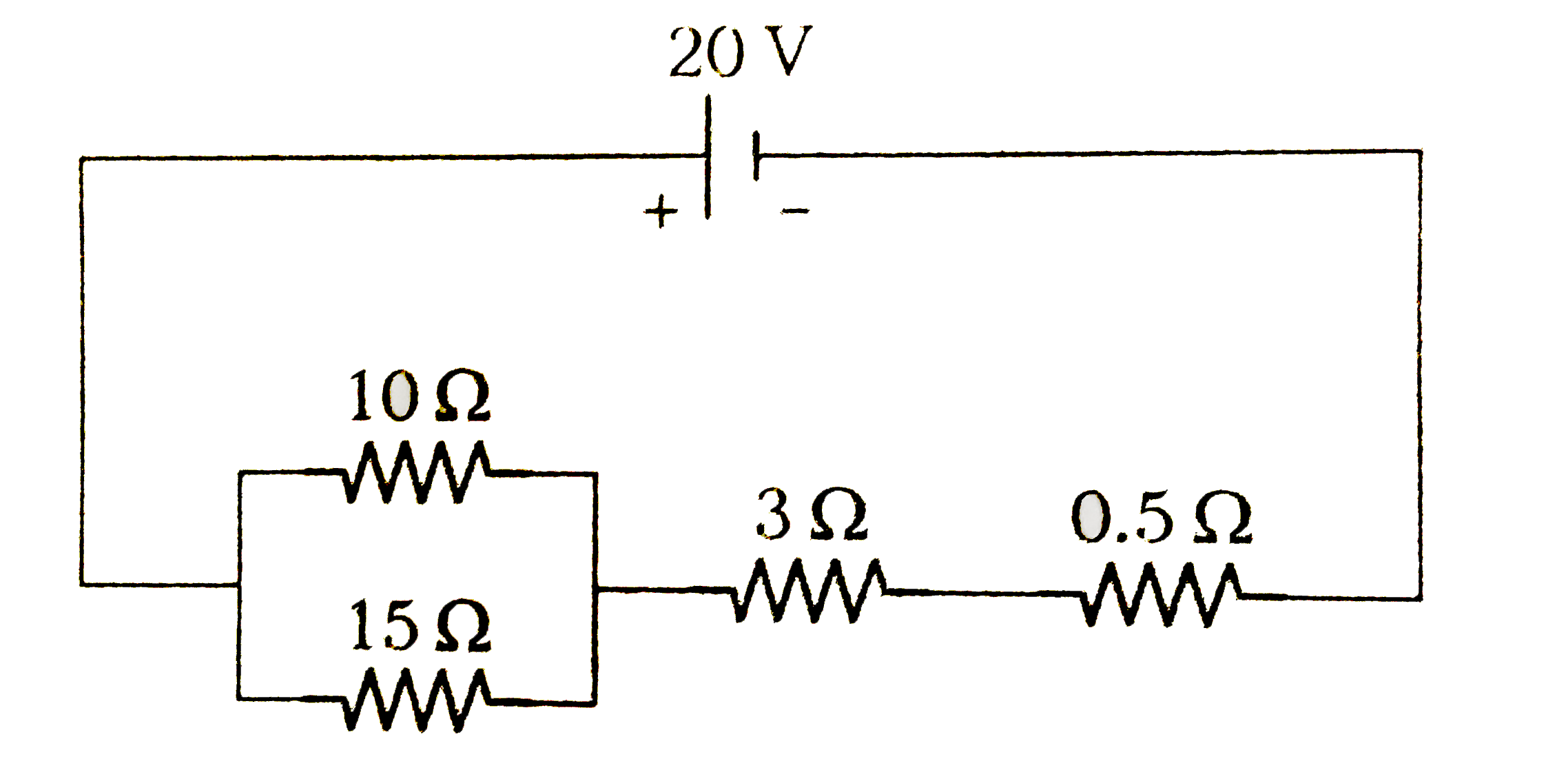
View SolutionIn the given circuit current flowing through 2 Omega resistance is
View SolutionCalculate the current flows through resistance 2Ω.
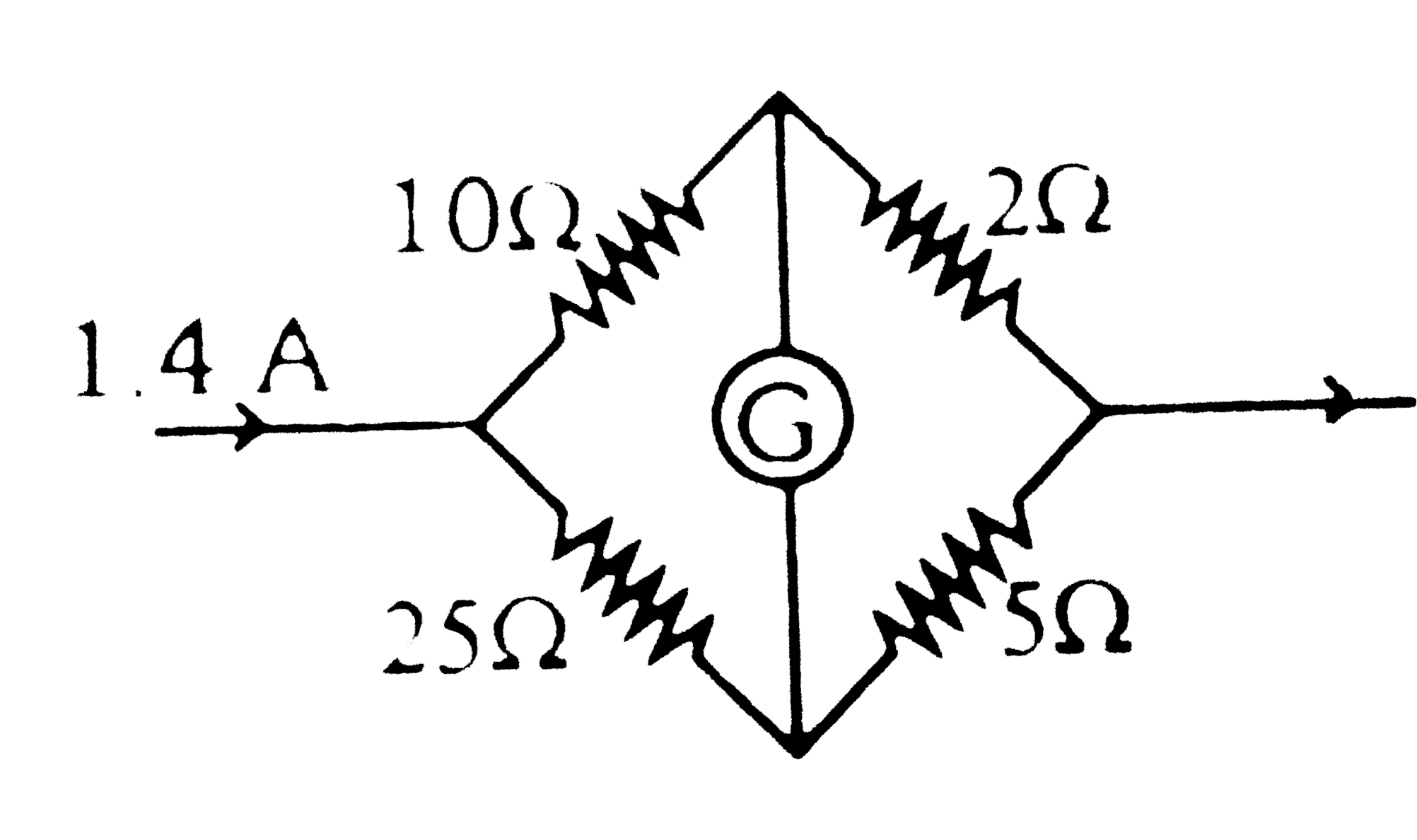
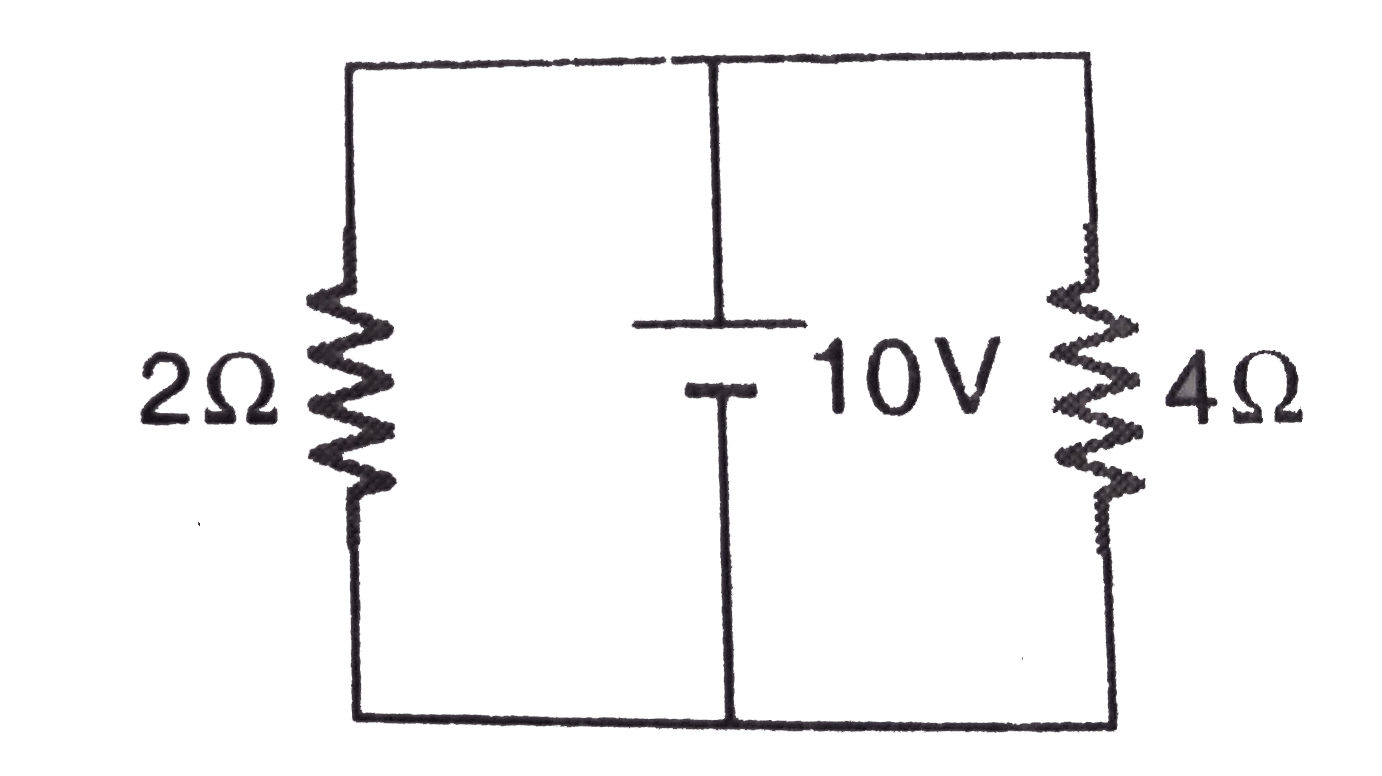
View SolutionFind the current through 2Ω and 4Ω resistance.
View SolutionThe resistance of galvanometer is 999Ω. A shunt of 1Ω is connected to it. If the main current is 10−2A, what is the current flowing through the galvanometer.
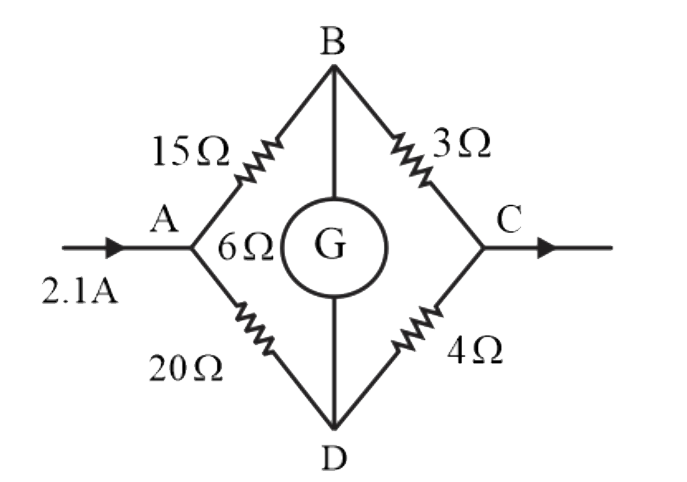
View SolutionIn the following network, the current flowing through 15Ω resistance is
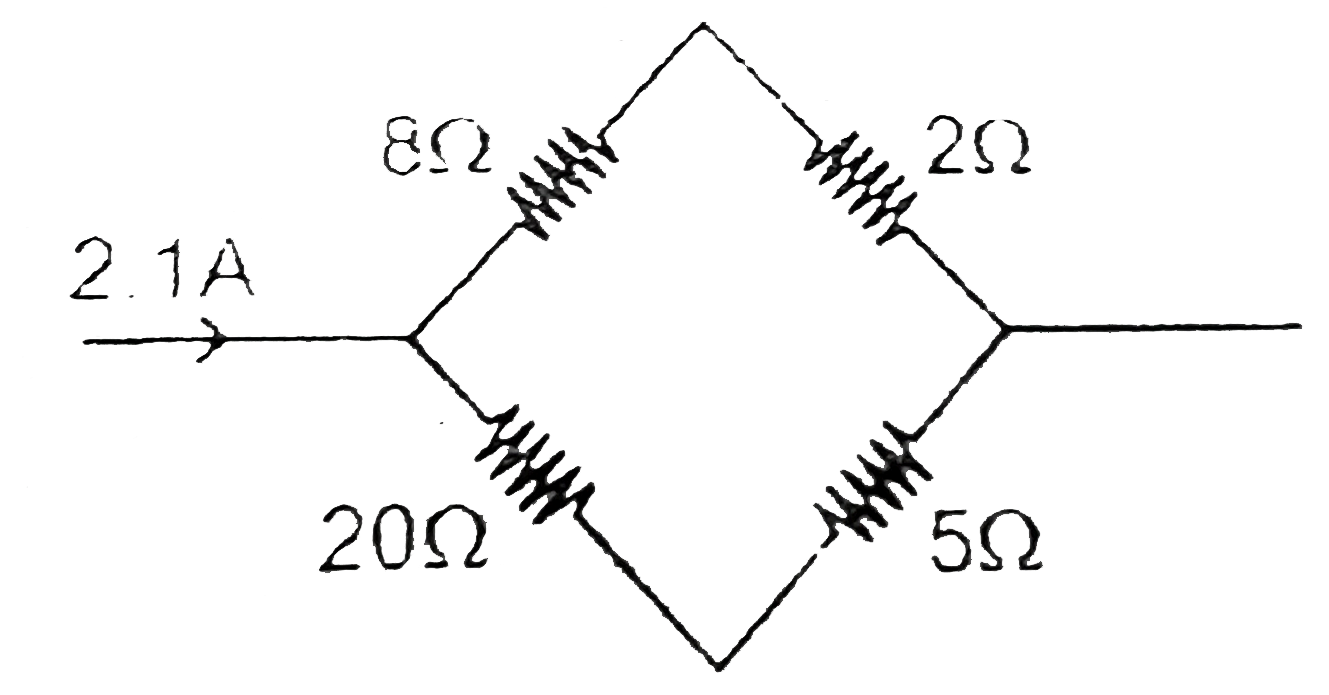
View SolutionIn the circuit in figure, the current flowing 5Ω resistance is :
View Solution