Two point charges are located on the x - axis : q1=−e at x=0 and q2=+e at x=a. A third charge q3=+e is brought to x=2a.
The total potential energy of the system of three charges.
e24πε0a
e28πε0a
−e28πε0a
−e24πε0a
The correct Answer is:C
To find the total potential energy of the system of three charges, we will follow these steps:
Step 1: Identify the Charges and Their Positions
We have three charges:
- q1=−e located at x=0
- q2=+e located at x=a
- q3=+e located at x=2a
Step 2: Write the Formula for Potential Energy
The potential energy U between two point charges q1 and q2 separated by a distance r is given by:
U=kq1q2r
where k is Coulomb's constant, k=14πϵ0.
Step 3: Calculate the Potential Energy Between Each Pair of Charges
1. Potential Energy between q1 and q2:
- Distance r12=a
- U12=k(−e)(e)a=−ke2a
2. Potential Energy between q2 and q3:
- Distance r23=a
- U23=k(e)(e)a=ke2a
3. Potential Energy between q1 and q3:
- Distance r13=2a
- U13=k(−e)(e)2a=−ke22a
Step 4: Sum the Potential Energies
Now, we can find the total potential energy Utotal of the system by summing the potential energies calculated above:
Utotal=U12+U23+U13
Substituting the values we calculated:
Utotal=(−ke2a)+(ke2a)+(−ke22a)
Step 5: Simplify the Expression
Notice that −ke2a and ke2a cancel each other out:
Utotal=0−ke22a=−ke22a
Step 6: Substitute the Value of k
Now substitute k=14πϵ0:
Utotal=−12a⋅e24πϵ0=−e28πϵ0a
Final Answer
Thus, the total potential energy of the system of three charges is:
Utotal=−e28πϵ0a
We have three charges:
- q1=−e located at x=0
- q2=+e located at x=a
- q3=+e located at x=2a
The potential energy U between two point charges q1 and q2 separated by a distance r is given by:
U=kq1q2r
where k is Coulomb's constant, k=14πϵ0.
1. Potential Energy between q1 and q2:
- Distance r12=a
- U12=k(−e)(e)a=−ke2a
- Distance r23=a
- U23=k(e)(e)a=ke2a
- Distance r13=2a
- U13=k(−e)(e)2a=−ke22a
Now, we can find the total potential energy Utotal of the system by summing the potential energies calculated above:
Utotal=U12+U23+U13
Substituting the values we calculated:
Utotal=(−ke2a)+(ke2a)+(−ke22a)
Notice that −ke2a and ke2a cancel each other out:
Utotal=0−ke22a=−ke22a
Now substitute k=14πϵ0:
Utotal=−12a⋅e24πϵ0=−e28πϵ0a
Thus, the total potential energy of the system of three charges is:
Utotal=−e28πϵ0a
Topper's Solved these Questions
- ELECTRIC POTENTIALBook:CENGAGE PHYSICS ENGLISHChapter:ELECTRIC POTENTIALExercise:Integer
Explore 5 Videos
- ELECTRIC POTENTIALBook:CENGAGE PHYSICS ENGLISHChapter:ELECTRIC POTENTIALExercise:DPP 3.1
Explore 14 Videos
- ELECTRIC POTENTIALBook:CENGAGE PHYSICS ENGLISHChapter:ELECTRIC POTENTIALExercise:Multiple Correct
Explore 10 Videos
- ELECTRIC FLUX AND GAUSS LAWBook:CENGAGE PHYSICS ENGLISHChapter:ELECTRIC FLUX AND GAUSS LAWExercise:MCQ s
Explore 38 Videos
- ELECTRICAL MEASURING INSTRUMENTSBook:CENGAGE PHYSICS ENGLISHChapter:ELECTRICAL MEASURING INSTRUMENTSExercise:M.C.Q
Explore 2 Videos
Similar Questions
Two point charges are located on the x - axis : q1=−e at x=0 and q2=+e at x=a.
The work done by an external force to bring a third point charge q3=+e from infinity to x=2a is.
View SolutionA point charge q1=4.00nC is placed at the origin, and a second point charge q2=−3.00nC, placed on the x-axis at x = + 20.0 cm. A third point charge q3=2.00nC is placed on the X-axis between q1,andq2. (Take as zero the potential energy of the three charges when they are infinitely far apart).
(a) What is the potential energy of the system of the three charges if q3 is placed at x= + 10.0 cm?
(b) Where should q3 be placed to make the potential energy of the system equal to zero?
View SolutionTwo points charges are located on the x-axis, q1=−1μC at x=0andq2=+1μc at x=1m.
a. Find the work that must be done by an external force to bring a third point charge q3=+1μC from infinity to x=2m.
b. Find the total potential energy of the system of three charges.
View SolutionTwo points charges are located on the x-axis, q1=−1μC at x=0andq2=+1μc at x=1m.
a. Find the work that must be done by an external force to bring a third point charge q3=+1μC from infinity to x=2m.
b. Find the total potential energy of the system of three charges.
View SolutionThree charges −q,+Q and −q are placed in a straight line as shown

If the total potential energy of the system is zero, then the ratio qQ is
View SolutionTwo equal charges q are placed at a distance of 2a and a third charge −2q is placed at the midpoint. The potential energy of the system is
View SolutionThe charges - q, Q, -q are placed along x-axis at x = 0, x = a and x = 2a. If the potential energy of the system is zero, then Q:q is
View SolutionTwo point charges q each are fixed at (a,0) and (-a,0). A third charge Q is placed at origin. Electrons potential energy of the system will
View SolutionCharges−q,Q,and−q are placed at an equal distance on a straight liner. If the total potential energy of the system of three charges is zero, then find the ratio Q/q.
 .
.
View SolutionThree charges are placed along x-axis at x = - a x = 0 and x = a as shown in the figure. The potential energy of the system is
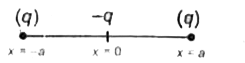
View Solution