A solid sphere is given an angular velocity ω and kept on a rough fixed incline plane. Then choose the correct statement.
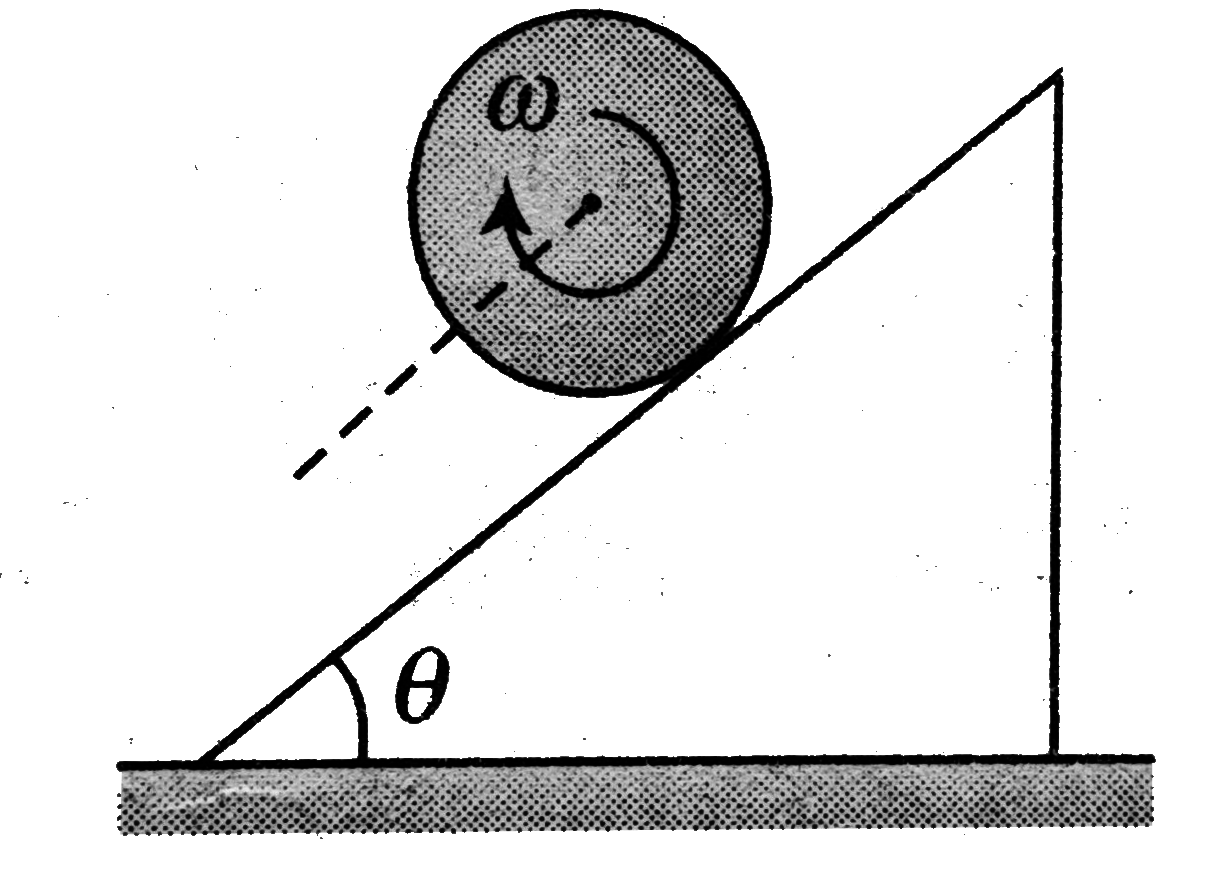
If μ=tanθ, then the sphere will be in linear equilibrium for some time and after that pure rolling down the plane will start
If μ=tanθ, then sphere will move up the plane and frictional force acting all the time will be 2mgsinρ.
If μ=tanθ/2 there will never be pure rolling (consider inclined plane to be long enough).
If the incline plane is not fixed at it is on the smooth horizontal surface, then the linear momentum of the system (wedge and sphere) can be conserved in the horizontal direction.
The correct Answer is:A, D
Due to the torque of friction about CM ζ eventually decreases to zero, initially there is no translation. Friction is sufficient for pure rolling, therfore after sometime pure rolling begins. There is no external force in x direction, therefore momentum is conserved along x direction.
Topper's Solved these Questions
- MISCELLANEOUS VOLUME 2Book:CENGAGE PHYSICS ENGLISHChapter:MISCELLANEOUS VOLUME 2Exercise:LC_TYPE
Explore 34 Videos
- MISCELLANEOUS VOLUME 2Book:CENGAGE PHYSICS ENGLISHChapter:MISCELLANEOUS VOLUME 2Exercise:INTEGER_TYPE
Explore 10 Videos
- MISCELLANEOUS VOLUME 2Book:CENGAGE PHYSICS ENGLISHChapter:MISCELLANEOUS VOLUME 2Exercise:INTEGER_TYPE
Explore 10 Videos
- MISCELLANEOUS KINEMATICSBook:CENGAGE PHYSICS ENGLISHChapter:MISCELLANEOUS KINEMATICSExercise:Interger type
Explore 3 Videos
- NEWTON'S LAWS OF MOTION 1Book:CENGAGE PHYSICS ENGLISHChapter:NEWTON'S LAWS OF MOTION 1Exercise:Integer
Explore 5 Videos
Similar Questions
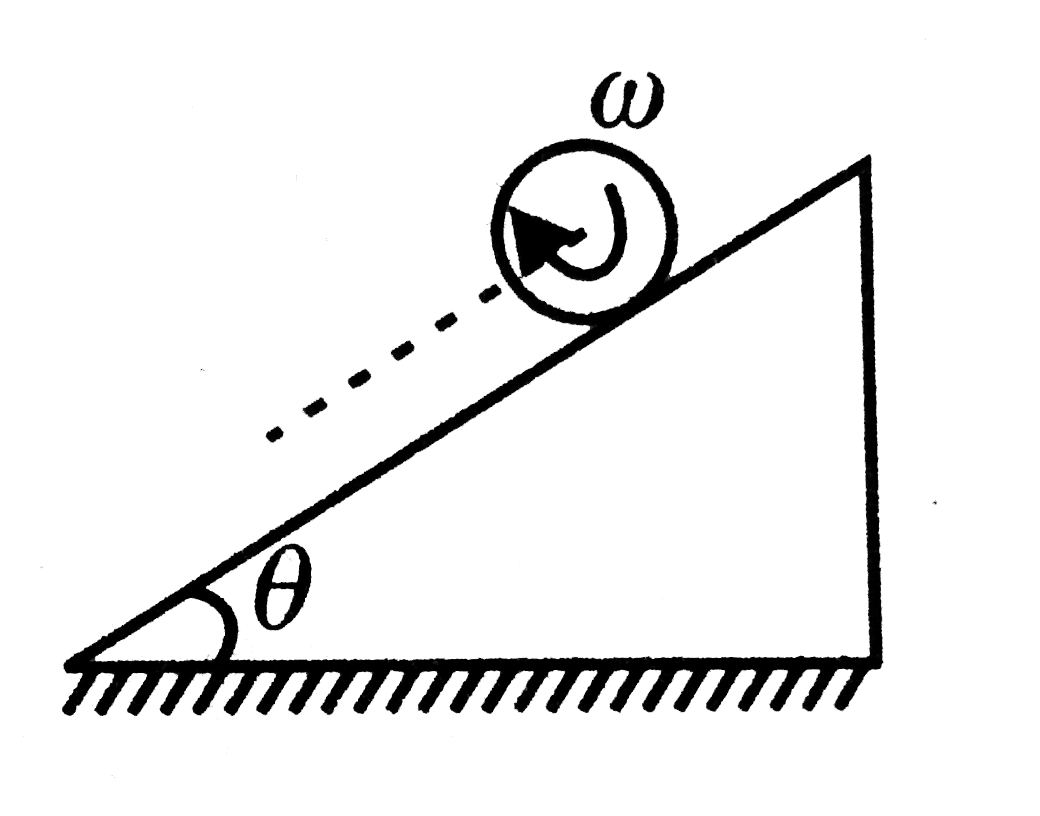
A solid sphere is given an angular velocity ω and kept on a rough fixed incline plane. The choose the correct statement.
View SolutionChoose the correct statement w. r.t. RuBisCO
View SolutionA wheel of mass M and radius a and M.I. IG (about centre of mass) is set rolling with angular velocity ω up a rough inclined plane of inclination theta. The distance traveled by it up the plane is
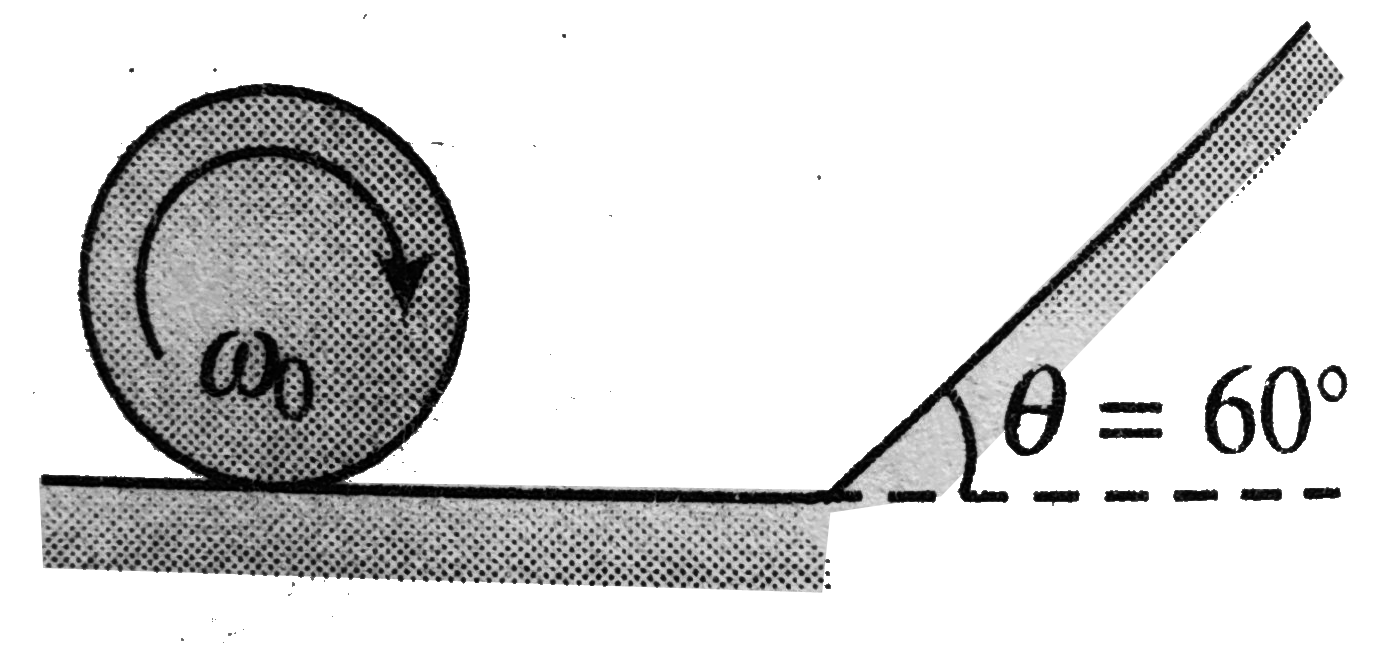
View SolutionA uniform solid sphere of radius R, rolling without sliding on a horizontal surface with an angular velocity ω0, meets a rough inclined plane of inclination θ=60∘. The sphere starts pure rolling up the plane with an angular velocity ω Find the value of ω.
View SolutionA disc of radius R is moving on a rough horizontal surface with velocity v and angular speed ω at an instant as shown in figure. Choose the correct statement.

View SolutionA disc is rotated about its axis with a certain angular velocity and lowered gently on a rough inclined plane as shown in Fig., then

View SolutionA disc is rotated about its axis with a certain angular velocity and lowered gently on a rough inclined plane as shown in Fig., then

View SolutionA solid cylinder is given a velocity v0 and an angular speed ω0 in the anticlockwise direction and then it is placed gently on a very long rough plank ( initially at rest ) as shown in the figure. Then, choose the incorrect statement :
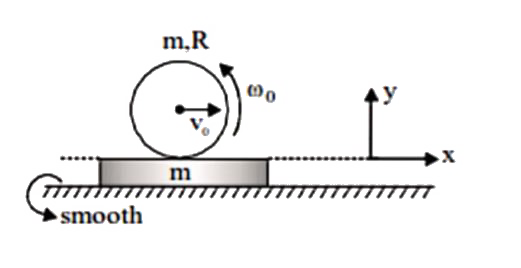
View SolutionA disc is given an initial angular velocity ω0 and placed on a rough horizontal surface as shown Fig. The quantities which will not depend on the coefficient of friction is/are
View SolutionA disc is given an angular velocity ω0 and a linear velocity v0 as shown in the figure. It is released on a rough horizontal surface of friction coefficient μ. Mark the correct statement (ω0=3v0/R)

View Solution