A man of mass m walks from end A to the other end B of a boat of mass M and length l. The coefficient of friction between the man and the boat is μ an neglect any resistive force between the boat and the water.
(a)If the man runs at his maximum acceleration, the acceleration of the boat is (m/M)μg.
(b)The minimum time take by the man to reach the other end of the boat is √2MI(M+m)μg
(c)If man runs at his maximum acceleration the acceleration of boat is mm+Mμg
(d)The minimum time take by the man to reach the other end of the boat is √2ml(M+m)μg
The correct Answer is:A, B
To solve the problem of a man walking from one end of a boat to the other, we need to analyze the forces and accelerations involved. Here's a step-by-step breakdown of the solution:
Step 1: Understand the system
- We have a man with mass m walking on a boat with mass M and length l.
- The coefficient of friction between the man and the boat is μ.
- We will neglect any resistive forces between the boat and the water.
Step 2: Determine the maximum acceleration of the man
- The maximum force of friction that can act on the man as he walks is given by:
Ffriction=μ⋅m⋅g
- This frictional force provides the maximum acceleration am of the man:
am=Ffrictionm=μ⋅m⋅gm=μ⋅g
Step 3: Determine the acceleration of the boat
- According to Newton's third law, the man walking forward will exert a backward force on the boat. The acceleration ab of the boat can be derived from the same frictional force:
Ffriction=M⋅ab
- Thus, the acceleration of the boat is:
ab=FfrictionM=μ⋅m⋅gM
Step 4: Determine the relative acceleration
- The relative acceleration arelative between the man and the boat is given by:
arelative=am+ab=μg+μmgM
- This can be simplified to:
arelative=μg(1+mM)
Step 5: Calculate the time taken to reach the other end of the boat
- The distance S that the man needs to cover is the length of the boat l.
- Using the equation of motion, S=12arelativet2, we can solve for time t:
l=12arelativet2
t2=2larelative
t=
⎷2lμg(1+mM)
Conclusion
- The maximum acceleration of the man is μg.
- The maximum acceleration of the boat is μmgM.
- The time taken by the man to reach the other end of the boat is
⎷2lμg(1+mM).
- We have a man with mass m walking on a boat with mass M and length l.
- The coefficient of friction between the man and the boat is μ.
- We will neglect any resistive forces between the boat and the water.
- The maximum force of friction that can act on the man as he walks is given by:
Ffriction=μ⋅m⋅g
- This frictional force provides the maximum acceleration am of the man:
am=Ffrictionm=μ⋅m⋅gm=μ⋅g
- According to Newton's third law, the man walking forward will exert a backward force on the boat. The acceleration ab of the boat can be derived from the same frictional force:
Ffriction=M⋅ab
- Thus, the acceleration of the boat is:
ab=FfrictionM=μ⋅m⋅gM
- The relative acceleration arelative between the man and the boat is given by:
arelative=am+ab=μg+μmgM
- This can be simplified to:
arelative=μg(1+mM)
- The distance S that the man needs to cover is the length of the boat l.
- Using the equation of motion, S=12arelativet2, we can solve for time t:
l=12arelativet2
t2=2larelative
t=
⎷2lμg(1+mM)
- The maximum acceleration of the man is μg.
- The maximum acceleration of the boat is μmgM.
- The time taken by the man to reach the other end of the boat is
⎷2lμg(1+mM).
Topper's Solved these Questions
- MISCELLANEOUS VOLUME 2Book:CENGAGE PHYSICS ENGLISHChapter:MISCELLANEOUS VOLUME 2Exercise:LC_TYPE
Explore 34 Videos
- MISCELLANEOUS VOLUME 2Book:CENGAGE PHYSICS ENGLISHChapter:MISCELLANEOUS VOLUME 2Exercise:INTEGER_TYPE
Explore 10 Videos
- MISCELLANEOUS VOLUME 2Book:CENGAGE PHYSICS ENGLISHChapter:MISCELLANEOUS VOLUME 2Exercise:INTEGER_TYPE
Explore 10 Videos
- MISCELLANEOUS KINEMATICSBook:CENGAGE PHYSICS ENGLISHChapter:MISCELLANEOUS KINEMATICSExercise:Interger type
Explore 3 Videos
- NEWTON'S LAWS OF MOTION 1Book:CENGAGE PHYSICS ENGLISHChapter:NEWTON'S LAWS OF MOTION 1Exercise:Integer
Explore 5 Videos
Similar Questions
A man pulls a block of mass equal to himself with a light string .The coefficient of friction between the man and the floor is greater than that between the block and the floor
View SolutionA man of mass m runs without sliding from rest from one end of a boat of mass M and length l with an acceleration a relative to the boat. If the friction between water and boat is neglected find the

a. acceleration of CM of the system (M+m)
b. acceleration of the man and boat
c. position of man at the time when he reaches to other end of the boat
d. frictional force
e. work done by friction on boat.
f. total work done by friction.
g. velocities of man and boat when the man reaches other end of the boat.
h. work down by man
View SolutionA small block of mass m is projected on a larger block of mass 10m and length l with a velocity v as shown in the figure. The coefficient of friction between the two block is μ2 while that between the lower block and the ground is μ1. Given that μ2>11μ1.
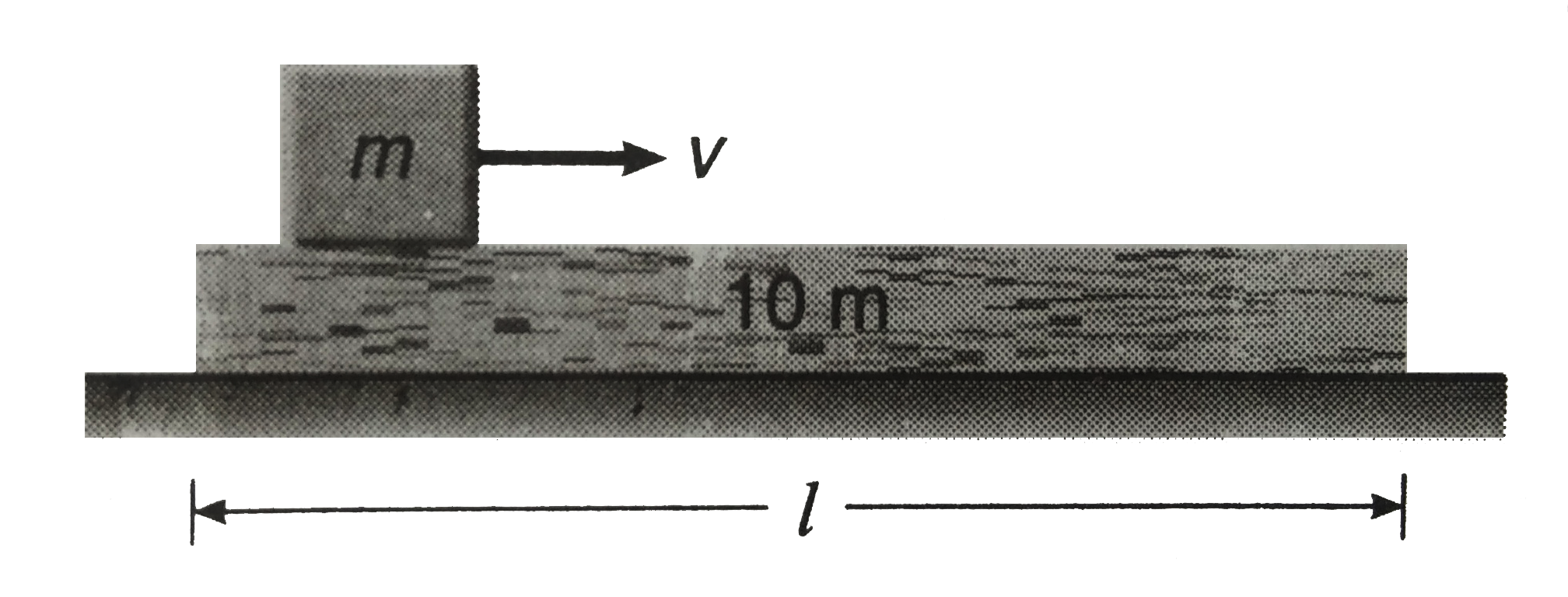
(a) Find the minimum value of v, such that the mass m falls off the block of mass 10m .
(b) If v has minimum value, find the time taken by block m to do so.
View SolutionA boy having a mass of 40kg stands at one end A of a boat of length 2m at rest. The boy walks to the other end B of the boat and stops. What is the distance moved by the boat? Friciton exists between the feet of the boy and the surface of the boat. But the friction between the boat and the water surface may be neglected. Mass of the boat is 15kg.

View SolutionAn insect of mass m is initially at one end of a stick of length L and mass M, which rests on a smooth floor. The coefficientg of friction between the insect and the stick is k. The minimum time in which the insect can reach the other end of the stick is t. Then t2 is equal to
View Solution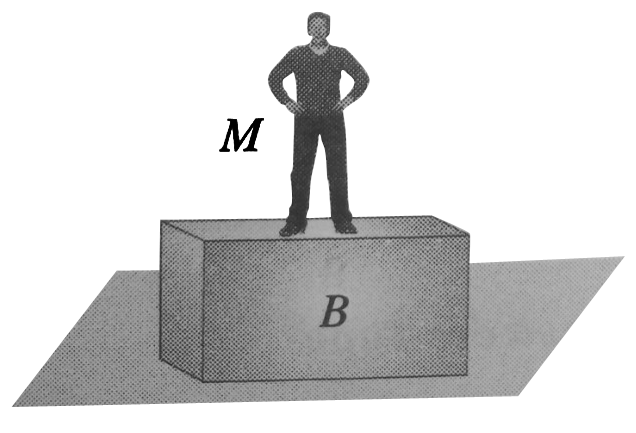
As shown in the figure, M is a man of mass 60 kg standing on a block of mass 40 kg kept on ground. The co-efficient of friction between the feet of the man and the block is 0.3 and that between B and the ground is 0.1. If the man accelerates with an acceleration 2ms2 in the forward direction, then,
View SolutionA block of mass m is stationary on a rough inclined plane making an angle θ with the horizontal. Find the contact force between block and the plane
View SolutionA man of mass 70 kg is standing at one end of a stationary, floating barge of mass 210 kg. he then walks to the other end of the barge, a distance of 90 meters. Ignore any frictional effect between the barge and the water.
Q. If the man walks at an average velocity of 8m/s what is the average velocity of the barge?
View SolutionA man of mass 70 kg is standing at one end of a stationary, floating barge of mass 210 kg. he then walks to the other end of the barge, a distance of 90 meters. Ignore any frictional effect between the barge and the water.
Q. How far will the barge move?
View SolutionA block of mass m is placed behind in contact with vertical side of M as shown in the figure. The coefficient of static friction between m and M in μ. The least horizontal force with which m can be pushed so that the two blocks move together is (neglect friction between M and ground).
View Solution