Statement I: Imagine holding two identical bricks under water. Brick A is completely submerged just below the surface of water, while brick B is at a greater depth. The magnitude of force exerted by the person (on the brick) to hold brick B in place is the same as magnitude of force. exerted by the person (on the brick) to hold brick A in place.
Statement II: The magnitude of buoyant force on a brick completely submerged in water is equal to the magnitude of weight of water it displaced and does not depend on the depth of the brick in water.
Statement I is true, statement II is true and Statement II is a correct explanation for Statement I.
Statement I is true, Statement II is true and Statement II is NOT the correct explanation for Statement I.
Statement I is true, Statement II is false.
Statement I is false, Statement II is true.
The correct Answer is:A
To solve the question, we need to analyze both statements and apply the principles of fluid mechanics, particularly Archimedes' principle.
Step 1: Understand the scenario
We have two identical bricks, A and B. Brick A is submerged just below the surface of the water, while brick B is at a greater depth. We need to determine if the forces exerted by a person to hold each brick in place are the same.
Hint: Consider the definitions of buoyant force and how it relates to the weight of the displaced fluid.
Step 2: Apply Archimedes' Principle
According to Archimedes' principle, the buoyant force acting on a submerged object is equal to the weight of the fluid displaced by the object. Since both bricks are identical and fully submerged, they displace the same volume of water, leading to the same buoyant force acting on both bricks.
Hint: Remember that the buoyant force depends only on the volume of fluid displaced, not on the depth of the object.
Step 3: Analyze the forces acting on the bricks
For each brick, the person must exert a force to counteract the buoyant force in order to hold the brick in place. Since the buoyant force is the same for both bricks (as established in Step 2), the force exerted by the person to hold each brick must also be the same.
Hint: Think about the equilibrium of forces acting on each brick when submerged.
Step 4: Evaluate Statement I
Statement I claims that the magnitude of the force exerted by the person to hold brick B is the same as that for brick A. Since we established that the buoyant forces are equal, this statement is true.
Hint: Compare the forces acting on both bricks and their equilibrium conditions.
Step 5: Evaluate Statement II
Statement II states that the magnitude of the buoyant force on a brick completely submerged in water is equal to the weight of the water it displaces and does not depend on the depth of the brick in water. This is also true, as buoyant force is determined solely by the volume of fluid displaced.
Hint: Recall that buoyant force is independent of depth for fully submerged objects.
Conclusion
Both statements are true, and Statement II correctly explains Statement I. Therefore, the correct answer is that both statements are true, and Statement II is the correct explanation for Statement I.
Final Answer: Both statements are true, and Statement II is the correct explanation of Statement I.
We have two identical bricks, A and B. Brick A is submerged just below the surface of the water, while brick B is at a greater depth. We need to determine if the forces exerted by a person to hold each brick in place are the same.
According to Archimedes' principle, the buoyant force acting on a submerged object is equal to the weight of the fluid displaced by the object. Since both bricks are identical and fully submerged, they displace the same volume of water, leading to the same buoyant force acting on both bricks.
For each brick, the person must exert a force to counteract the buoyant force in order to hold the brick in place. Since the buoyant force is the same for both bricks (as established in Step 2), the force exerted by the person to hold each brick must also be the same.
Statement I claims that the magnitude of the force exerted by the person to hold brick B is the same as that for brick A. Since we established that the buoyant forces are equal, this statement is true.
Statement II states that the magnitude of the buoyant force on a brick completely submerged in water is equal to the weight of the water it displaces and does not depend on the depth of the brick in water. This is also true, as buoyant force is determined solely by the volume of fluid displaced.
Both statements are true, and Statement II correctly explains Statement I. Therefore, the correct answer is that both statements are true, and Statement II is the correct explanation for Statement I.
Topper's Solved these Questions
- FLUID MECHANICSBook:CENGAGE PHYSICS ENGLISHChapter:FLUID MECHANICSExercise:Assertion-Reasoning
Explore 8 Videos
- FLUID MECHANICSBook:CENGAGE PHYSICS ENGLISHChapter:FLUID MECHANICSExercise:Linked Comprehension
Explore 43 Videos
- FLUID MECHANICSBook:CENGAGE PHYSICS ENGLISHChapter:FLUID MECHANICSExercise:Single Correct
Explore 95 Videos
- DIMENSIONS & MEASUREMENTBook:CENGAGE PHYSICS ENGLISHChapter:DIMENSIONS & MEASUREMENTExercise:Integer
Explore 2 Videos
- GRAVITATIONBook:CENGAGE PHYSICS ENGLISHChapter:GRAVITATIONExercise:INTEGER_TYPE
Explore 1 Video
Similar Questions
Assertion: Pascal law is the working principle of hydraulic lift.
Reason: Pressure =thrustarea
View SolutionA small spherical ball of radius r is released from its completely submerged Position (as shown in the figure) in a liquid whose density varies with height h (measured from the bottom) as ρL=ρ0[4−(3h/h0)].The density of the ball is (5/2)ρ0. The height of the vessel is h0=12/π2 Consider r<<h0 and g=10m/s2.
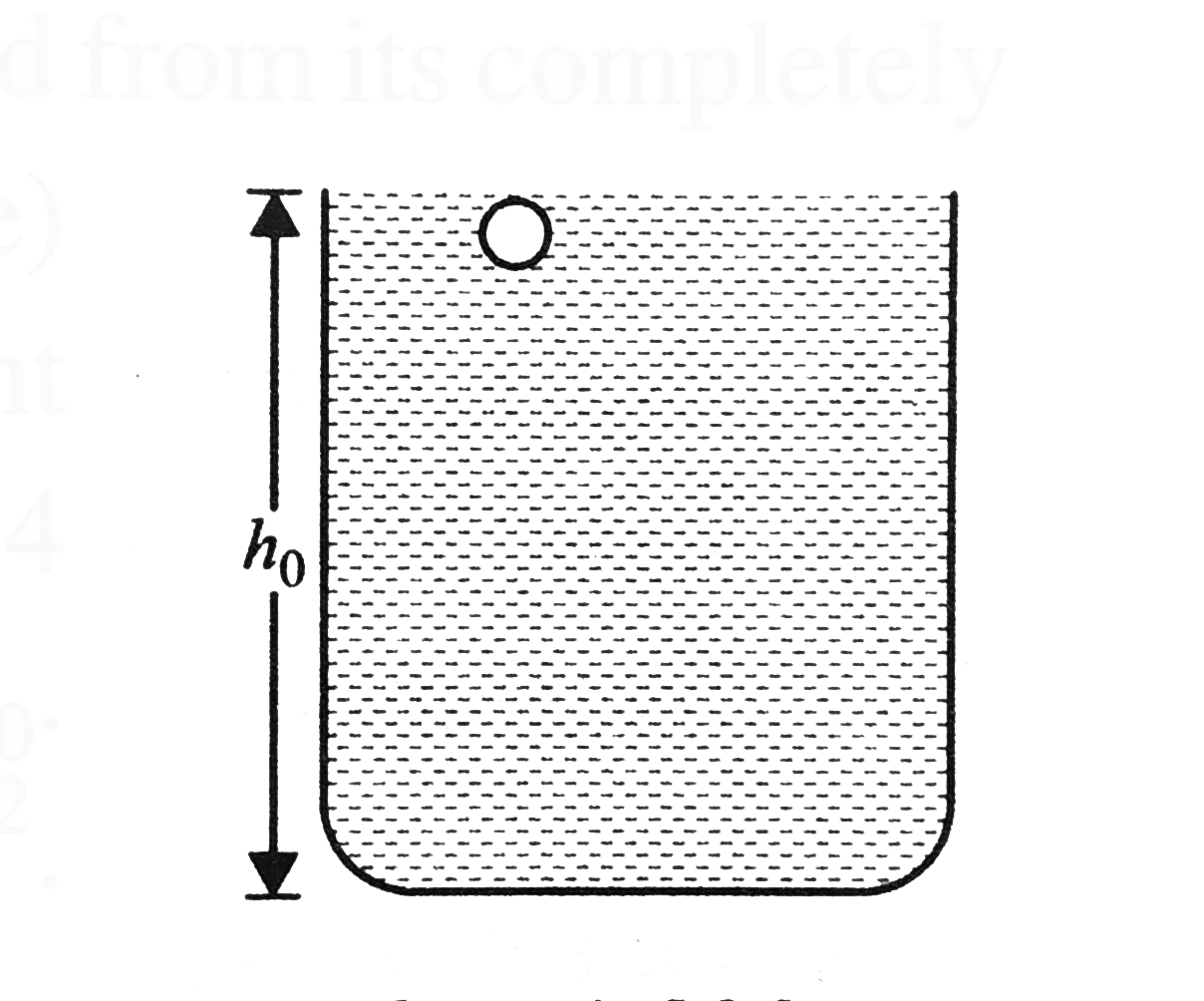
The motion of the ball in the vessel is
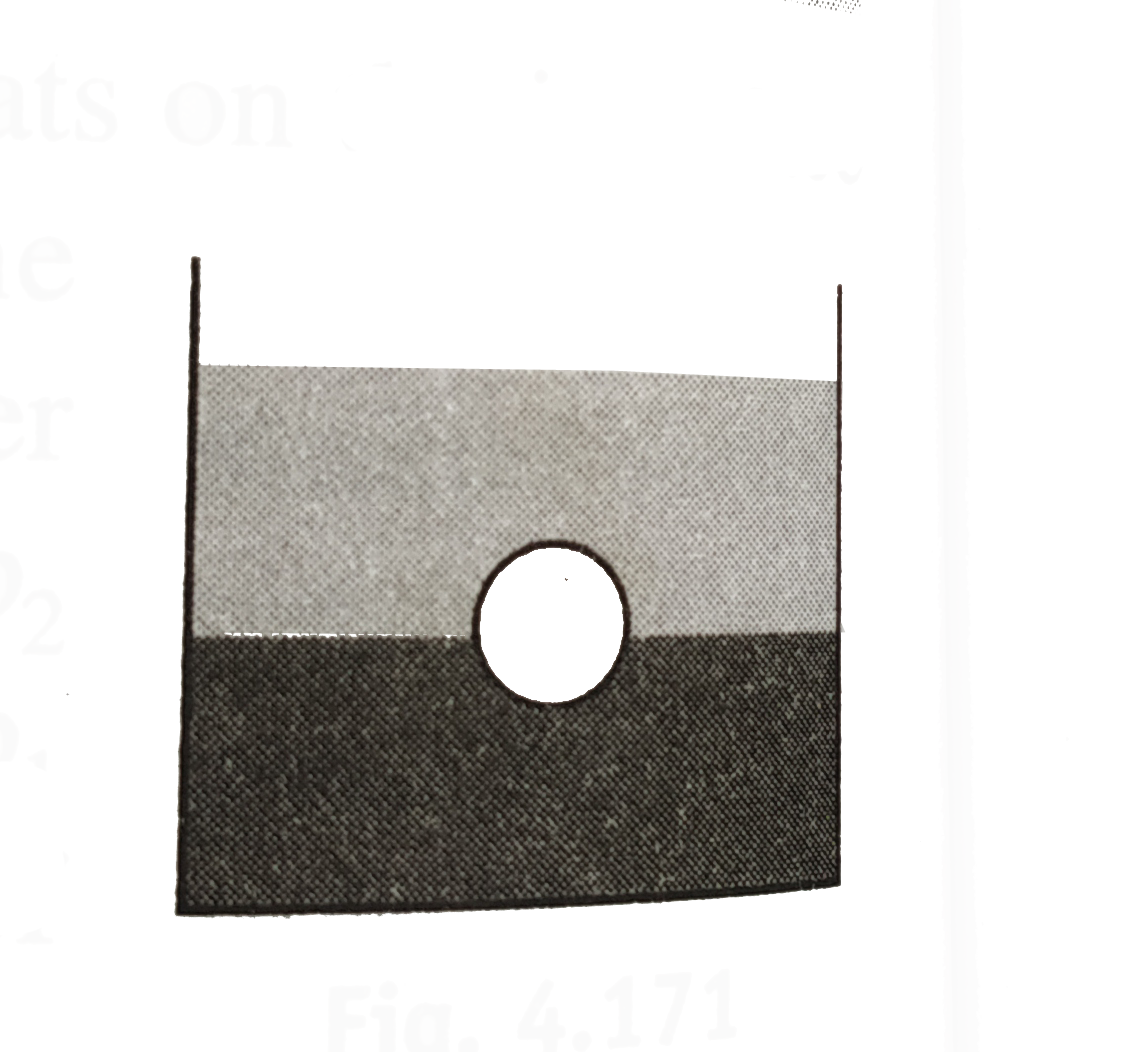
View SolutionA solid uniform ball of volume V floats on the interface of two immiscible liquids (see the figure). The specific gravity of the upper liquid is ρ1 and that of lower one is ρ2 and the specific gravity of ball is ρ(ρ1>ρ>ρ2) The fraction of the volume the ball in the u of upper liquid is
View SolutionTo find the distance d over which a signal can be seen clearly in foggy conditions, a railways-engineer uses dimensions and assumes that the distance depends on the mass density ρ of the fog, intensity (power/area) S of the light from the signal and its frequency f. the engineer finds that d is proportional to S1/n. the value of n is
View SolutionGravitational acceleration on the surface of a planet is √611g. where g is the gravitational acceleration on the surface of the earth. The average mass density of the planet is 23 times that of the earth. If the escape speed on the surface of the earht is taken to be 11kms−1 the escape speed on the surface of the planet in kms−1 will be
View SolutionStatement I: Imagine holding two identical bricks under water. Brick A is completely submerged just below the surface of water, while brick B is at a greater depth. The magnitude of force exerted by the person (on the brick) to hold brick B in place is the same as magnitude of force. exerted by the person (on the brick) to hold brick A in place. Statement II: The magnitude of buoyant force on a brick completely submerged in water is equal to the magnitude of weight of water it displaced and does not depend on the depth of the brick in water.
View SolutionA brick of length L is placed on the horizontal floor. The bricks of same length and size are placed on this brick, one above the other by providing a margin of L8 from the edge of the brick placed just below, in the same direction.
Find the correct option.
View SolutionA brick stands on a box having 60 cm length, 40 cm breadth and 20 cm width. Pressure exerted by the brick will be:
View SolutionA cistern , internally measuring 150cm×120m×110cn has 129600cm3 of water in it .porous bricks are placed in the water until the cistern is full to the brim . Each brick absorbs one - seventeenth of its own volume of water . How many bricks can be put in without overflowing the water , each brick being 22.5cm×7.5cm×6.5cm ?
View SolutionThe volume of brick is 2.197L. The submerged brick is balanced by a 2.54 kg mass on the beam scale. The weight of the brick is
View SolutionFour identical bricks are kept one over another in such a way that a part of each brick is projected out of the brick below it. For equilibrium of the system, how much of the three upper bricks can be extended out?
View SolutionA brick stands on a box having 60 cm length, 40 cm breadth and 20 cm width. Pressure exerted by the brick will be:
View SolutionA boat with bricks is floating on the water. When the bricks are thrown into water, the level of water in the pond:
View Solutionईंट से ईंट बजाना मुहावरे का अर्थ है-
View Solution'ईंट से ईंट बजाना' मुहावरे का अर्थ है
View Solution