One quarter sector is cut from a uniform circular disc of radius $R$ . This sector has mass $M$ . It is made to rotate about a line perpendicular to its plane and passing through the centre of the original disc. It moment of inertia about the axis of rotation is.
.

A

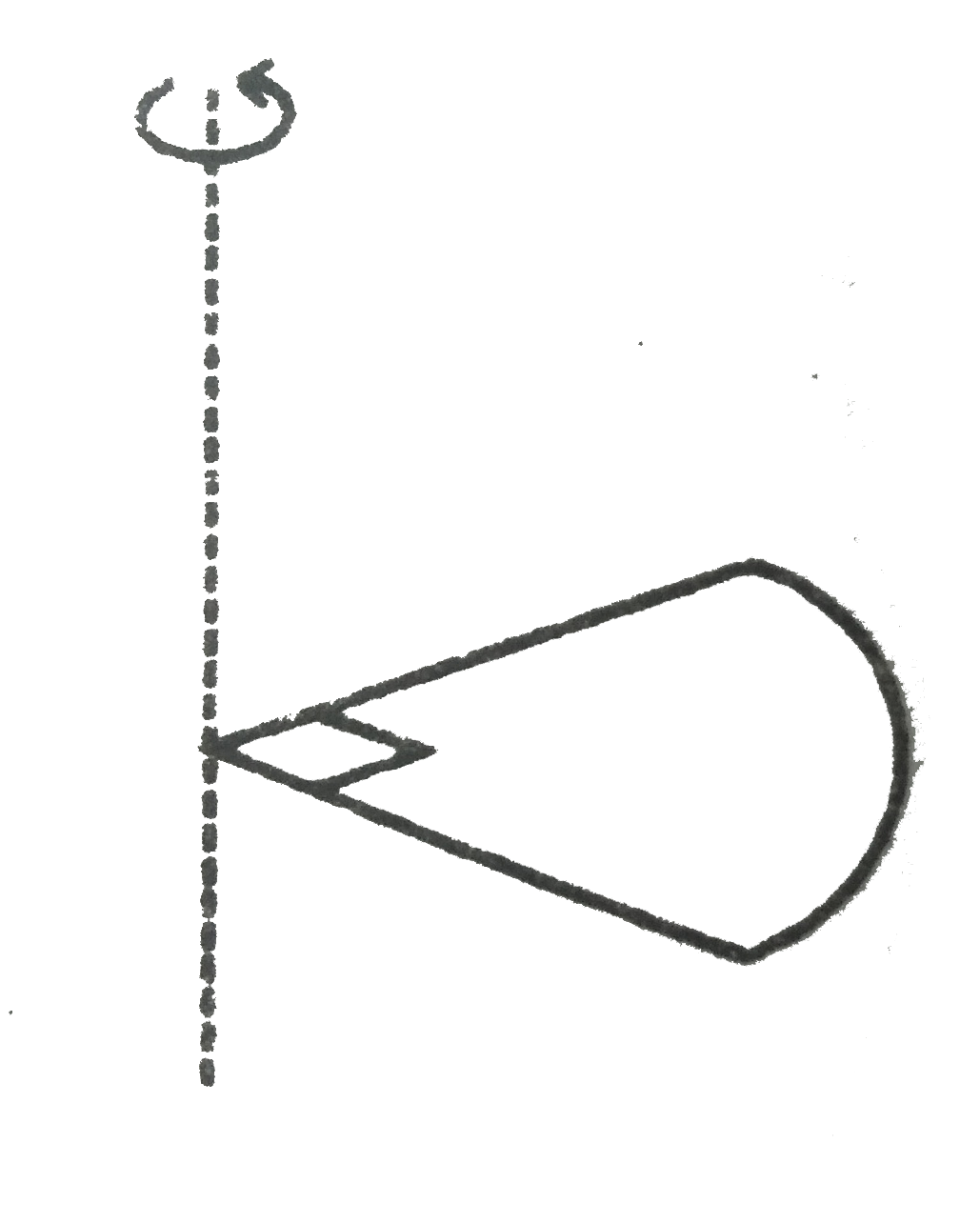$\frac{1}{2} M R^{2}$

B

$\frac{1}{4} M R^{2}$

C

$\frac{1}{8} M R^{2}$

D

$\sqrt{2} M R^{2}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

We cannot consider the quadrant as a single mass as the distance of different particles is different form the axis of rotation. So we take the help of calculus. Let us consider a segment as shown in figure. All masses lying in this segment are at a distance $r$ form the axis and hence considered as a small differential mass $d m$ . Let te thickness of the segment be $d r$ .

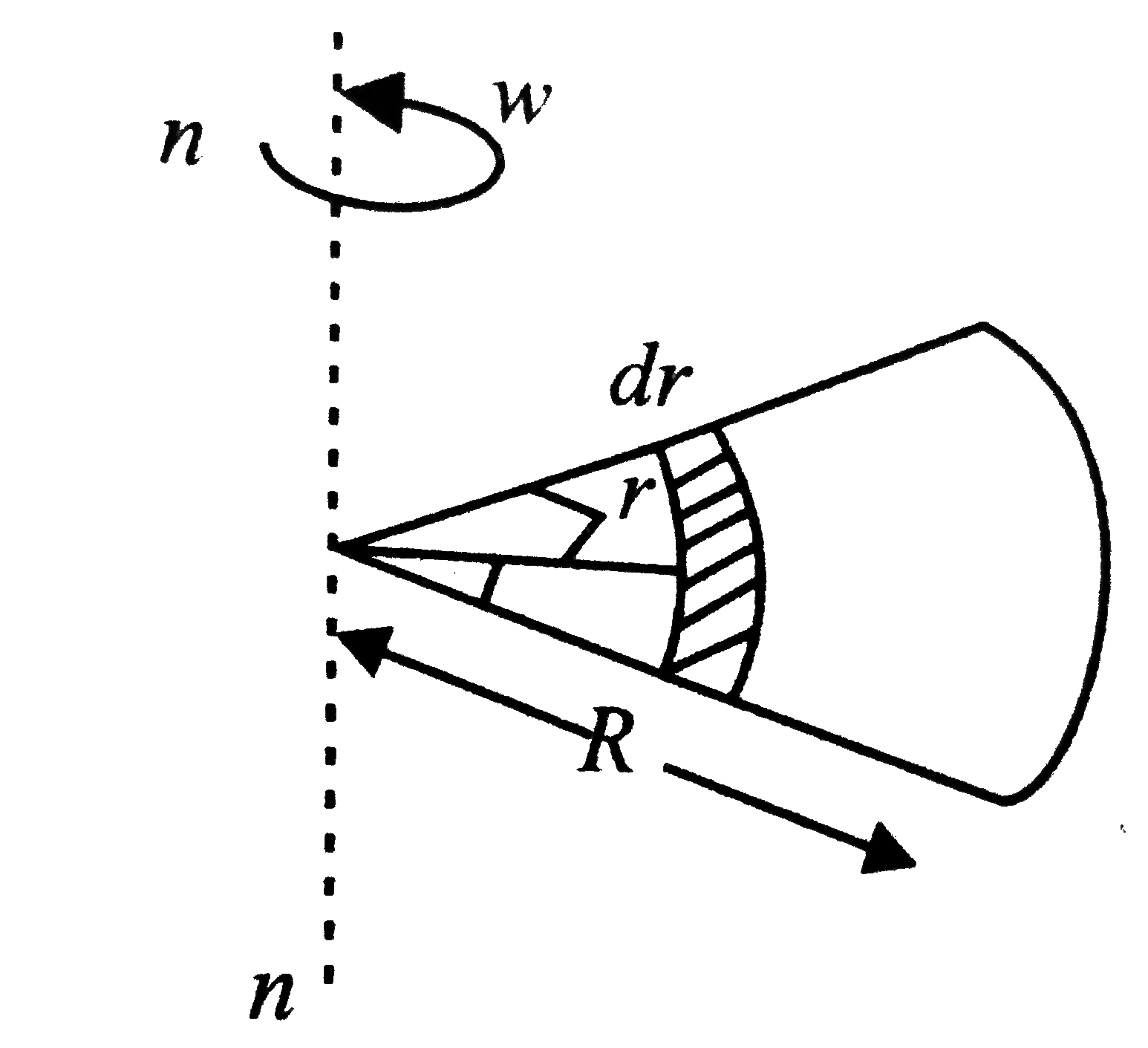
The mass per unit of the quadrant $= \frac{M}{π R^{2} / 4} = \frac{4 M}{π R^{2}}$
Area of the segment $= \frac{1}{4} [π {(r + d r)}^{2} - π r^{2}] = \frac{1}{4} \times 2 π r d r = \frac{π r d r}{2}$
Mass of the segment $d m = \frac{π r d r}{2} \times \frac{4 M}{π R^{2}} = \frac{2 M}{R^{2}} r^{2} r d r$
$M I$ of the segment about $nn' = \frac{2 M}{R^{2}} r d r x r^{2} \frac{2 M}{R^{2}} r^{3} d r$
$M I$ of the quadrant about $nn' = \int_{0}^{R} \frac{2 M}{R^{2}} r^{2} d r$
$\frac{2 M}{R^{2}} \times \frac{R^{4}}{4} \times \frac{M R^{2}}{2}$

Updated on:21/07/2023

|

Class 11 PHYSICS RIGID BODY DYNAMICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

One quarter sector is cut from a uniform circular disc of radius R. The sector has mass M. IT is made to rotate about a line perpendicular to its plane and passing through the centre of the original disc. Its moment of inertia about the axis of rotation is
A $M R^{2} / 2$
B $M R^{2} / 4$
C $M R^{2} / 8$
D $\sqrt{2} M R^{2}$

CENGAGE PHYSICS ENGLISH-RIGID BODY DYNAMICS 2-SCQ_TYPE

Home
Ask Doubt
Profile