The uniform speed of a body is the same as seen from any point in the body. A light cord is wrapped around the rim of the disc and mass of 1kg is tied to the free end. If it is released from rest,
the tension in the cord is 5N
in the first 4s the angular displacement of the disc is 40rad
the work done by the torque on the disc in the first 4s is 200J
the increase in kinetic energy of the disc in the first 4s is 200J
The correct Answer is:A, B, C, D
To solve the problem step by step, we will analyze the forces acting on the system and apply the relevant equations of motion for both linear and rotational dynamics.
Step 1: Analyze the Forces
When the mass is released, it experiences two forces:
1. The gravitational force (weight) acting downward, Fg=mg.
2. The tension in the cord acting upward, T.
For the mass m=1kg:
- Weight, Fg=mg=1×9.81=9.81N.
Step 2: Apply Newton's Second Law
Using Newton's second law for the mass, we can write:
mg−T=ma
Where a is the linear acceleration of the mass.
Step 3: Relate Linear Acceleration to Angular Acceleration
The linear acceleration a of the mass is related to the angular acceleration α of the disc by the equation:
a=rα
Where r is the radius of the disc.
Step 4: Calculate Torque on the Disc
The torque τ acting on the disc due to the tension in the cord is given by:
τ=T⋅r
The moment of inertia I for a disc about its center is:
I=12mdiscr2
Assuming the mass of the disc is 2kg.
Step 5: Apply Newton's Second Law for Rotation
Using Newton's second law for rotation:
τ=Iα
Substituting the expressions for torque and moment of inertia:
T⋅r=12mdiscr2α
Step 6: Substitute α in Terms of a
Substituting α=ar into the torque equation:
T⋅r=12mdiscr2(ar)
This simplifies to:
T=12mdisca
Substituting mdisc=2kg:
T=12⋅2⋅a=a
Step 7: Substitute T Back into the Linear Equation
Now substituting T=a back into the linear motion equation:
mg−a=ma
This gives:
mg=ma+a
mg=a(m+1)
Solving for a:
a=mgm+1=9.812=4.905m/s2
Step 8: Calculate Tension
Since T=a:
T=4.905N
Step 9: Calculate Angular Displacement
Using the formula for angular displacement:
θ=ω0t+12αt2
Since the initial angular velocity ω0=0:
θ=12αt2
Where α=ar=4.9051=4.905rad/s2 and t=4s:
θ=12⋅4.905⋅(42)=12⋅4.905⋅16=39.24radians
Step 10: Calculate Work Done by Torque
The work done by torque is given by:
W=τ⋅θ
Where τ=T⋅r=4.905⋅1=4.905N m:
W=4.905⋅39.24=192.3J
Conclusion
1. The tension in the cord is approximately 4.905N.
2. The angular displacement of the disc in the first 4 seconds is approximately 39.24radians.
3. The work done by torque on the disc is approximately 192.3J.
When the mass is released, it experiences two forces:
1. The gravitational force (weight) acting downward, Fg=mg.
2. The tension in the cord acting upward, T.
- Weight, Fg=mg=1×9.81=9.81N.
Using Newton's second law for the mass, we can write:
mg−T=ma
Where a is the linear acceleration of the mass.
The linear acceleration a of the mass is related to the angular acceleration α of the disc by the equation:
a=rα
Where r is the radius of the disc.
The torque τ acting on the disc due to the tension in the cord is given by:
τ=T⋅r
The moment of inertia I for a disc about its center is:
I=12mdiscr2
Assuming the mass of the disc is 2kg.
Using Newton's second law for rotation:
τ=Iα
Substituting the expressions for torque and moment of inertia:
T⋅r=12mdiscr2α
Substituting α=ar into the torque equation:
T⋅r=12mdiscr2(ar)
This simplifies to:
T=12mdisca
Substituting mdisc=2kg:
T=12⋅2⋅a=a
Now substituting T=a back into the linear motion equation:
mg−a=ma
This gives:
mg=ma+a
mg=a(m+1)
Solving for a:
a=mgm+1=9.812=4.905m/s2
Since T=a:
T=4.905N
Using the formula for angular displacement:
θ=ω0t+12αt2
Since the initial angular velocity ω0=0:
θ=12αt2
Where α=ar=4.9051=4.905rad/s2 and t=4s:
θ=12⋅4.905⋅(42)=12⋅4.905⋅16=39.24radians
The work done by torque is given by:
W=τ⋅θ
Where τ=T⋅r=4.905⋅1=4.905N m:
W=4.905⋅39.24=192.3J
1. The tension in the cord is approximately 4.905N.
2. The angular displacement of the disc in the first 4 seconds is approximately 39.24radians.
3. The work done by torque on the disc is approximately 192.3J.
Topper's Solved these Questions
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Multiple Correct
26 Videos
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Linked Comprehension
71 Videos
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Subjective
19 Videos
- RIGID BODY DYNAMICS 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Integer
11 Videos
- SOUND WAVES AND DOPPLER EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer
16 Videos
Similar Questions
A ring rolls without slipping on the ground. Its centre C moves with a constant speed u.P is any point on the ring. The speed of P with respect to the ground is v.
View SolutionA man of mass 100kg stands at the rim of a turtable of radius 2m and moment of inertia 4000kgm2 mounted on a vertical frictionless shaft at its centre. The whole system is initially at rest. The man now walks along the outer edge of the turntable with a velocity of 1m/s relative to the earth
a. With what angular velocity and in what direction does the turntable rotate?
b. Through what angle will it have rotated when the man reaches his initial position on the turntable?
c. Through what angle will it have rotated when the man reaches his initial position relative to the earth?
View SolutionA uniform thin circular ring of mass m(m=0.4kg) and radius R has a small particle of the same mass m fixed on it as shown in Fig. The line joining the particle to centre is initially horizontal. The ground is frictionless. Find the contact force (magnitude) exerted by the ground on the ring, when the system is released from rest.

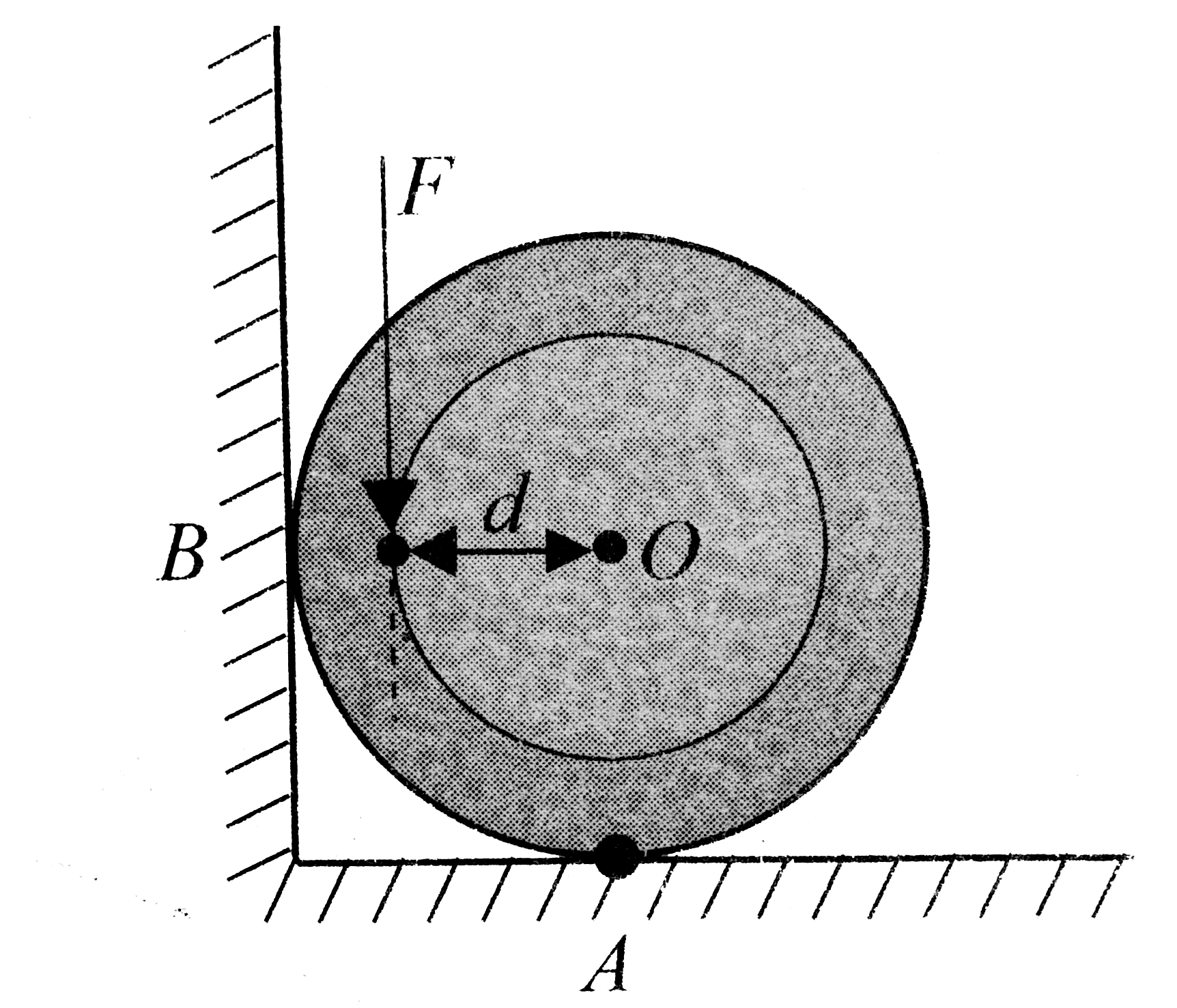
View SolutionA solid cylinder with r=0.1m and mass M=2kg is placed such that it is in contact with the vertical and a horizontal surface as shown in Fig. The coefficient of friction is μ=(1/3) for both the surfaces. Find the distance (in CM) from the centre of the cylinder at .which a force F=40N should be applied vertically so that the cylinder just starts rotating in anticlockwise direction.
View SolutionThe average power transmitted across a cross section by two sound waves moving in the same direction are equal. The wavelength of two sound waves are in the ratio of 1:2, then find the ratio of their pressure amplitudes.
View SolutionA uniform disc of mass M and radius R is mounted on an axle supported in frictionless bearings. A light cord is wrapped around the rim of the disc and a steady downward pull T is exerted on the cord. The angular acceleration of the disc is
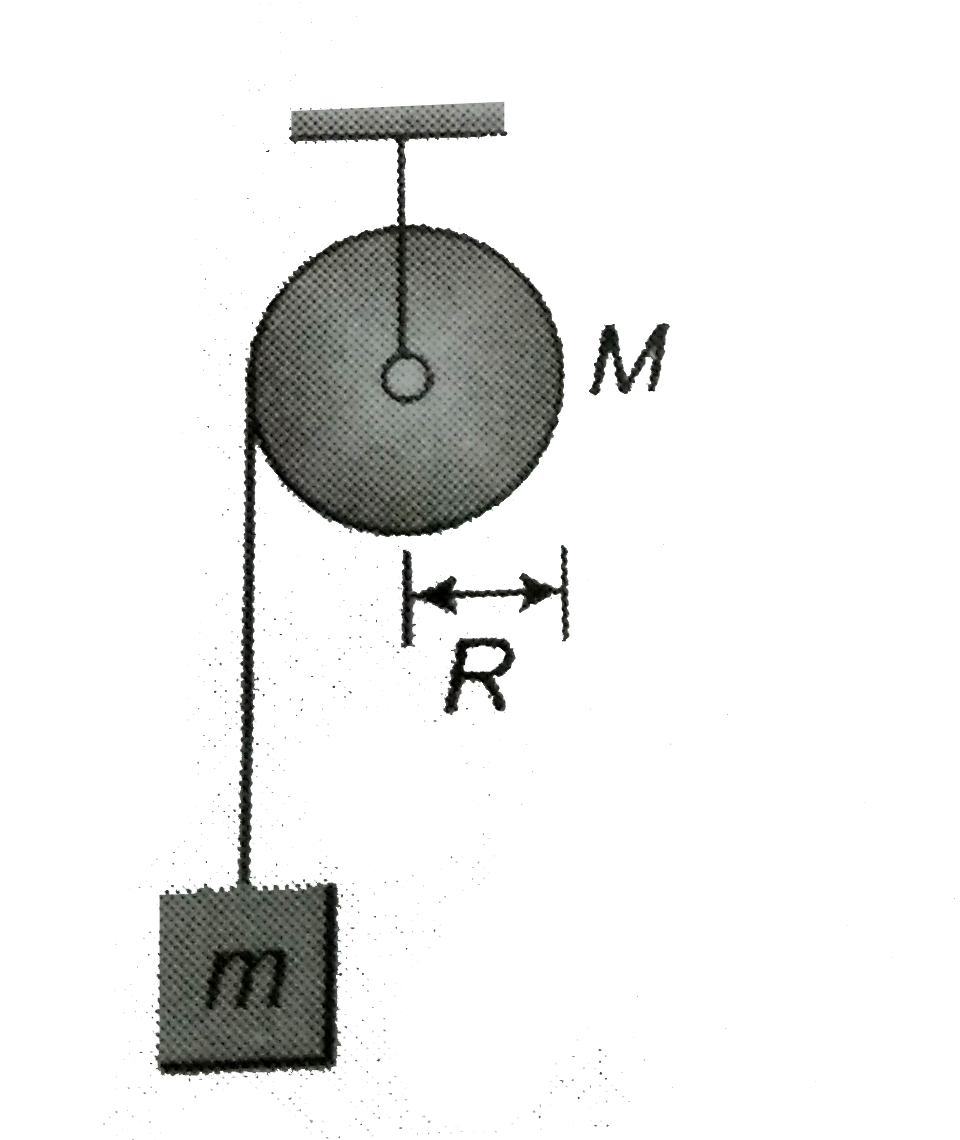
View SolutionA frictionless pulley has the shape of a uniform solid disc of mass M and radius R. A stone of mass m is attached to a light wire that is wrapped around the rim of the pulley and a system is released from rest. If a stone falls by a distance h, what is the angular speed of pulley at that moment?
View SolutionTwo discs A and B each of mass 2kg and radius 0.5m are rigidly fixed to the end of a thin shaft S , which is supported in horizontal position with the help of two smooth bearings at points P_(1) and P_(2) . Two blocks of masses 2 kg and 1 kg are connected to the light cords wrapped around the discs as shown in the figure. There is no slipping between the cord and the discs. If the system is released from rest from the position shown, the mark released from rest from the position shown,
View SolutionIn the arrangement shown in figure two equal masses (each m) hung light cords wrapped around a uniform solid cylinder of mass M and radius R. The cylinder is free to roate about a harizontal axis. If the system is released from rest then, the tension in each cord is-

View SolutionA disc of mass m and radius r is free to rotate about its centre as shown in the figure. A string is wrapped over its rim and a block of mass m is attached to the free end of the string. The system is released from rest. The speed of the block as it descends through a height h, is :-

View SolutionA uniform disc of radius R and mass M is free to rotate only about its axis. A string is wrapped over its rim and a body of mass m is tied to the free end of the string as shown in the figure. The body is released from rest. Then the acceleration of the body is
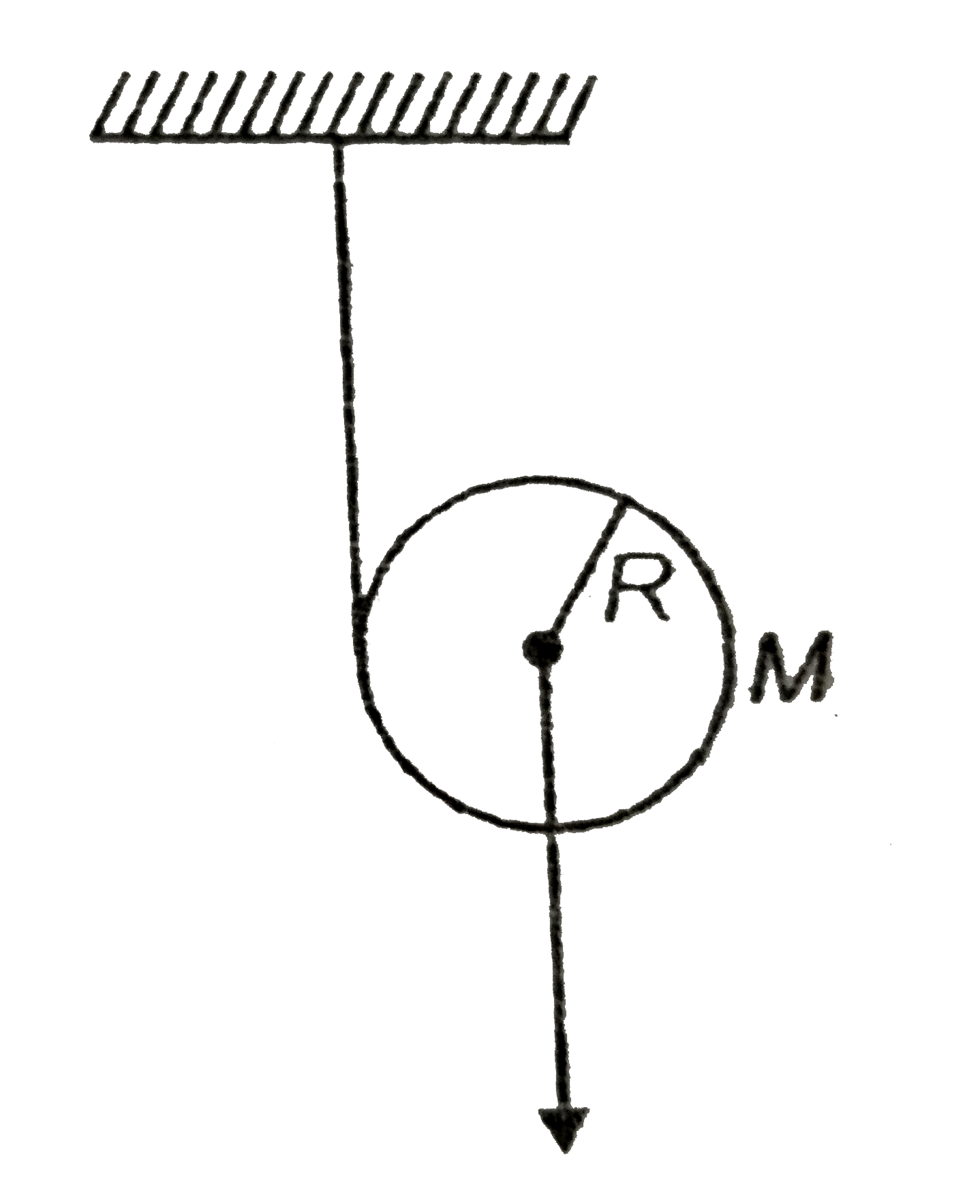
View SolutionA disc of mass M has a light, thin string wrapped several times around its circumference. The free end of string is attaced to the ceiling and the disc is released from rest. Find the acceleration of the disc and the tension in the string.
View SolutionM1=0.3 किग्रा द्रव्यमान का एक पिण्ड छत से एक हल्की डोरी द्वारा लटकाया गया है। M2=0.7 किग्रा का दूसरा पिण्ड प्रथम पिण्ड से दूसरी हल्की डोरी द्वारा लटकाया गया है। दोनों डोरियों में तनाव का परिकलन कीजिए।
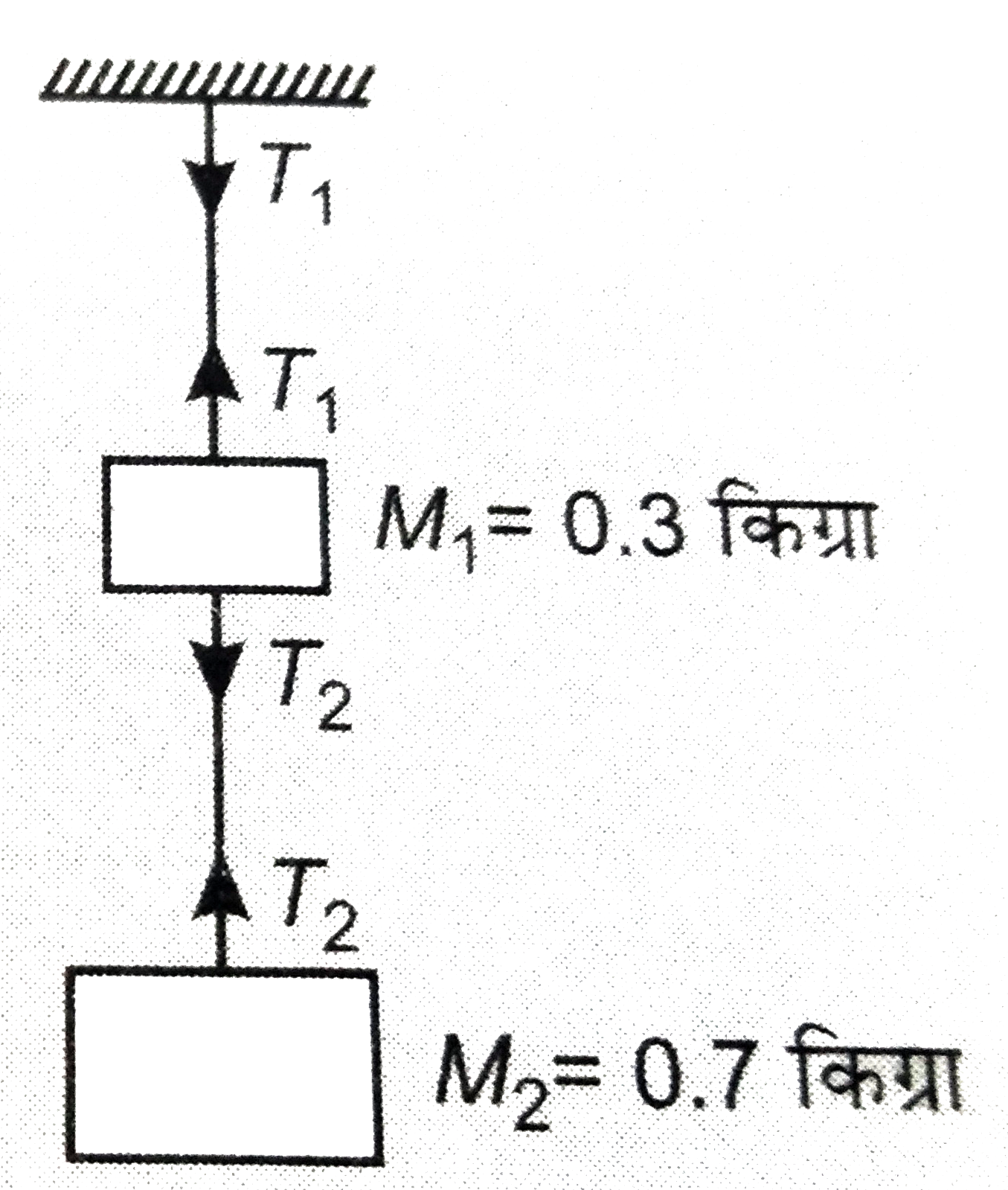
View SolutionA uniform disc of mass 2 kg and radius 1m is mounted on an axle supported on fixed frictionless bearings. A light chord is wrapped around the rim of the disc and mass of 1kg is tied to the free end. If it is released from rest-
View SolutionA uniform disc of radius R and mass M is free to rotate only about its axis. A string is wrapped over its rim and a body of mass m is tied to the free end of the string as shown in the figure. The body is released from rest. Then the acceleration of the body is

View Solution
