Suppose in the previous problem, the child stays at rest on the platform and one of his friend throws a ball of mas m1 towards him with a velocity of v from a direction tangential to the platform and the child on the platform catches the ball. Find the angular velocity of the platform after he catches the ball.
To solve the problem, we need to apply the principle of conservation of angular momentum. Here are the steps to find the angular velocity of the platform after the child catches the ball:
1. Identify the Initial Conditions:
- The system consists of a child of mass m standing on a circular platform with moment of inertia I and radius r.
- A ball of mass m1 is thrown towards the child with a velocity v tangentially.
2. Calculate Initial Angular Momentum:
- Since the platform is initially at rest, the initial angular momentum Linitial can be calculated using the linear momentum of the ball.
- The linear momentum of the ball is p=m1v.
- The angular momentum of the ball about the center of the platform is given by:
Linitial=m1vr
3. Determine the Final Angular Momentum:
- After the child catches the ball, the system (child + ball) will rotate together with an angular velocity Ω.
- The moment of inertia of the system after catching the ball is:
Ifinal=I+mr2+m1r2
- Here, I is the moment of inertia of the platform, mr2 is the moment of inertia of the child, and m1r2 is the moment of inertia of the ball.
4. Apply Conservation of Angular Momentum:
- According to the conservation of angular momentum:
Linitial=Lfinal
- The final angular momentum can be expressed as:
Lfinal=IfinalΩ
- Therefore, we have:
m1vr=(I+mr2+m1r2)Ω
5. Solve for Angular Velocity Ω:
- Rearranging the equation to solve for Ω:
Ω=m1vrI+mr2+m1r2
Final Answer:
The angular velocity of the platform after the child catches the ball is:
Ω=m1vrI+mr2+m1r2
- The system consists of a child of mass m standing on a circular platform with moment of inertia I and radius r.
- A ball of mass m1 is thrown towards the child with a velocity v tangentially.
- Since the platform is initially at rest, the initial angular momentum Linitial can be calculated using the linear momentum of the ball.
- The linear momentum of the ball is p=m1v.
- The angular momentum of the ball about the center of the platform is given by:
Linitial=m1vr
- After the child catches the ball, the system (child + ball) will rotate together with an angular velocity Ω.
- The moment of inertia of the system after catching the ball is:
Ifinal=I+mr2+m1r2
- Here, I is the moment of inertia of the platform, mr2 is the moment of inertia of the child, and m1r2 is the moment of inertia of the ball.
- According to the conservation of angular momentum:
Linitial=Lfinal
- The final angular momentum can be expressed as:
Lfinal=IfinalΩ
- Therefore, we have:
m1vr=(I+mr2+m1r2)Ω
- Rearranging the equation to solve for Ω:
Ω=m1vrI+mr2+m1r2
The angular velocity of the platform after the child catches the ball is:
Ω=m1vrI+mr2+m1r2
Topper's Solved these Questions
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Exercise 3.3
21 Videos
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Exercise 3.4
13 Videos
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Exercise 3.1
11 Videos
- RIGID BODY DYNAMICS 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Integer
11 Videos
- SOUND WAVES AND DOPPLER EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer
16 Videos
Similar Questions
A kid of mass M stands at the edge of a platform of radius R which can be freely rotated about its axis. The moment of inertia of the platform is I . The system is at rest when a friend throws as ball of mass m and the kid catches it. If the velocity of the ball is v horizontally along the tangent to the edge of the platform when it was caught by the kid find the angular speed of the platform after the event.
View SolutionA child of mass m is standing, on the periphery of a circular platform of radius R, which can rotate about its central axis. The moment of inertia of the platform is I. Child jumps off from the platform with a velocity u tangentially relative to the platform. Find the angular speed of the platform after the child jumps off.
View Solution
Knowledge Check
A circular platform is mounted on a vertical frictionless axle. Its radius is r=2m and its moment of inertiaI=200kgm2. It is initially at rest. A 70kg man stands on the edge of the platform and begins to walk along the edge at speed v0=1ms−1 relative to the ground. The angular velocity of the platform is: a) 1.2 rad/s b) 0.4 rad/s c) 0.7 rad/s d) 2 rad/s
A1.2rads−1B0.4rads−1C0.7rads−1D2rads−1
A uniform rod of mass m and length L is at rest on a smooth horizontal surface. A ball of mass m, moving with velocity v0, hits the rod perpendicularly at its one end and sticks to it. The angular velocity of rod after collision is

View SolutionTwo friends A and B (each weighing 40kg) are sitting on a frictionless platform some distance d apart. A rolls a ball of mass 4kg on the platform towards B which B catches. Then B rolls the ball towards A and A catches it. The ball keeps on moving back and forth between A and B. The ball has a fixed speed of 5m/s on the platform.
a. Find the speed of A after he rolls the ball for the first time.
b. Find the speed of A after he catches the ball for the first time.
c. Find the speed of A and B after the ball has made five round trips and is held by A.
d. How many times can A roll the ball?
e. Where is the centre of mass of the system A+B+ ball at. the end of the nth trip?
View SolutionTwo persons, A of mass 60kg and B of mass 40kg, are standing on a horizontal platform of mass 50kg. The platform is supported on wheels on a horizontal frictionless surface and is initially at rest. Consider the following situations.
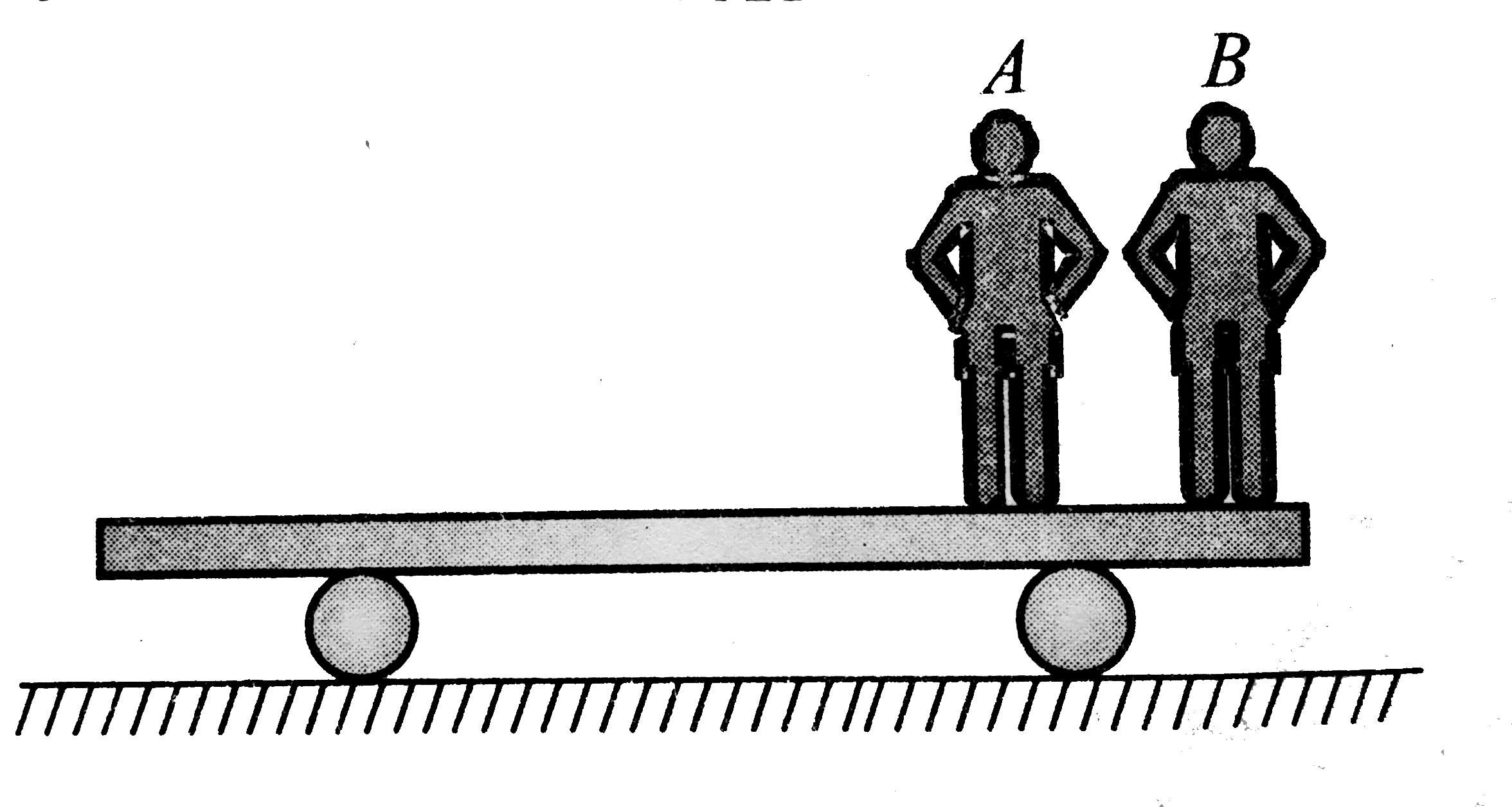
(i) Both A and B jump from the platform simultaneously and in the same horizontal direction.
(ii) A jumps first in a horizontal direction and after a few seconds B also jumps in the same direction. In both the situations above, just after the jump, the person (A or B) moves away from the platform with a speed of 3m/s relative to the platform and along the horizontal.
In situation (i), just after both A and B jump from the platform, velocity of centre of mass of the system (A,B and the platform) is
View SolutionFrom a lift moving upwards with a uniform acceleration a = 2ms–2, a man throws a ball vertically upwards. with a velocity v = 12ms−1 releative to lift the ball comes back to the man after a time t find. t
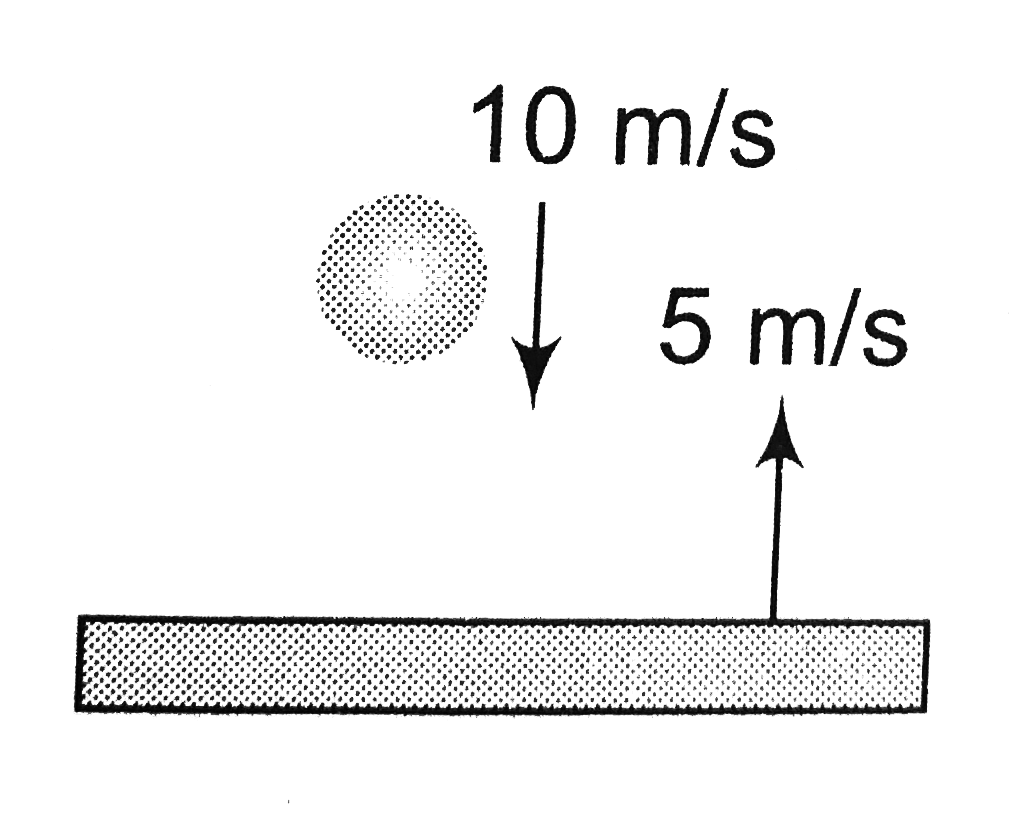
View SolutionA ball of mass 1kg strikes a heavy plarform elastically, moving upwards with a velocity of 5m/s . The speed of the ball just before the collision is 10m/s downwards. Then the impulse imparted by the platform on the ball is
View SolutionA bullet of mass m moving at a speed v hits a ball of mass M kept at rest. A small part having mass m ′ breaks from the ball and sticks to the bullet. The remaining ball is found to move at a speed v 1 , in the direction of the bullet. Find the velocity of the bullet after the collision.
View SolutionA flat car of mass M is at rest on a frictionless floor with a child of mass m standing at its edge. If the child jumps off from the car towards right with an initial velocity u, with respect to the car, find the velocity of the car after its jump.
View Solution
