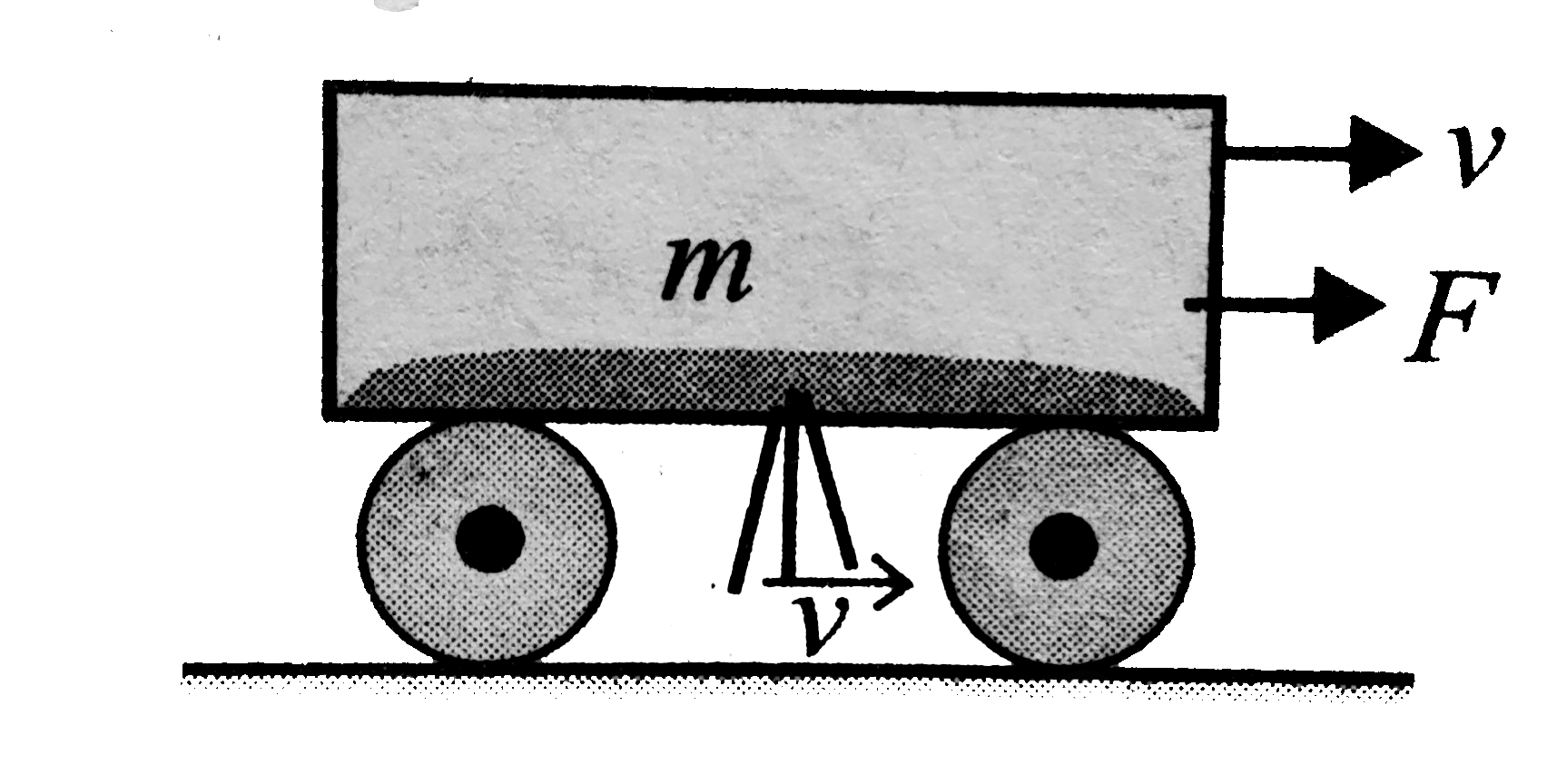
A cart loaded with sand moves along a horizontal floor due to a constant force F coinciding in direction with cart's velocity vector. In the process, the sand spills through a hole in the bottom with a constant rate μkg/s. Find the acceleration and velocity of the cart at the moment t, if at the initial moment t=0 the cart with loaded sand had mass m0 and its velocity was equal to zero. Friction is to be neglected.
In this problem the sand spills through a hole at the bottom of the cart. Hene ,the relative velocity of the sand vr will be zero, because it will acquire the same velocity as that of the cart at the moment of separation.
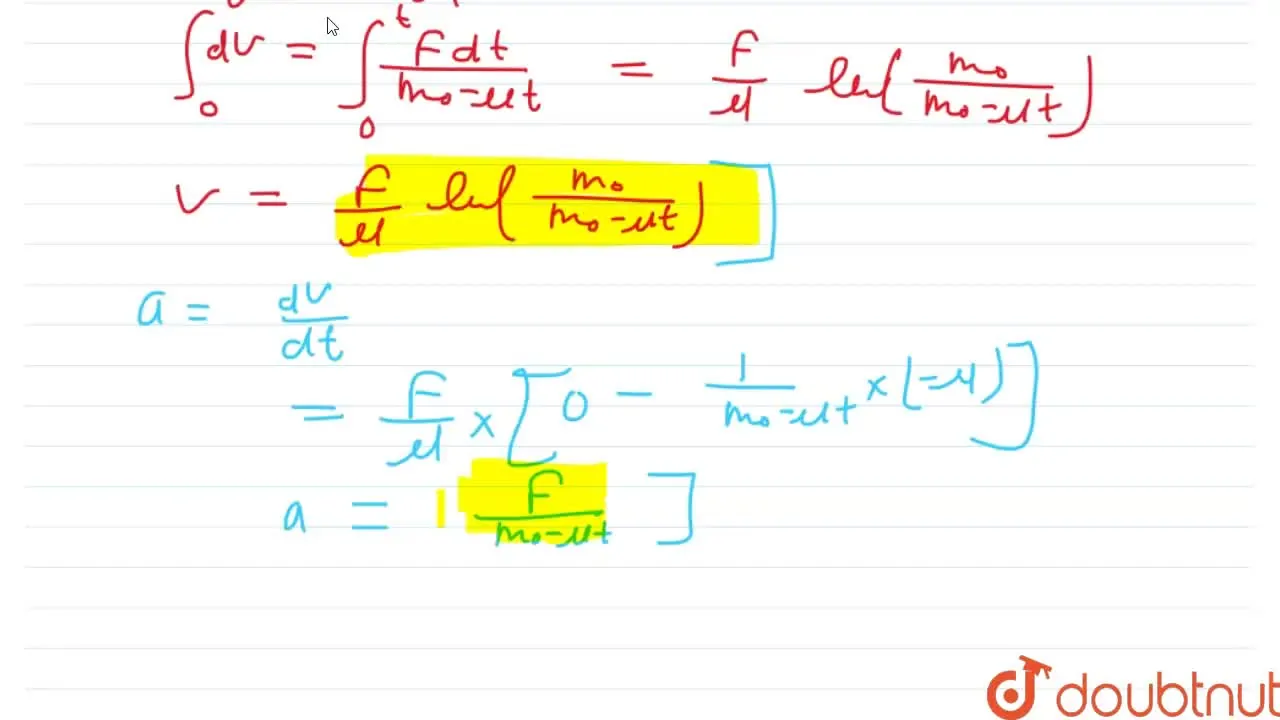
vrel=0
Apply F=vreldmdt=mdvdt
⇒F+0×(dmdt)=(m0−μt)dvdt .............i
⇒(m0−μt)dvdt=F
or ∫v0dv=∫t0Fdtm0−μt
⇒v=F−μ[In(m0−μt)]t0
or v=FμIn(m0m0−μt)
Frome eqn i acceleration of the cart
a=dvdt=Fm or a=Fm0−μt
Related Playlists
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Solved Examples13videos
CENTRE OF MASS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Exercise 1.120videos
CENTRE OF MASS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Solved Example13videos
CALORIMETRY
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer2videos
DIMENSIONS & MEASUREMENT
Similar Questions
In the figure shown a cart moves on a smooth horizontal surface due to an external constant force of magnitude F. The initial mass of the cart is M0 and velocity is zero. Sand falls on to the cart with negligible velocity at constant rate μkg/s and sticks to the cart. The velocity of the cart at time t is :
 View Solution
View SolutionA cart loaded with sand having total mass m0=1800kg moves on a straight horizontal road starting from rest under the action of a force of 120N. The sand spills through a small hole hole in the bottom at a rate of 0.5 kg/sec. What will be the velocity of cart after 20 min?
View SolutionA cart moves with a constant speed along a horizontal circular path. From the cart, a particle is thrown up vertically with respect to the cart.
View SolutionA particle of mass m is moving along the line y-b with constant acceleration a. The areal velocity of the position vector of the particle at time t is (u=0)
View SolutionA body is just supported at the face of a cart moving at an acceleration. The acceleration of the cart so that the body does not slide
View SolutionA particle moves rectilinearly with initial velocity u and constant acceleration a. Find the average velocity of the particle in a time interval from t=0 to t=t second of its motion.
View SolutionA railway carriage of mass Mc filled with sand of mass Ms moves along the rails. The carriage is given an impulse and it starts with a velocity v0. At the same time it is observed that the sand starts leaking through a hole at the bottom of the carriage at a constant mass rate λ. Find the distance at which the carriage becomes empty and the velocity attained by the carriage at that time. (Neglect the friction along the rails).
View SolutionA constant force F is applied on a trolley of initial mass m0 kept over a smooth surface. Sand is poured gently over the trolley at a constant rate of (μ)kg//s. Afer time t, find

(a) mass of the trolley (with sand)
(b) net force on the trolley
(c) velocity of trolleyView SolutionA horse continues to apply a force in order to move a cart with a constant speed. Explain why?
View SolutionA cart is moving with a velocity 20m/s. Sand is being dropped into the cart at the rate of 50 kg/min. The force required to move the cart with constant velocity will be :-
View Solution