A particle is taken from point A to point B under the influence of a force field. Now it is taken back from B to A and it is observed that the work done in taking the particle from A to B is not equal to the work done in taking it from B to A. If Wnc and Wc are the work done by non-conservative and conservative forces present in the system, respectively, ΔU is the change in potential energy and Δk is the change in kinetic energy, then
(a) Wnc−ΔU=Δk
(b) Wc=−ΔU
(c) Wnc+Wc=Δk
(d) Wnc−ΔU=−Δk
The correct Answer is:A, B, C
According to the work-energy theorem for a non-conservative system, we have Wc+Wnc=ΔK
Also, we know that the work done by a conservative force equals the decrease in potential energy, so Wc=−ΔU
Wnc−ΔU=ΔK
Topper's Solved these Questions
- WORK, POWER & ENERGYBook:CENGAGE PHYSICS ENGLISHChapter:WORK, POWER & ENERGYExercise:Linked Comprehension
Explore 55 Videos
- WORK, POWER & ENERGYBook:CENGAGE PHYSICS ENGLISHChapter:WORK, POWER & ENERGYExercise:Integer
Explore 14 Videos
- WORK, POWER & ENERGYBook:CENGAGE PHYSICS ENGLISHChapter:WORK, POWER & ENERGYExercise:Single Correct
Explore 100 Videos
- VECTORSBook:CENGAGE PHYSICS ENGLISHChapter:VECTORSExercise:Exercise Multiple Correct
Explore 5 Videos
Similar Questions
Knowledge Check
Work done in carrying a particle from A to B is independent of the path chosen implies that
Athe linear momentum is conservedBthe angular momentum is conservedCthe component of the angular momentum along the line joining A and B is conserved.DThe potential function can be defined.
Work done by the conservative force on a system is equal to :
View SolutionThe work done in taking a unit positive charge from P to A is WA and P to B is WB. Then
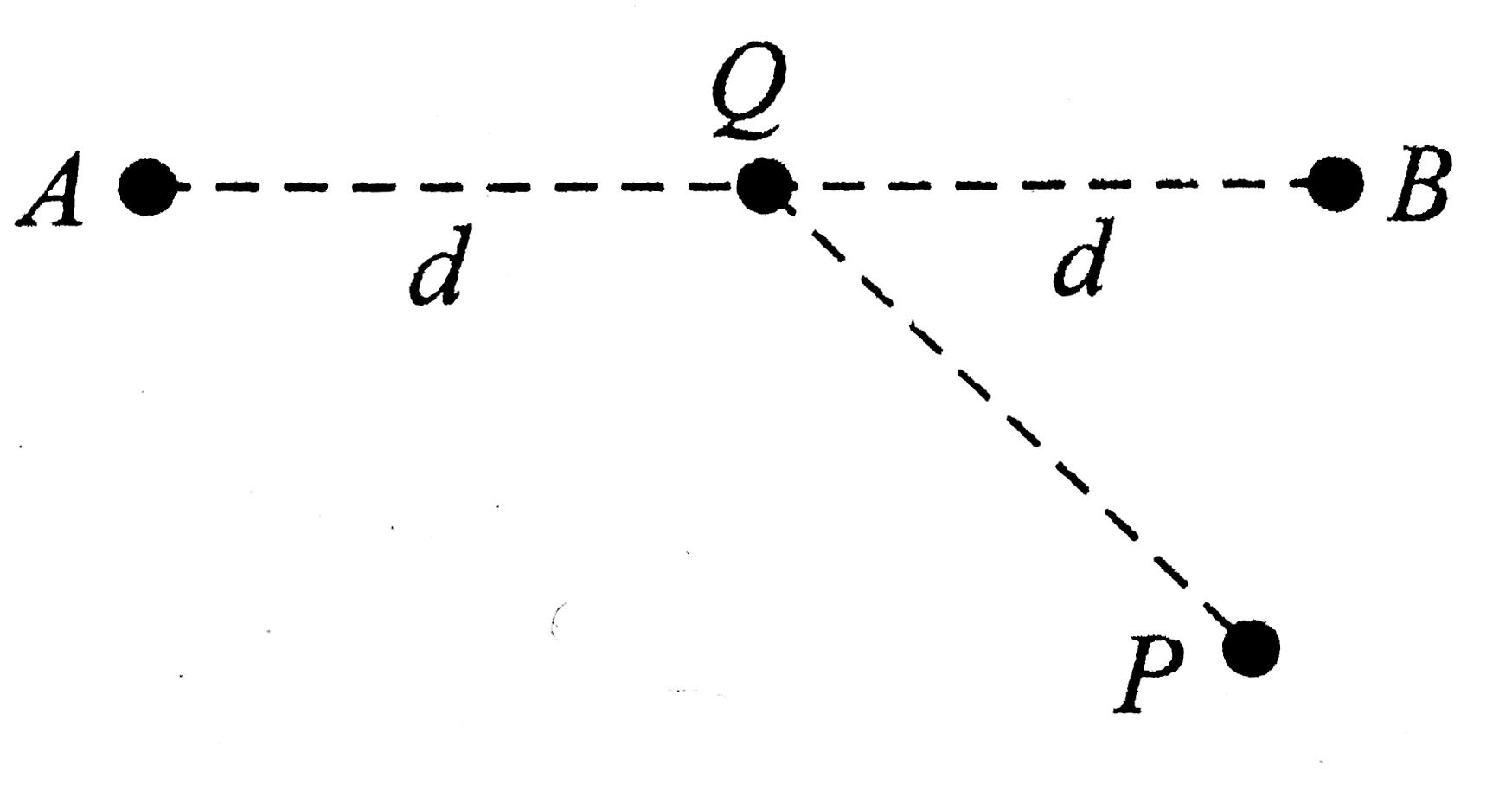 .
.
View SolutionA point charge is taken from a point A to a pint B in an electric field. Does the work done by the electric field depend on the path of the charge ?
View SolutionParticle moves from point A to point B along the line shown in figure under the action of force. VecF=−xˆi+yˆj. Determine the work done on the particle by →F in moving the particle from point A to point B.
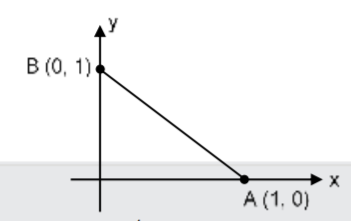
View SolutionParticle moves from point A to point B along the line shown in figure under the action of force. evcF=−xˆi+yˆj. Determine the work done on the particle by →F in moving the particle from point A to point B.

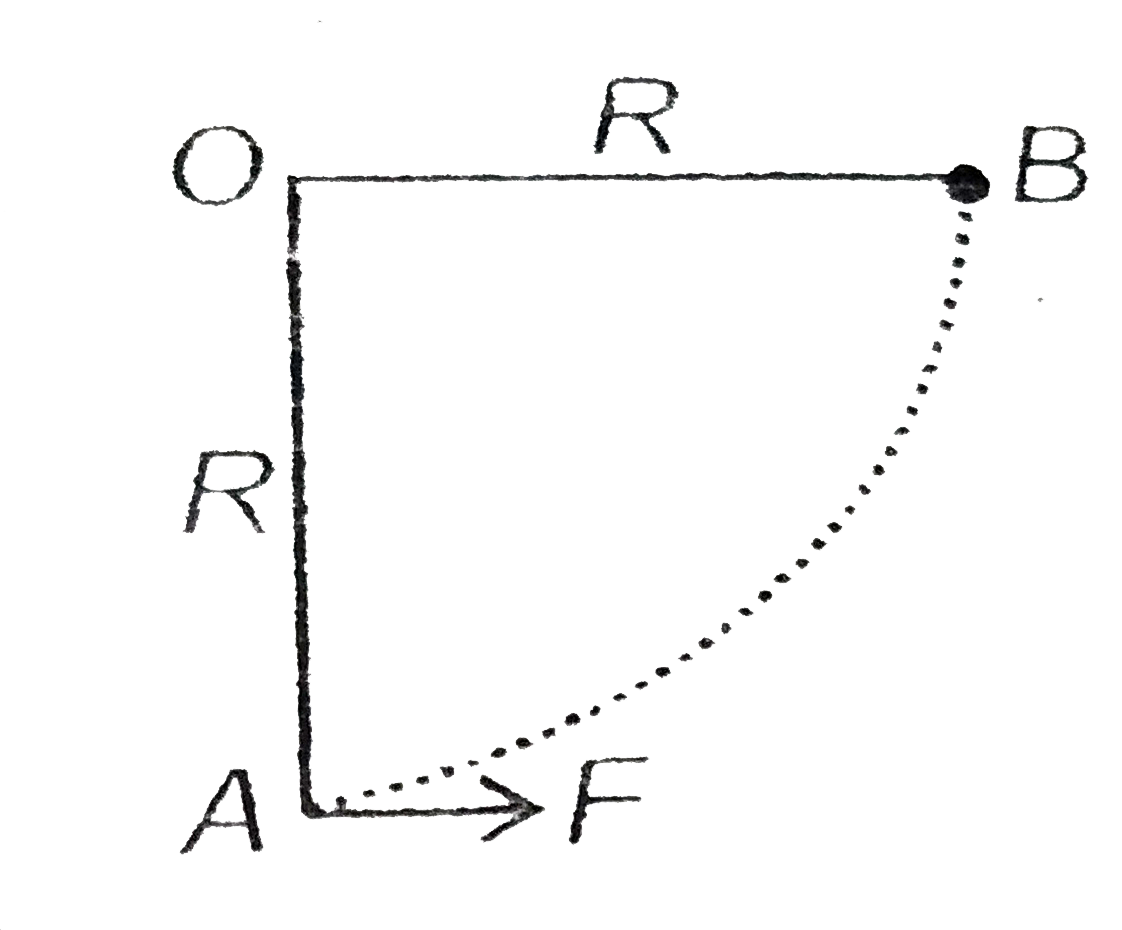
View SolutionA block of mass m is taken from A to B under the action of a constant force F. Work done by this force is
View SolutionConsider the situation of figure . The work done in taking a point charge form P to A isWA from P to B is WB from P to C is WC.

View Solution