Question

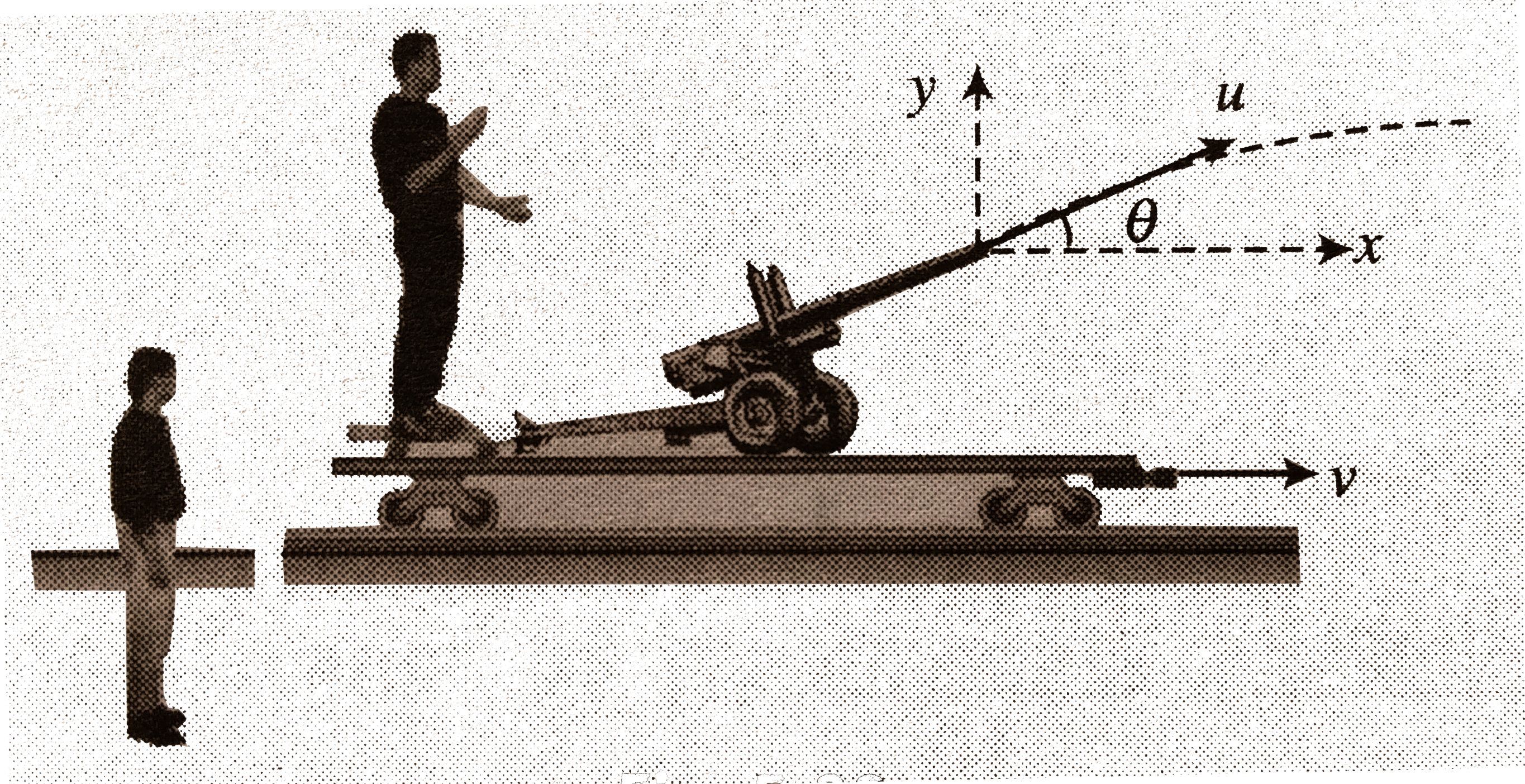
A shell is projected from a gun with a muzzle velocity, $u$. The gun is fitted with a trolly car at an angle $\theta$ as shown in (Fig. 5.33). If the trolley car is made to move with constant velocity $v$ towards right, find the
(a) horizontal range of the shell relative to ground.
(b) horizontal range of the shell relative to a person travelling with trolley.
.

Explanation

The shell will follow parabolic path as seen from the observer travelling with trolley as well as seen from the observer on ground.
(a) The velocity of projection of the shell is `vec v_s = vec v _(s c) + vec V_c`.
Substituting `vec u_(s c) = u cos theta hat i+u sin theta hat j and vec v_c = vec v hat i`, we have
`vec u_s = (u cos theta + v)hat i + u sin theta hat j`
For horizontal range `R` of the shell, its displacement in horizontal direction can be given as `vec s = R hat i, vec a = - g hat j and vec u_s = (u cos theta + v) hat i+ u sin theta hat j`
Using `vec s = vec u t + (1)/(2) vec a t^2,` we have
`R hat i = (u cos theta + v) t hat i + (u t sin theta - (1)/(2) "gt"^2)) hat j`
Comparing the coefficient of `hat i and hat j`, we obtain
`R = (u cos theta + v)t` ...(i)
and `ut sin theta - (1)/(2) "gt"^2 = 0` ...(ii)
From (ii), we find `t = (2 u sin theta)/(g)`
Or we should have directly used the formula for the time of flight directly to get same result.
Finally, substituting `t = (2 u sin theta)/(g)` in (i), we have
`R = (2 u sin theta (u cos theta + v))/(g)`
(b) The horizontal component of the initial velocity of the shell, as seen from trolley, `u_x = u cos theta`.
The vertical component, `u_y = u sin theta`.
The time of flight of shell will be same for both observers at trolley as well as ground.
Hence, range as seen from trolley is equal to the horizontal displacement in x - direction q.r.t trolley.
`R' = (u cos theta) t = u cos theta xx ((2 u sin theta)/(g)) = (2 u^2 sin theta. cos theta)/(g)`
=`(u^2 sin 2 theta)/(g)`.