The numuerical value of the ratio of instantaneous velocity to instantaneous spedd is.
Always less than 1
Always equal to 1
Always more than 1
Equal to or less than 1
The correct Answer is:B
At and instant. The magnitudes of velocity and speed will be equal.
Related Playlists
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-1EXERCISE - Graphical Concept17videos
KINEMATICS-1
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-1EXERCISE - Graphical cancept1videos
KINEMATICS-1
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-1EXERCISE - Subjective29videos
KINEMATICS-1
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GRAVITATIONEXERCISE - INTEGER_TYPE1videos
GRAVITATION
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-2EXERCISE - Exercise Integer9videos
KINEMATICS-2
Similar Questions
A particle is moving with a constant speed in a circular path. Find the ratio of average velocity to its instantaneous velocity when the particle rotates an angle θ=(π2) .
View SolutionInstantaneous source of energy is
View SolutionInstantaneous source of energy is
View SolutionAssertion: Average speed of a particle in a given time interval is never less than the magnitude of the average velocity.
Reason: The magnitude of the velocity (instantaneous velocity) of a particle is equal to its speed.View SolutionHow is average velocity different from instantaneous velocity ?
View SolutionThe variation of the instantaneous current (I) and the instantaneous e.m.f (E) in a circuit is as shown in figure. Which of the following statement is correct?
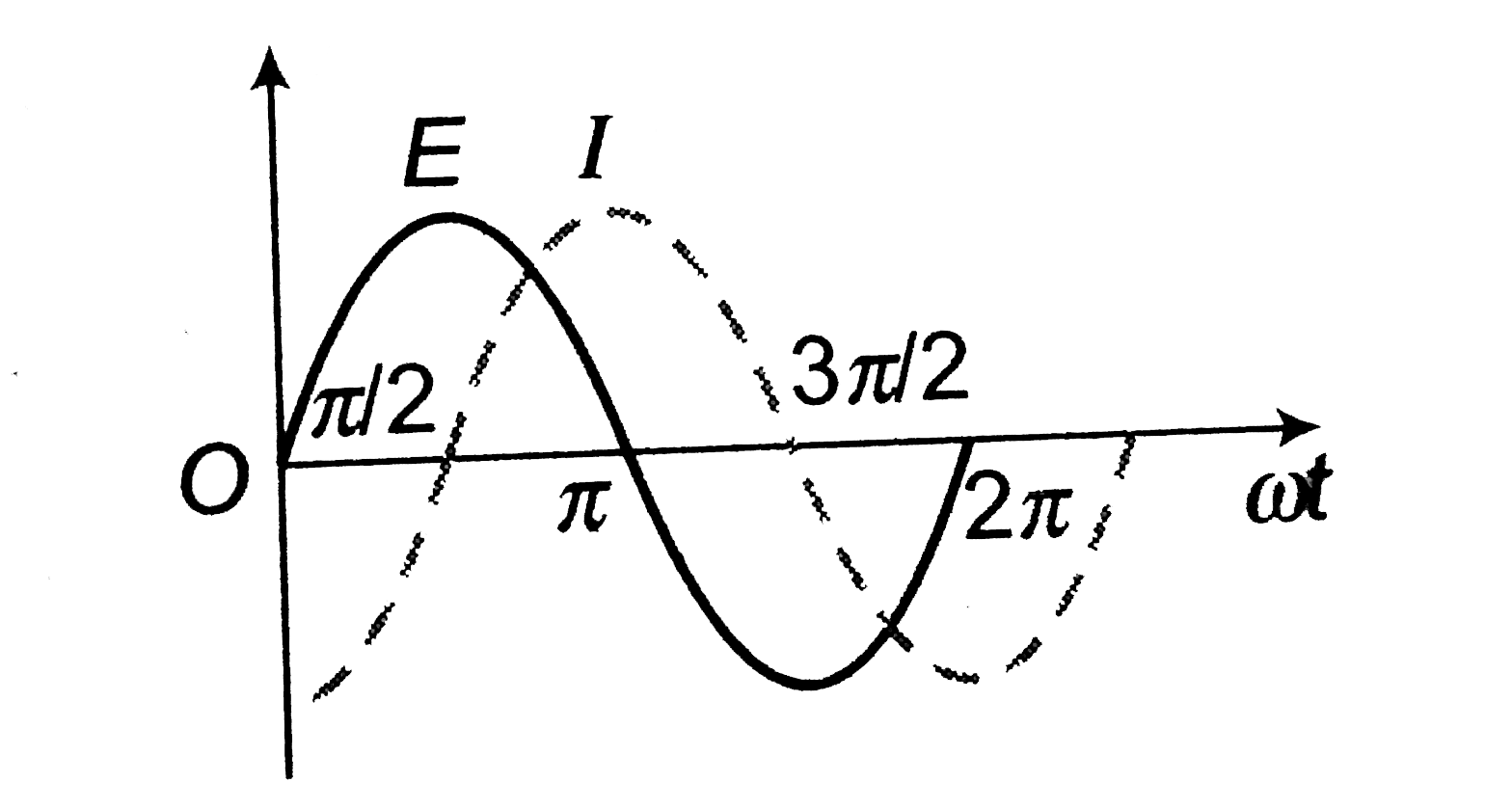 View Solution
View SolutionStatement I: The average velocity of the body may be equal to its instantaneous velocity.
Statement II: For a given time interval of a given motion, average veocity is single valued while average speed can have many values.View SolutionA spaceship in space sweeps stationary interplanetary dust . As a result , its mass increase at a rate dM(t)dt=bv2(t) , where v(t) is its instantaneous velocity . The instantaneous acceleration of the satellite is :
View SolutionWhen a particle moves with constant velocity, its average velocity, its instantaneous velocity and its speed are all equal. Comment on this statement.
View SolutionWhen a particle moves with constant velocity its average velocity, its instantaneous velocity and its speed all are equal. Is this statement true or false ?
View Solution


