A sound wave having wavelength λ forms stationary waves after reflection from a surface. The distance between two consecutive nodes is
λ
λ/2
λ/4
λ/8
The correct Answer is:A
To solve the problem of finding the distance between two consecutive nodes in a stationary wave formed by a sound wave reflecting from a surface, we can follow these steps:
1. Understanding the Concept of Stationary Waves:
- A stationary wave is formed when two waves of the same frequency and amplitude travel in opposite directions and interfere with each other. In this case, one wave is traveling towards a rigid surface and reflects back.
2. Identifying Nodes and Antinodes:
- In a stationary wave, nodes are points where there is no displacement (the wave does not move), and antinodes are points where the displacement is maximum. The nodes occur at regular intervals along the wave.
3. Wavelength Definition:
- The wavelength (λ) is the distance between two consecutive points that are in phase (e.g., crest to crest or trough to trough).
4. Relationship Between Wavelength and Nodes:
- In a stationary wave, the distance between two consecutive nodes is half the wavelength. This is because a full wavelength consists of one complete cycle of the wave, which includes one node and one antinode.
5. Calculating the Distance Between Nodes:
- Since the distance between two consecutive nodes is half of the wavelength, we can express this mathematically as:
Distance between two nodes=λ2
6. Conclusion:
- Therefore, the distance between two consecutive nodes in the stationary wave formed by the reflection of the sound wave is λ2.
Final Answer:
The distance between two consecutive nodes is λ2.
- A stationary wave is formed when two waves of the same frequency and amplitude travel in opposite directions and interfere with each other. In this case, one wave is traveling towards a rigid surface and reflects back.
- In a stationary wave, nodes are points where there is no displacement (the wave does not move), and antinodes are points where the displacement is maximum. The nodes occur at regular intervals along the wave.
- The wavelength (λ) is the distance between two consecutive points that are in phase (e.g., crest to crest or trough to trough).
- In a stationary wave, the distance between two consecutive nodes is half the wavelength. This is because a full wavelength consists of one complete cycle of the wave, which includes one node and one antinode.
- Since the distance between two consecutive nodes is half of the wavelength, we can express this mathematically as:
Distance between two nodes=λ2
- Therefore, the distance between two consecutive nodes in the stationary wave formed by the reflection of the sound wave is λ2.
The distance between two consecutive nodes is λ2.
Topper's Solved these Questions
- COMPETITION CARE UNITBook:ICSEChapter:COMPETITION CARE UNITExercise:OBJECTIVE QUESTIONS FROM PREVIOUS IAS EXAMINATIONS
Explore 50 Videos
- COMPETITION CARE UNITBook:ICSEChapter:COMPETITION CARE UNITExercise:WAVES
Explore 98 Videos
- CIRCULAR MOTION Book:ICSEChapter:CIRCULAR MOTION Exercise:MODULE 2 (FROM ROTATIONAL KINETIC ENERGY , WORK ,POWER)
Explore 24 Videos
- DIMENSIONSBook:ICSEChapter:DIMENSIONSExercise:SELECTED PROBLEMS (FROM CONVERSIONS OF ONE SYSTEMS OF UNITS INTO ANOTHER)
Explore 9 Videos
Similar Questions
Two waves are approaching each other with a velocity of 20m/s and frequency n. The distance between two consecutive nodes is
View SolutionWaves of wavelength λ are generated on the water surface. The phase difference between two points at a distance of λ is
View SolutionThe change in phase if a wave is reflected from a rigid surface is
View SolutionA tube closed at one end has a vibrating diaphragm at the other end , which may be assumed to be a displacement node . It is found that when the frequency of the diaphragm is 2000Hz , a stationary wave pattern is set up in which the distance between adjacent nodes is 8cm. When the frequency is gradually reduced , the stationary wave pattern reappears at a frequency of 1600Hz. Calculate
i. the speed of sound in air ,
ii. the distance between adjacent nodes at a frequency of 1600Hz,
iii. the distance between the diaphragm and the closed end ,
iv. the next lower frequencies at which stationary wave patterns will be obtained.
View SolutionA sound wave of frequency 500 Hz is producing longitudinal waves in air. The distance between two consecutive rarefactions in the wave is 0.64 m and the amplitude of the oscillation of air particle is 0.002 m. Obtain the displacement equation of this wave.
View SolutionA driver is riding a car with velocity vc between two vertical walls on a horizontal surface as shown in figure.A source of sound of frequency 'f' is situated on the car. (vc<<v, where v is the speed of sound in air )
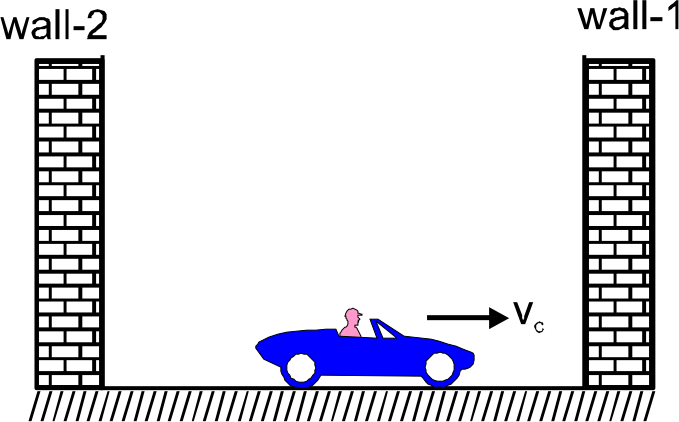
Consider the sound wave observed by the driver directly from car has a wavelength λ1 and the sound wave after reflection from wall-1 observed by the driver has wavelength λ2 then λ1−λ2λ1+λ2 is :
View SolutionA standing wave in second overtone is maintained in a open pipe of length L. The distance between consecutive displacement node and pressure node is L/x. Find x
View SolutionHertz , in his historical experiment, produced stationary EM waves and measured the distance between two successive nodes. Explain how this measurement enabled him to show that EM waves travelled with the same speed as the speed of light.
View SolutionWhen a sound wave is reflected from a wall the phase difference between the reflected and incident pressure wave is:
View SolutionWhen a sound wave is reflected from a wall the phase difference between the reflected and incident pressure wave is:
View Solution