A particle moves such that its accleration a is given by a=−bx, where x is the displacement from equilibrium position and b is a constant. The period of oscillation is
√2π/b
2π/√b
2π/b
2√(π/b)
The correct Answer is:B
To solve the problem, we need to determine the period of oscillation for a particle whose acceleration is given by the equation a=−bx, where x is the displacement from the equilibrium position and b is a constant.
1. Understanding the Given Equation:
The acceleration a is given by a=−bx. This indicates that the acceleration is directly proportional to the displacement x but acts in the opposite direction (hence the negative sign). This is characteristic of simple harmonic motion (SHM).
2. Identifying the Nature of Motion:
Since the acceleration is proportional to the displacement and directed towards the equilibrium position, we can conclude that the particle is undergoing simple harmonic motion (SHM).
3. Using the Formula for Period in SHM:
The formula for the period T of a simple harmonic oscillator is given by:
T=2π√mk
where m is the mass of the particle and k is the spring constant. In our case, we can relate the given acceleration to the standard form of SHM.
4. Relating Acceleration to SHM:
In SHM, we have:
a=−ω2x
where ω is the angular frequency. Comparing this with our equation a=−bx, we can identify:
ω2=b
5. Finding the Period:
The angular frequency ω is related to the period T by the formula:
T=2πω
Substituting ω=√b into the period formula gives us:
T=2π√b
6. Final Expression for the Period:
Therefore, the period of oscillation T is:
T=2π√1b
Conclusion:
The period of oscillation for the particle is given by:
T=2π√1b
The acceleration a is given by a=−bx. This indicates that the acceleration is directly proportional to the displacement x but acts in the opposite direction (hence the negative sign). This is characteristic of simple harmonic motion (SHM).
Since the acceleration is proportional to the displacement and directed towards the equilibrium position, we can conclude that the particle is undergoing simple harmonic motion (SHM).
The formula for the period T of a simple harmonic oscillator is given by:
T=2π√mk
where m is the mass of the particle and k is the spring constant. In our case, we can relate the given acceleration to the standard form of SHM.
In SHM, we have:
a=−ω2x
where ω is the angular frequency. Comparing this with our equation a=−bx, we can identify:
ω2=b
The angular frequency ω is related to the period T by the formula:
T=2πω
Substituting ω=√b into the period formula gives us:
T=2π√b
Therefore, the period of oscillation T is:
T=2π√1b
The period of oscillation for the particle is given by:
T=2π√1b
Topper's Solved these Questions
- COMPETITION CARE UNITBook:ICSEChapter:COMPETITION CARE UNITExercise:WAVES
Explore 98 Videos
- COMPETITION CARE UNITBook:ICSEChapter:COMPETITION CARE UNITExercise:NDA EXAM QUESTIONS
Explore 55 Videos
- COMPETITION CARE UNITBook:ICSEChapter:COMPETITION CARE UNITExercise:THERMAL RADIATION
Explore 13 Videos
- CIRCULAR MOTION Book:ICSEChapter:CIRCULAR MOTION Exercise:MODULE 2 (FROM ROTATIONAL KINETIC ENERGY , WORK ,POWER)
Explore 24 Videos
- DIMENSIONSBook:ICSEChapter:DIMENSIONSExercise:SELECTED PROBLEMS (FROM CONVERSIONS OF ONE SYSTEMS OF UNITS INTO ANOTHER)
Explore 9 Videos
Similar Questions
A particle moves such that its acceleration is given by a=−β(x−2)
Here, β is a positive constnt and x the x-coordinate with respect to the origin. Time period of oscillation is
View SolutionA particle executes simple harmonic motion. Its instantaneous acceleration is given by a=−px, where p is a positive constant and x is the displacement from the mean position. Find angular frequency of oscillation.
View SolutionAcceleration of a particle in SHM at displacement x=10cm (from the mean position is a=−2.5cm/s2). Find time period of oscillations.
View SolutionA small ring of mass m1 is connected by a string of length l to a small heavy bob of mass m2. The ring os free to move (slide) along a fixed smooth horizontal wire. The bob is given a small displacement from its equilibrium position at right angles to string. Find period of small oscillations.
_E01_106_Q01.png)
View SolutionA mass of 1.5 kg is connected to two identical springs each of force constant 300Nm−1 as shown in the figure. If the mass is displaced from its equilibrium position by 10cm, then the period of oscillation is

View SolutionA 5 kg collar is attached to a spring of spring constant 500Nm−1 . It slides without friction over a horizontal rod. The collar is displaced from its equilibrium position by 10 cm and released. Calculate (a) The period of oscillations (b) The maximum speed and (c) maximum acceleration of the collar.
View SolutionA block of mass m hangs from a vertical spring of spring constant k. If it is displaced from its equilibrium position, find the time period of oscillations.
View SolutionA particle starts from point A, moves along a straight line path with an accleration given by a=2(4−x) where x is distance from point A. The particle stops at point B for a moment. Find the distance AB (in m). (All values are in S.I. units)
View SolutionFigure shows a system consisting of a massless pulley, a spring of force constant k and a block of mass m. If the block is slightly displaced vertically down from is equilibrium position and then released, the period of its vertical oscillation is

View SolutionA system shown in the figure consists of a massless pulley, a spring of force constant k displaced vertically downwards from its equilibrium position and released, then the Period of vertical oscillations is
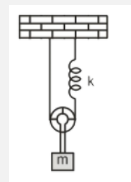
View Solution