The kinetic energy of a particle, executing S.H.M. is 16 J when it is in its mean position. IF the amplitude of oscillation is 25 cm and the mass of the particle is 5.12 Kg, the time period of its oscillations is
π/5 sec
2πsec
20πsec
5π sec
The correct Answer is:A
To solve the problem step by step, we will use the formulas related to kinetic energy in simple harmonic motion (SHM) and the relationship between angular frequency and time period.
Step 1: Understand the given data
- Kinetic Energy (KE) at mean position = 16 J
- Amplitude (A) = 25 cm = 0.25 m (convert to meters)
- Mass (m) = 5.12 kg
Step 2: Write down the formula for kinetic energy in SHM
The kinetic energy (KE) of a particle in SHM at the mean position is given by the formula:
KE=12mω2A2
where:
- ω is the angular frequency in radians per second,
- A is the amplitude in meters.
Step 3: Substitute the known values into the kinetic energy formula
Substituting the known values into the formula:
16=12×5.12×ω2×(0.25)2
Step 4: Simplify the equation
Calculate (0.25)2:
(0.25)2=0.0625
Now substitute this value into the equation:
16=12×5.12×ω2×0.0625
Step 5: Rearrange the equation to solve for ω2
Multiply both sides by 2 to eliminate the fraction:
32=5.12×ω2×0.0625
Now divide both sides by (5.12×0.0625):
ω2=325.12×0.0625
Step 6: Calculate ω2
Calculate 5.12×0.0625:
5.12×0.0625=0.32
Now substitute this back into the equation:
ω2=320.32=100
Step 7: Find ω
Take the square root of both sides to find ω:
ω=√100=10rad/s
Step 8: Calculate the time period T
The time period T is related to angular frequency ω by the formula:
T=2πω
Substituting ω=10:
T=2π10=π5seconds
Final Answer
The time period of the oscillations is:
T=π5seconds
---
- Kinetic Energy (KE) at mean position = 16 J
- Amplitude (A) = 25 cm = 0.25 m (convert to meters)
- Mass (m) = 5.12 kg
The kinetic energy (KE) of a particle in SHM at the mean position is given by the formula:
KE=12mω2A2
where:
- ω is the angular frequency in radians per second,
- A is the amplitude in meters.
Substituting the known values into the formula:
16=12×5.12×ω2×(0.25)2
Calculate (0.25)2:
(0.25)2=0.0625
Now substitute this value into the equation:
16=12×5.12×ω2×0.0625
Multiply both sides by 2 to eliminate the fraction:
32=5.12×ω2×0.0625
Now divide both sides by (5.12×0.0625):
ω2=325.12×0.0625
Calculate 5.12×0.0625:
5.12×0.0625=0.32
Now substitute this back into the equation:
ω2=320.32=100
Take the square root of both sides to find ω:
ω=√100=10rad/s
The time period T is related to angular frequency ω by the formula:
T=2πω
Substituting ω=10:
T=2π10=π5seconds
The time period of the oscillations is:
T=π5seconds
Topper's Solved these Questions
- COMPETITION CARE UNITBook:ICSEChapter:COMPETITION CARE UNITExercise:WAVES
Explore 98 Videos
- COMPETITION CARE UNITBook:ICSEChapter:COMPETITION CARE UNITExercise:NDA EXAM QUESTIONS
Explore 55 Videos
- COMPETITION CARE UNITBook:ICSEChapter:COMPETITION CARE UNITExercise:THERMAL RADIATION
Explore 13 Videos
- CIRCULAR MOTION Book:ICSEChapter:CIRCULAR MOTION Exercise:MODULE 2 (FROM ROTATIONAL KINETIC ENERGY , WORK ,POWER)
Explore 24 Videos
- DIMENSIONSBook:ICSEChapter:DIMENSIONSExercise:SELECTED PROBLEMS (FROM CONVERSIONS OF ONE SYSTEMS OF UNITS INTO ANOTHER)
Explore 9 Videos
Similar Questions
The kinetic energy of a particle executing shm is 32 J when it passes through the mean position. If the mass of the particle is.4 kg and the amplitude is one metre, find its time period.
View SolutionThe maximum velocity of a particle, executing SHM with an amplitude 7 mm is 4.4 m/s. the period of oscillation is
View SolutionThe potential energy of a particle executing S.H.M. is 2.5 J, when its displacement is half of amplitude. The total energy of the particle will be
View SolutionTime period of a particle executing SHM is 16s.At time t=2s, it crosses the mean position . Its amplitude of motion is 32√2πm. Its velocity at t=4s is
View SolutionThe potential energy of a particle execuring S.H.M. is 5 J, when its displacement is half of amplitude. The total energy of the particle be
View SolutionThe potential energy of a particle executing S H M is 25 J. when its displacement is half of amplitude. The total energy of the particle is
View SolutionThe time period of a particle executing S.H.M.is 12 s. The shortest distance travelledby it from mean position in 2 second is ( amplitude is a )
View SolutionPotential energy of a particle at mean position is 4 J and at extreme position is 20 J. Given that amplitude of oscillation is A. Match the following two columns
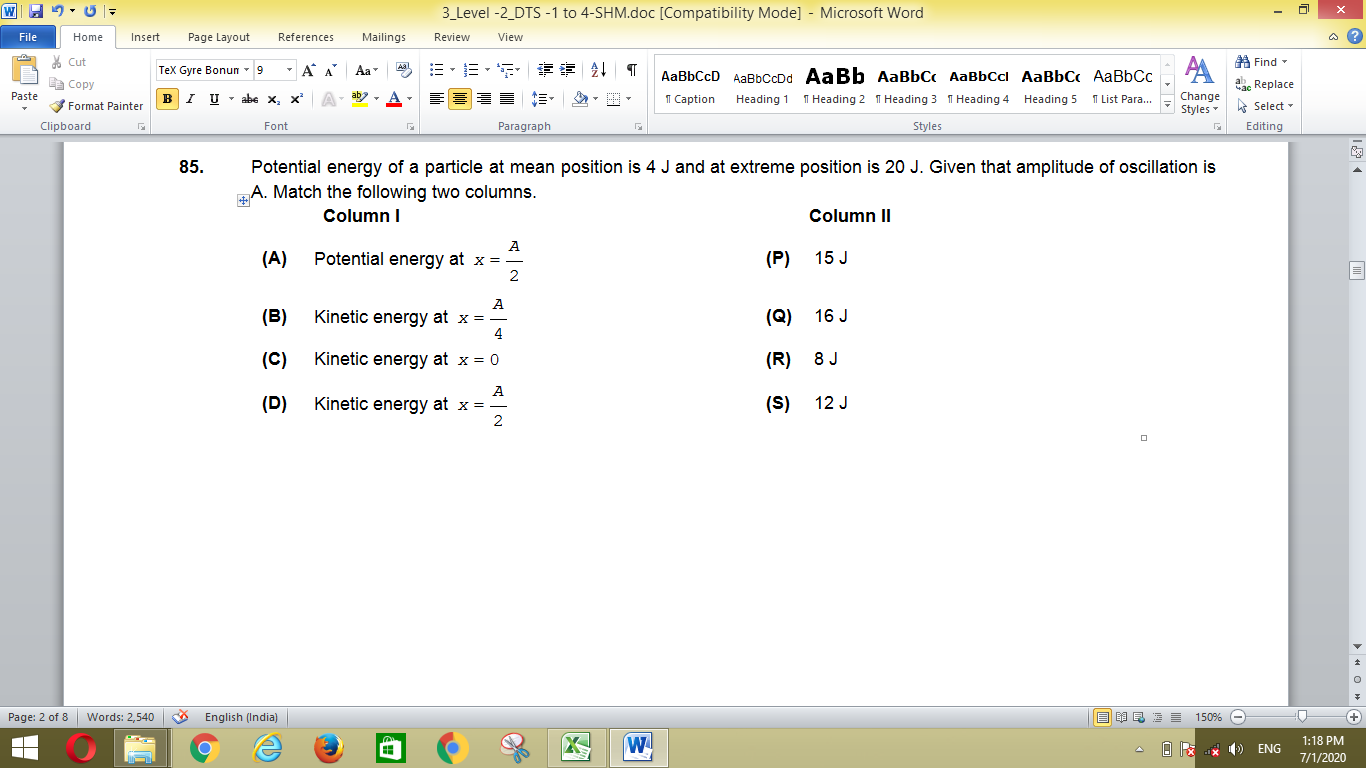
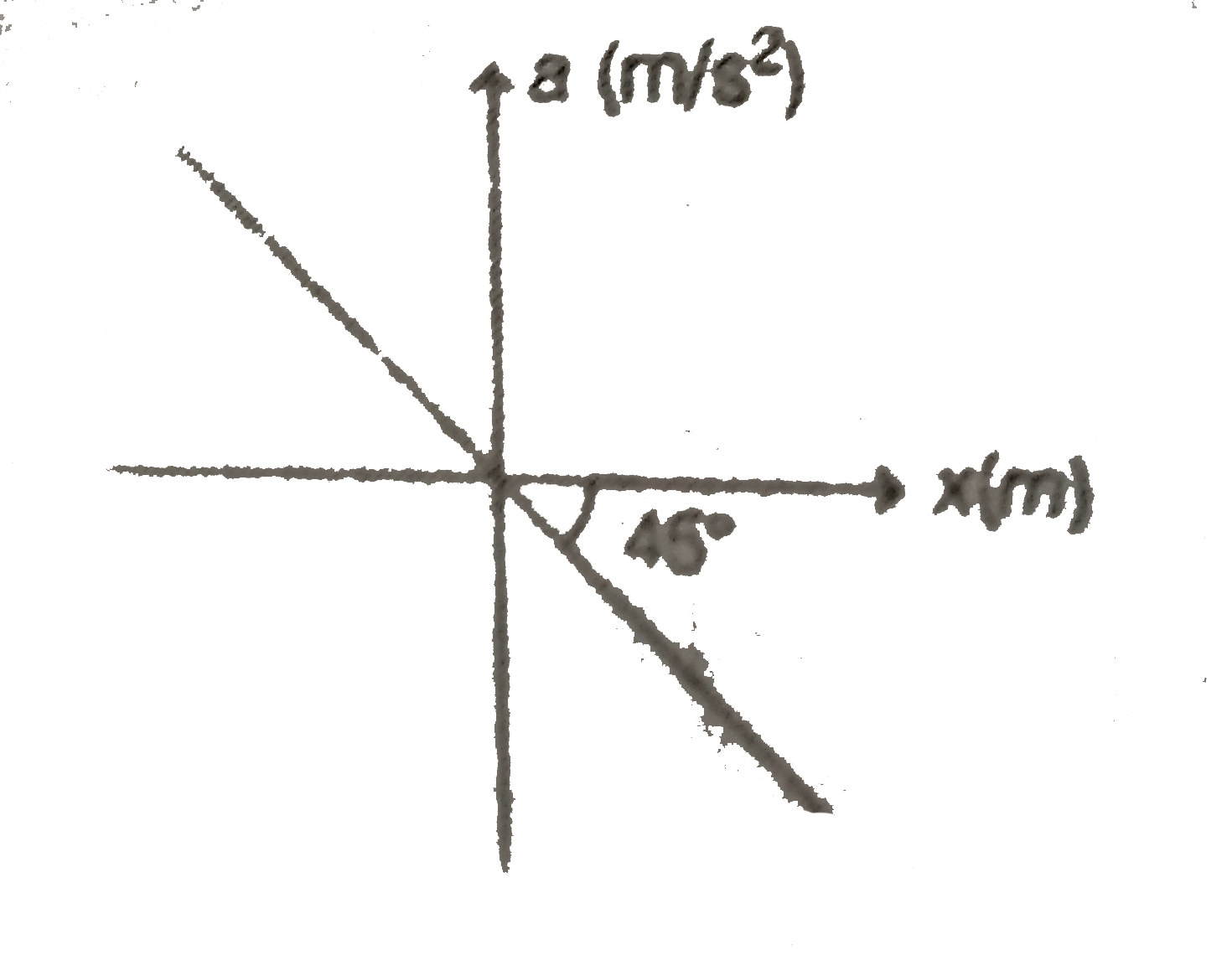
View SolutionAcceleration -displacemnet graph of a particle executing SHM is as shown in given figure. The time period of its oscillations is (in s)
View SolutionThe total energy of a particle in SHM is E. Its kinetic energy at half the amplitude from mean position will be
View Solution