One end of a uniform wire of length L and of weight W is attached rigidly to a point in the roof and a weight W1 is suspended from its lower end. IF S is the area of cross-section of the wire, the stress in the wire at a height 3L/4 from its lower end is
W1/S
(W1+W4)S
W1+3W4S
(W1+W)S
The correct Answer is:C
To solve the problem, we need to determine the stress in the wire at a height of 3L4 from its lower end. Here's the step-by-step solution:
Step 1: Understand the setup
- We have a uniform wire of length L and weight W attached to a roof at one end.
- A weight W1 is suspended from the lower end of the wire.
- We need to find the stress in the wire at a height of 3L4 from the lower end.
Step 2: Identify the forces acting on the wire
- The total weight acting on the wire at the point 3L4 from the lower end includes:
- The weight of the suspended mass W1.
- The weight of the portion of the wire below the height 3L4.
Step 3: Calculate the weight of the wire below the height 3L4
- The length of the wire below the height 3L4 is L4.
- The weight of this portion of the wire can be calculated as:
Weight of wire below 3L4=WL⋅L4=W4
- This is because the weight of the wire is uniformly distributed along its length.
Step 4: Calculate the total downward force at height 3L4
- The total force acting downwards at the height 3L4 is the sum of the weight of the suspended mass and the weight of the wire below that point:
Ftotal=W1+W4
Step 5: Calculate the stress in the wire
- Stress (σ) is defined as the force per unit area. Therefore, the stress at the height 3L4 is given by:
σ=FtotalS=W1+W4S
Final Answer
Thus, the stress in the wire at a height of 3L4 from its lower end is:
σ=W1+W4S
---
- We have a uniform wire of length L and weight W attached to a roof at one end.
- A weight W1 is suspended from the lower end of the wire.
- We need to find the stress in the wire at a height of 3L4 from the lower end.
- The total weight acting on the wire at the point 3L4 from the lower end includes:
- The weight of the suspended mass W1.
- The weight of the portion of the wire below the height 3L4.
- The length of the wire below the height 3L4 is L4.
- The weight of this portion of the wire can be calculated as:
Weight of wire below 3L4=WL⋅L4=W4
- This is because the weight of the wire is uniformly distributed along its length.
- The total force acting downwards at the height 3L4 is the sum of the weight of the suspended mass and the weight of the wire below that point:
Ftotal=W1+W4
- Stress (σ) is defined as the force per unit area. Therefore, the stress at the height 3L4 is given by:
σ=FtotalS=W1+W4S
Thus, the stress in the wire at a height of 3L4 from its lower end is:
σ=W1+W4S
Topper's Solved these Questions
- COMPETITION CARE UNITBOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - PROPERTIES OF MATTER (SURFACE TENSION )
23 Videos
- COMPETITION CARE UNITBOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - PROPERTIES OF MATTER (CALORIMETRY, CHANGE OF STATE & KINETIC THEORY OF GASES )
30 Videos
- COMPETITION CARE UNITBOOK - ICSECHAPTER - COMPETITION CARE UNITEXERCISE - GRAVITATION
25 Videos
- CIRCULAR MOTION BOOK - ICSECHAPTER - CIRCULAR MOTION EXERCISE - MODULE 2 (FROM ROTATIONAL KINETIC ENERGY , WORK ,POWER)
24 Videos
- DIMENSIONSBOOK - ICSECHAPTER - DIMENSIONSEXERCISE - SELECTED PROBLEMS (FROM CONVERSIONS OF ONE SYSTEMS OF UNITS INTO ANOTHER)
9 Videos
Similar Questions
One end of uniform wire of length L and of weight W is attached rigidly to a point in the roof and a weight W1 is suspended from its lower end. If s is the area of cross section of the wire, the stress in the wire at a height (3L/4) from its lower end is
View SolutionOne end of a wire of length L and weight w is attached rigidly to a point in roof and a weight w1 is suspended from its lower end. If A is the area of cross-section of the wire then the stress in the wire at a height 3L4 from its lower end is
View Solution
Knowledge Check
A metal wire of length L1 and area of cross section A is attached to a rigid support. Another metal wire of length L2 and of the same cross sectional area is attached to the free end of the first wire. A body of mass M is then suspended from the free end of the second wire, if Y1 and Y2 are the Young's moduli of the wires respectively the effective force constant of the system of two wires is
A[(Y1Y2)A][2(Y1L2+Y2L1)]B[(Y1Y2)A](L1L2)2C[(Y1Y2)A](Y1L2+Y2L1)D[(Y1Y2)1/2A](L2+L1)1/2
A block of mass M is suspended from a wire of length L, area of cross-section A and Young's modulus Y. The elastic potential energy stored in the wire is
View SolutionOne end of a Nichrome wire of length 2L and cross-sectional area A is attached to an end of another Nichrome wire of length L and cross-sectional area 2A. If the free end of the longer wire is at an electric potential of 8.0 volts, and the free end of the shorter wire is at an electric potential 1.0 volts, the potential at the junction of the two wires is equal to
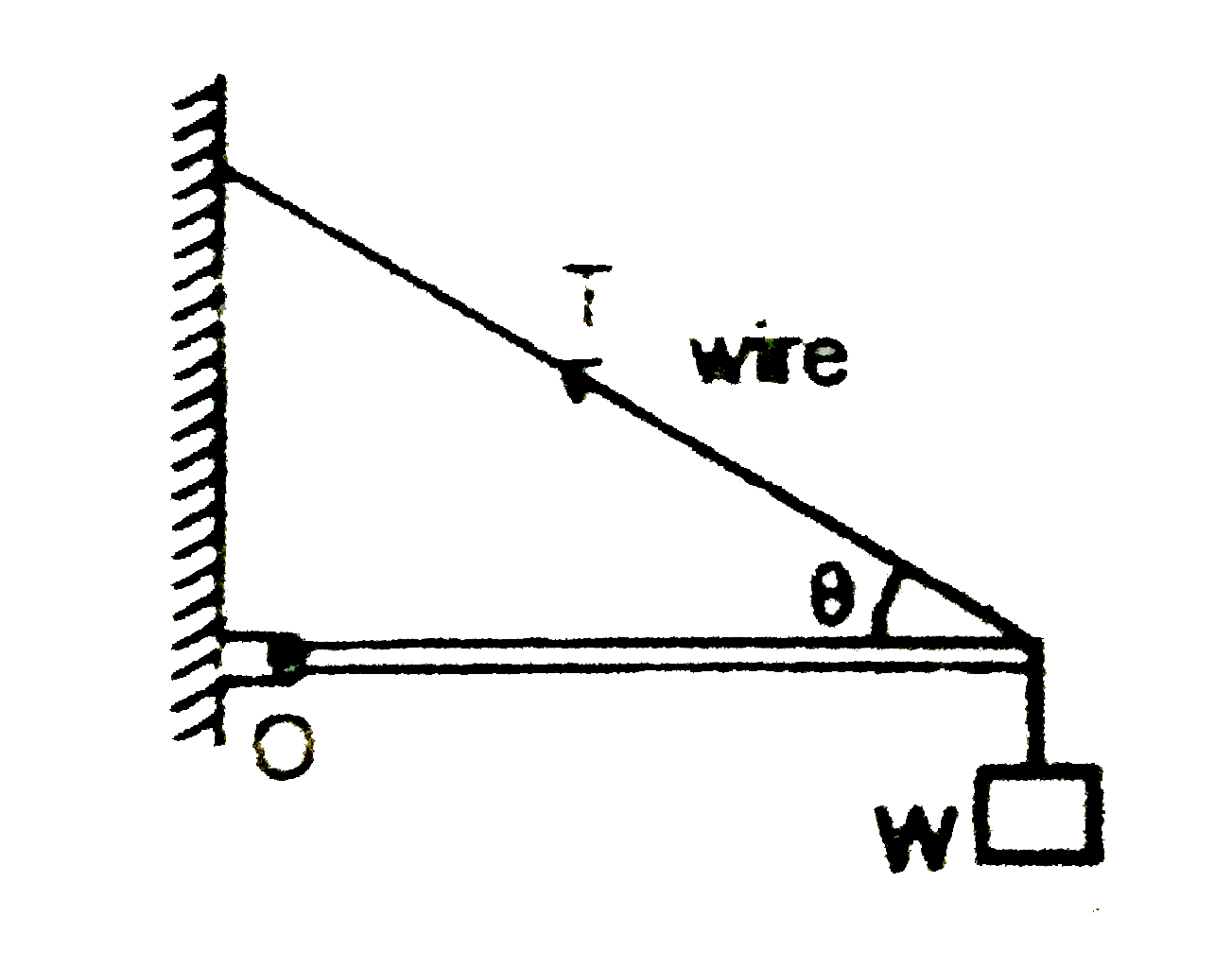
View SolutionA uniform rod of length L and weight WR is suspended as shown in the accompanying figure. A weight W is added to the end of the rod. The rod rests in horizontal position and is hinged at O. the support wire is at an angle θ to the rod. What is the tension T in the wire ?
View SolutionA bar of mass m and length l is hanging from point A as shown in the figure . If the Young's modulus of elasticity of the bar is Y and area of cross - section of the wire is A, then the increase in its length due to its own weight will be

View SolutionA wire of length L,area of cross section A is hanging from a fixed support. The length of the wire changes to L1 when mass M is suspended from its free end. The expression for Young's modulus is:
View SolutionA wire of resistance 4Ω is stretched to twice its original length. In the process of stretching, its area of cross-section gets halved. Now, the resistance of the wire is
View SolutionA weight is suspended from a long metal wire. If the wire suddenly breaks, its temperature
View Solution
