One end of uniform wire of length and of weight is attached rigidly to a point in the roof and a weight is suspended from its lower end. If is the area of cross section of the wire, the stress in the wire at a height () from its lower end is
The correct Answer is:C
To find the stress in the wire at a height of 3L4 from its lower end, we can follow these steps:
Step 1: Understand the Setup
We have a uniform wire of length L and weight W attached at one end to the roof. A weight W1 is suspended from the lower end of the wire. We need to find the stress at a point that is 3L4 from the lower end.
Step 2: Identify the Forces Acting on the Wire
At the point 3L4 from the lower end, the forces acting on the wire include:
1. The weight W1 acting downward.
2. The weight of the portion of the wire below this point. Since the total weight of the wire is W and its length is L, the weight of the portion of the wire from 3L4 to L (which is L4) can be calculated as:
Weight of the lower part of the wire=14W
Step 3: Calculate the Total Weight Acting Downward
The total downward force (restoring force) at the height 3L4 is the sum of W1 and the weight of the lower part of the wire:
F=W1+14W
Step 4: Calculate the Stress
Stress (σ) is defined as the force per unit area. The area of cross-section of the wire is given as S. Therefore, the stress at the height 3L4 from the lower end can be calculated as:
σ=FS=W1+14WS
Final Expression
Thus, the stress in the wire at a height of 3L4 from the lower end is:
σ=W1+14WS
We have a uniform wire of length L and weight W attached at one end to the roof. A weight W1 is suspended from the lower end of the wire. We need to find the stress at a point that is 3L4 from the lower end.
At the point 3L4 from the lower end, the forces acting on the wire include:
1. The weight W1 acting downward.
2. The weight of the portion of the wire below this point. Since the total weight of the wire is W and its length is L, the weight of the portion of the wire from 3L4 to L (which is L4) can be calculated as:
Weight of the lower part of the wire=14W
The total downward force (restoring force) at the height 3L4 is the sum of W1 and the weight of the lower part of the wire:
F=W1+14W
Stress (σ) is defined as the force per unit area. The area of cross-section of the wire is given as S. Therefore, the stress at the height 3L4 from the lower end can be calculated as:
σ=FS=W1+14WS
Thus, the stress in the wire at a height of 3L4 from the lower end is:
σ=W1+14WS
Topper's Solved these Questions
- ELASTICITYBOOK - DC PANDEY ENGLISHCHAPTER - ELASTICITYEXERCISE - Match the columns
4 Videos
- ELASTICITYBOOK - DC PANDEY ENGLISHCHAPTER - ELASTICITYEXERCISE - Medical entrances s gallery
21 Videos
- ELASTICITYBOOK - DC PANDEY ENGLISHCHAPTER - ELASTICITYEXERCISE - Check point 12.3
15 Videos
- CURRENT ELECTRICITYBOOK - DC PANDEY ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - All Questions
469 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Integer
17 Videos
Similar Questions
One end of a wire of length L and weight w is attached rigidly to a point in roof and a weight w1 is suspended from its lower end. If A is the area of cross-section of the wire then the stress in the wire at a height 3L4 from its lower end is
View SolutionA block of mass M is suspended from a wire of length L, area of cross-section A and Young's modulus Y. The elastic potential energy stored in the wire is
View Solution
Knowledge Check
One end of a uniform wire of length L and of weight W is attached rigidly to a point in the roof and a weight W1 is suspended from its lower end. IF S is the area of cross-section of the wire, the stress in the wire at a height 3L/4 from its lower end is
AW1/SB(W1+W4)SCW1+3W4SD(W1+W)SA wire is suspended from the ceiling and stretched under the action of weight F suspended from its other end. The force exerted by the ceiling on it is equal and opposite to the weight.
ATensile stress at any cross section A of the wire is FA.BTensile stress at any cross section is zero.CTensile stress at any cross section A of the wire is 2FA.DTension at any cross section A of the wire is 2F.
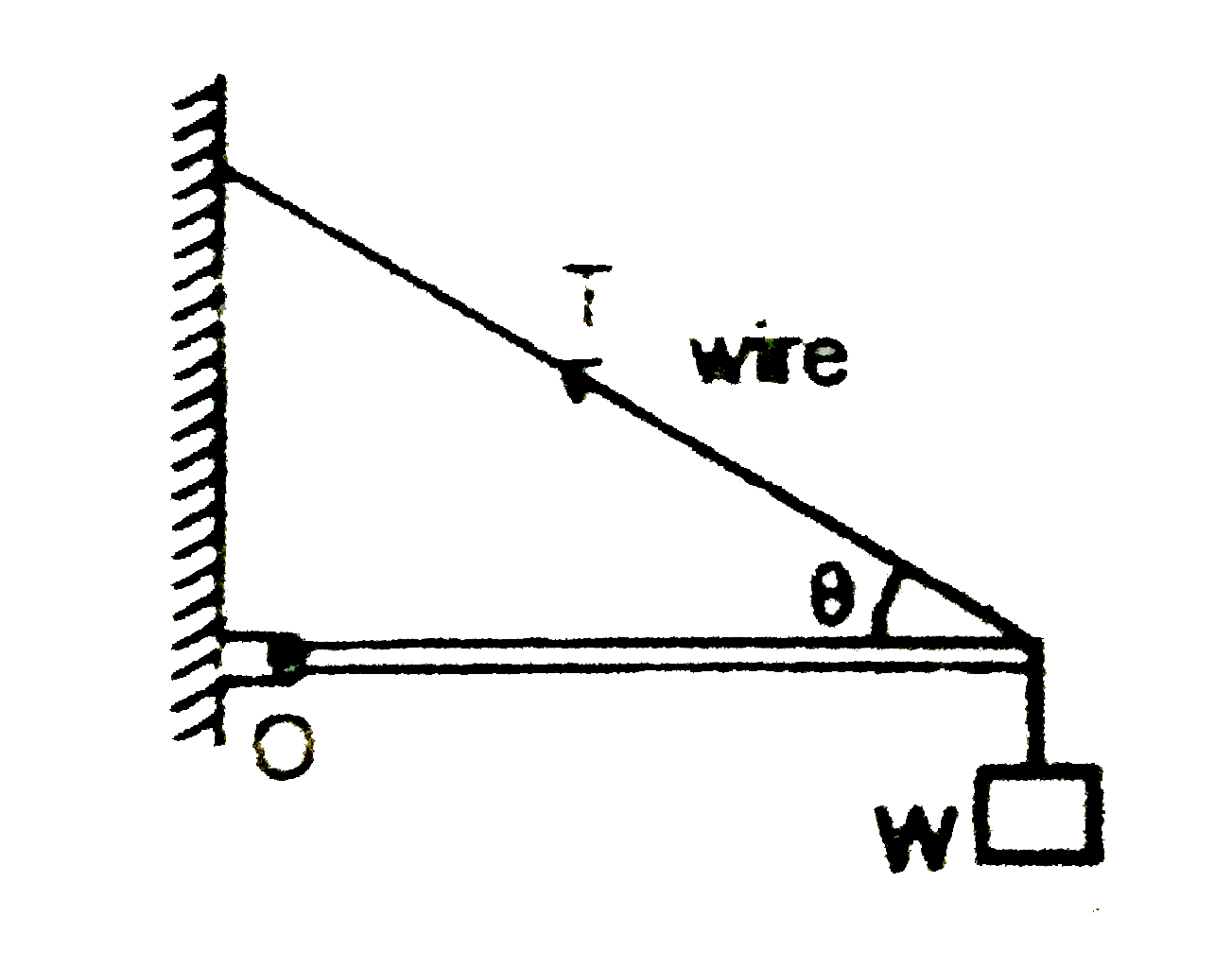
A uniform rod of length L and weight WR is suspended as shown in the accompanying figure. A weight W is added to the end of the rod. The rod rests in horizontal position and is hinged at O. the support wire is at an angle θ to the rod. What is the tension T in the wire ?
View SolutionA wire is suspended from the ceiling and stretched under the action of weight F suspended from its other end. The force exerted by the ceiling on it is equal and opposite to the weight.
View SolutionA steel wire 2m long is suspended from the ceiling. When a mass is hung from its lower end, the increase in length recorded is 1cm. Determine the strain in the wire.
View SolutionA bar of mass m and length l is hanging from point A as shown in the figure . If the Young's modulus of elasticity of the bar is Y and area of cross - section of the wire is A, then the increase in its length due to its own weight will be

View SolutionA weight is suspended from a long metal wire. If the wire suddenly breaks, its temperature
View SolutionA wire of length L,area of cross section A is hanging from a fixed support. The length of the wire changes to L1 when mass M is suspended from its free end. The expression for Young's modulus is:
View Solution
